A Real Estate Analyst Believes That the Three Main Factors

Multiple Choice

A real estate analyst believes that the three main factors that influence an apartment's rent in a college town are the number of bedrooms, the number of bathrooms, and the apartment's square footage. For 40 apartments, she collects data on the rent (y, in $) , the number of bedrooms (x₁) , the number of bathrooms (x₂) , and its square footage (x₃) . She estimates the following model: Rent = β₀ + β₁Bedroom + β₂Bath + β₃Sqft + ε. The following table shows a portion of the regression results. 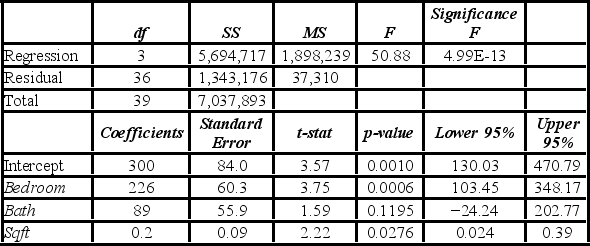When testing whether the explanatory variables Bath and Sqft are jointly significant, the p-value associated with the test is 0.0039. At the 5% significance level, she ________.

A) rejects H₀, and cannot conclude that at least one of the explanatory variables, Bath and/or Sqft, is significant in explaining Rent
B) does not reject H₀, and concludes that at least one of the explanatory variables, Bath and/or Sqft, is significant in explaining Rent
C) rejects H₀, and concludes that at least one of the explanatory variables, Bath and/or Sqft, is significant in explaining Rent
D) does not reject H₀, and cannot conclude that at least one of the explanatory variables, Bath and/or Sqft, is significant in explaining Rent