Multiple Choice
To examine the differences between salaries of male and female middle managers of a large bank, 90 individuals were randomly selected, and two models were created with the following variables considered: Salary = the monthly salary (excluding fringe benefits and bonuses) ,
Educ = the number of years of education,
Exper = the number of months of experience,
Train = the number of weeks of training,
Gender = the gender of an individual; 1 for males, and 0 for females.
Excel partial outputs corresponding to these models are available and shown below.
Model A: Salary = β0 + β1Educ + β2Exper + β3Train + β4Gender + ε 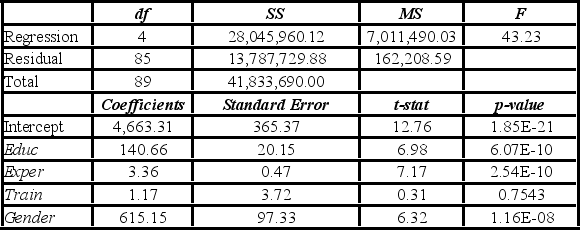 Model B: Salary = β0 + β1Educ + β2Exper + β3Gender + ε
Model B: Salary = β0 + β1Educ + β2Exper + β3Gender + ε 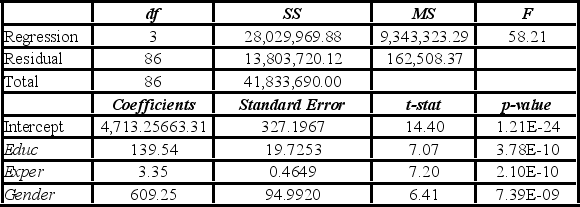 Which of the following is the regression equation found by Excel for Model A?
Which of the following is the regression equation found by Excel for Model A?
A) 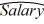 = 4663.31 + 140.66Educ + 3.36Exper + 1.17Train + 615.15Gender
= 4663.31 + 140.66Educ + 3.36Exper + 1.17Train + 615.15Gender
B) 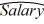 = 365.37 + 20.16Educ + 0.47Exper + 3.72Train + 97.33Gender
= 365.37 + 20.16Educ + 0.47Exper + 3.72Train + 97.33Gender
C) 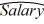 = 12.76 + 6.98Educ + 7.15Exper + 0.31Train + 6.32Gender
= 12.76 + 6.98Educ + 7.15Exper + 0.31Train + 6.32Gender
D) 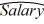 = 140.66Educ + 3.36Exper + 1.17Train + 615.15Gender
= 140.66Educ + 3.36Exper + 1.17Train + 615.15Gender
Correct Answer:

Verified
Correct Answer:
Verified
Q80: A medical researcher is interested in assessing
Q81: In the regression equation <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB6618/.jpg" alt="In
Q82: Consider the model y = β<sub>0 </sub>+
Q83: The major shortcoming of the general linear
Q84: To avoid the dummy variable _, the
Q86: A realtor wants to predict and compare
Q87: For the linear probability model y =
Q88: In the model y = β<sub>0</sub> +
Q89: To examine the differences between salaries of
Q90: A medical researcher is interested in assessing