Exam 12: Waiting Lines and Queuing Theory Models

A new shopping mall is considering setting up an information desk manned by one employee.Based upon information obtained from similar information desks,it is believed that people will arrive at the desk at the rate of 15 per hour.It takes exactly two minutes to answer each question.It is assumed that arrivals are Poisson. (a)Find the probability that the employee is idle. (b)Find the proportion of time that the employee is busy. (c)Find the average number of people receiving and waiting to receive information. (d)Find the average number of people waiting in line to get information. (e)Find the average time a person seeking information spends at the desk. (f)Find the expected time a person spends waiting in line to have his question answered.

Table 12-4 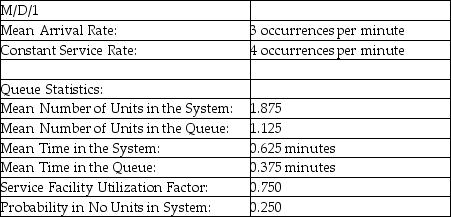-According to the information provided in Table 12-4,which presents a queuing problem solution for a queuing problem with a constant service rate,on average,how many customers arrive per time period?

Service times often follow a Poisson distribution.

The school of business has 3 fax machines.The toner in each machine needs to be changed after about 5 hours of use.There is one unit secretary who is responsible for the fax machine maintenance.It takes him 15 minutes to replace the toner cartridge.What is the average number of fax machines in the system?

A new shopping mall is considering setting up an information desk operated by two employees.Based on information obtained from similar information desks,it is believed that people will arrive at the desk at the rate of 20 per hour.It takes an average of 4 minutes to answer a question.It is assumed that arrivals are Poisson and answer times are exponentially distributed. (a)Find the proportion of the time that the employees are busy. (b)Find the average number of people waiting in line to get some information. (c)Find the expected time a person spends just waiting in line to have his question answered.

A finite population model differs from an infinite population model because there is a random relationship between the length of the queue and the arrival rate.

A queue system is in a transient state before the steady state is reached.

The initial state of a queuing system (for example,when a restaurant first opens),is referred to as the ________ state.

A vendor selling newspapers on a street corner is an example of a

Table 12-6 Customers arrive at coffee shop at a rate of 40 per hour.There are 2 servers available and it takes an average of 1 minute to serve each customer. -Using Table 12-6,what is the average number of customers waiting in line?

Which of the following distributions is most often used to estimate service times?

A hospital ward with only 30 beds could be modeled using a finite population model.

Balking customers are those who enter the queue but then become impatient and leave without completing the transaction.

What is the steady state of a queuing system?

Little's Flow Equations are advantageous because if one characteristic of the operating system is known,the other characteristics can be easily found.

The percent idle time is equal to 1 minus the utilization factor.

In queuing analysis,total expected cost is the sum of expected ________ plus expected ________.

Sam the Vet is running a rabies vaccination clinic for dogs at the local grade school.Sam can vaccinate a dog every 3 minutes.It is estimated that the dogs will arrive independently and randomly throughout the day at a rate of 1 dog every 6 minutes,according to a Poisson distribution.Also assume that Sam's vaccinating times are exponentially distributed. (a)Find the probability that Sam is idle. (b)Find the proportion of time that Sam is busy. (c)Find the average number of dogs receiving or waiting to be vaccinated. (d)Find the average number of dogs waiting to be vaccinated. (e)Find the average time a dog waits before getting vaccinated. (f)Find the average amount (mean)of time a dog spends between waiting in line and getting vaccinated.

The study of waiting lines is called queuing theory.

The school of business has 3 fax machines.The toner in each machine needs to be changed after about 5 hours of use.There is one unit secretary who is responsible for the fax machine maintenance.It takes him 15 minutes to replace the toner cartridge.What is the average waiting time in the queue?