Exam 6: Differential Equations
Exam 1: Preparation for Calculus96 Questions
Exam 2: Limits and Their Properties119 Questions
Exam 3: Differentiation208 Questions
Exam 4: Applications of Differentiation147 Questions
Exam 5: Integration165 Questions
Exam 6: Differential Equations88 Questions
Exam 7: Applications of Integration90 Questions
Exam 8: Integration Techniques, L'Hôpital's Rule, and Improper Integrals142 Questions
Exam 9: Infinite Series199 Questions
Exam 10: Parametric Equations, Polar Coordinates, and Vectors173 Questions
Exam 11: Mechanical Ventilation and Sedation: Assessment, Medications, and Complications225 Questions
Select questions type
Sketch a few solutions of the differential equation on the slope field and then find the general solution analytically. 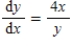

(Multiple Choice)
4.9/5  (33)
(33)
The isotope  has a half-life of 24,100 years.Given an initial amount of 6 grams of the isotope,how many grams will remain after 500 years? After 5,000 years? Round your answers to four decimal places.
has a half-life of 24,100 years.Given an initial amount of 6 grams of the isotope,how many grams will remain after 500 years? After 5,000 years? Round your answers to four decimal places.
(Multiple Choice)
4.9/5  (38)
(38)
The number of bacteria in a culture is increasing according to the law of exponential growth.After 5 hours there are 130 bacteria in the culture and after 10 hours there are 330 bacteria in the culture.Answer the following questions,rounding numerical answers to four decimal places.
(I)Find the initial population.
(II)Write an exponential growth model for the bacteria population.Let t represent time in hours.
(III)Use the model to determine the number of bacteria after 20 hours.
(IV)After how many hours will the bacteria count be 10,000?
(Multiple Choice)
4.7/5  (36)
(36)
Consider the differential equation 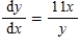 ,for
,for 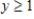 only,with initial value
only,with initial value 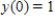 .
The particular solution y(x)is
.
The particular solution y(x)is
(Multiple Choice)
4.9/5  (35)
(35)
Find the logistic equation that satisfies the following differential equation and initial condition. 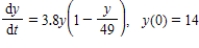
(Multiple Choice)
4.8/5  (39)
(39)
A population of rabbits in a certain habitat grows according to the differential equation  where t is measured in months
where t is measured in months 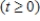 and y is measured in hundreds of rabbits.There were initially 100 rabbits in this habitat;that is,
and y is measured in hundreds of rabbits.There were initially 100 rabbits in this habitat;that is, 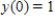 .
Which of the following slope fields represents an approximate general solution to the given differential equation?
.
Which of the following slope fields represents an approximate general solution to the given differential equation?
(Multiple Choice)
4.8/5  (33)
(33)
A conservation organization releases 40 coyotes into a preserve.After 4 years,there are 70 coyotes in the preserve.The preserve has a carrying capacity of 175.Determine the time it takes for the population to reach 140.
(Multiple Choice)
4.8/5  (28)
(28)
The logistic function 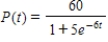 models the growth of a population.Identify the initial population.
models the growth of a population.Identify the initial population.
(Multiple Choice)
4.9/5  (34)
(34)
Showing 81 - 88 of 88
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)