Exam 9: Exponential and Logarithmic Functions
Exam 1: Real Numbers and Algebraic Expressions409 Questions
Exam 2: Equations, Inequalities, and Problem Solving282 Questions
Exam 3: Graphs and Functions388 Questions
Exam 4: Systems of Equations and Inequalities131 Questions
Exam 5: Polynomials and Polynomial Functions390 Questions
Exam 6: Rational Expressions292 Questions
Exam 7: Rational Exponents, Radicals, and Complex Numbers382 Questions
Exam 8: Quadratic Equations and Functions251 Questions
Exam 9: Exponential and Logarithmic Functions300 Questions
Exam 10: Conic Sections132 Questions
Exam 11: Fractions134 Questions
Select questions type
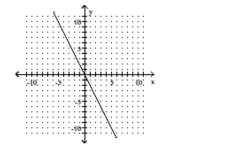
Graph the inverse of the function on the same set of axes.
-
Free
(Multiple Choice)
4.7/5  (43)
(43)
Correct Answer:
D
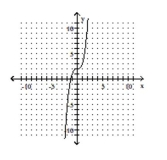
Graph the inverse of the function on the same set of axes.
-
Free
(Multiple Choice)
5.0/5  (39)
(39)
Correct Answer:
D
Graph the function and its inverse on the same set of axes.
-
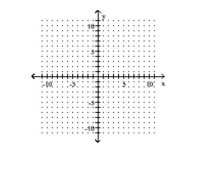
(Multiple Choice)
4.9/5  (40)
(40)
Express as the logarithm of a single expression. Assume that variables represent positive numbers.
-
(Multiple Choice)
4.8/5  (37)
(37)
Solve the equation. Give an approximate solution to four decimal places.
-
(Multiple Choice)
4.8/5  (32)
(32)
For the given functions f and g, find the composition.
-
Find .
(Multiple Choice)
4.9/5  (29)
(29)
Use a calculator to approximate the logarithm to four decimal places.
-
(Multiple Choice)
4.9/5  (30)
(30)
Write the expression as sums or differences of multiples of logarithms.
-
(Multiple Choice)
4.8/5  (31)
(31)
Determine whether the functions f and g are inverses of each other.
-
(Multiple Choice)
4.9/5  (33)
(33)
If the function is one-to-one, list the inverse function by switching coordinates or inputs and outputs.
-
(Multiple Choice)
4.8/5  (40)
(40)
For the given functions f and g, find the composition.
-
Find .
(Multiple Choice)
4.7/5  (39)
(39)
Express as the logarithm of a single expression. Assume that variables represent positive numbers.
-
(Multiple Choice)
4.8/5  (38)
(38)
Express as the logarithm of a single expression. Assume that variables represent positive numbers.
-
(Multiple Choice)
4.8/5  (40)
(40)
Solve the equation for x. Give an approximate solution accurate to four decimal places.
-
(Multiple Choice)
4.8/5  (38)
(38)
Showing 1 - 20 of 300
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)