Exam 7: Analytic Geometry

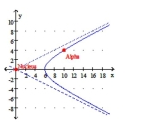
Solve the problem. -In 1911, Ernest Rutherford discovered the nucleus of the atom. Experiments leading to this discovery involved the scattering of alpha particles by the heavy nuclei in gold foil. When alpha particles are thrust towards the Gold nuclei, the particles are deflected and follow a hyperbolic path. Suppose the minimum distance that the alpha particles get to the gold nucleus is 6 microns, and that hyperbolic path has asymptotes of $y = \pm \frac { 1 } { 2 } x$ . Determine an equation of the path of the particle shown.

Write the word or phrase that best completes each statement or answers the question. Provide the missing information. -The line segment between the vertices of a hyperbola is called the ________axis.

The standard form of an equation of an ellipse centered at (h, k) with a horizontal major axis is ________, where $a > b > 0$ > 0. If the major axis is vertical, then the equation is________ .

Solve the problem. -A parabolic mirror on a telescope 30 cm in diameter has a focal length of 15 cm. For the coordinate system shown, write an equation of the parabolic cross section of the mirror.

Write the standard form of an equation of the ellipse subject to the given conditions. -Foci: $( - 13,4 )$ and $( 19,4 ) ;$ Eccentricity: $\frac { 4 } { 5 }$

Write the word or phrase that best completes each statement or answers the question. Provide the missing information. -The equation $\frac { x ^ { 2 } } { a ^ { 2 } } - \frac { y ^ { 2 } } { b ^ { 2 } } = 1$ = 1 represents a hyperbola with a (horizontal / vertical) transverse axis. The vertices are given by the ordered pairs ________and________ . The asymptotes are given by the equations________ and________ .

Graph the hyperbola. Identify the foci and write the equations for the asymptotes. - $\frac { y ^ { 2 } } { 144 } - \frac { x ^ { 2 } } { 25 } = 1$

Write the equation of the hyperbola in standard form. Identify the center and foci. - $6 x ^ { 2 } - 5 y ^ { 2 } + 60 x - 20 y + 100 = 0$

Solve the problem. -Atomic particles with like charges tend to repel one another. Suppose that two beams of like-charged particles are hurled towards each other from two parallel atomic accelerators. The path Defined by the particles is $x ^ { 2 } - 4 y ^ { 2 } = 36$ , where x and y are measured in microns. What is the Minimum distance between the particles?

Write the standard form of the equation of the hyperbola subject to the given conditions. -Vertices: $( - 2,5 ) , ( 10,5 ) ;$ eccentricity $\frac { 5 } { 3 }$

Determine the eccentricity of the hyperbola. - $\frac { \left( y + \frac { 7 } { 9 } \right) ^ { 2 } } { 196 } - \frac { \left( x - \frac { 5 } { 9 } \right) ^ { 2 } } { 2,304 } = 1$

Identify the vertices and the foci. - $- 10 x ^ { 2 } + 8 y ^ { 2 } = - 80$

Write the standard form of the equation of the hyperbola subject to the given conditions. -Vertices: $( 3,0 ) , ( - 3,0 )$ ; Foci: $( 5,0 ) , ( - 5,0 )$

An equation of a parabola is given. Write the equation of the parabola in standard form, and identify the vertex and the focus. - $x ^ { 2 } - 8 x - 16 y - 16 = 0$

Write the standard form of an equation of the ellipse subject to the given conditions. -Vertices: $( 0,10 )$ and $( 0 , - 10 )$ Passes through $\left( - \frac { 32 } { 5 } , 6 \right)$

Determine the standard form of an equation of the parabola subject to the given conditions. -Focus: $( - 4 , - 3 )$ ; Vertex: $( - 4 , - 5 )$

Write the word or phrase that best completes each statement or answers the question. Provide the missing information. -The equation $\frac { y ^ { 2 } } { a ^ { 2 } } - \frac { x ^ { 2 } } { b ^ { 2 } } = 1$ = 1 represents a hyperbola with a (horizontal / vertical) transverse axis. The vertices are given by the ordered pairs________ and________ . The asymptotes are given by the equations ________and ________.

Write the word or phrase that best completes each statement or answers the question. Provide the missing information. -The length of the latus rectum is called the________ diameter.

Solve the system of equations. - +4=103 4-=4

Graph the parabola. Identify the focus. - $2 x ^ { 2 } = 6 y$