Chat with AIExam 4: Applications of Differentiation
Exam 1: Preparation for Calculus125 Questions
Exam 2: Limits and Their Properties85 Questions
Exam 3: Differentiation193 Questions
Exam 4: Applications of Differentiation154 Questions
Exam 5: Integration184 Questions
Exam 6: Differential Equations93 Questions
Exam 7: Applications of Integration119 Questions
Exam 8: Integration Techniques and Improper Integrals130 Questions
Exam 9: Infinite Series181 Questions
Exam 10: Conics, Parametric Equations, and Polar Coordinates114 Questions
Exam 11: Vectors and the Geometry of Space130 Questions
Exam 12: Vector-Valued Functions85 Questions
Exam 13: Functions of Several Variables173 Questions
Exam 14: Multiple Integration143 Questions
Exam 15: Vector Anal142 Questions
Select questions type
The resistance R of a certain type of resistor is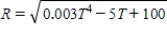 
where R is measured in ohms and the temperature T is measured in degrees Celsius. Use a computer algebra system to find 
.
where R is measured in ohms and the temperature T is measured in degrees Celsius. Use a computer algebra system to find . (Multiple Choice)
4.8/5 
(30)
(30) Locate the absolute extrema of the function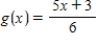 
on the closed interval 
.
on the closed interval 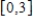. (Multiple Choice)
4.9/5 (43)
(43) The resistance R of a certain type of resistor is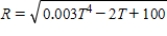 
where R is measured in ohms and the temperature T is measured in degrees Celsius. Use a computer algebra system to find the critical number of the function. Round numerical values in your answer to the nearest whole number.
where R is measured in ohms and the temperature T is measured in degrees Celsius. Use a computer algebra system to find the critical number of the function. Round numerical values in your answer to the nearest whole number. (Multiple Choice)
4.8/5 (36)
(36) Find the value of the derivative (if it exists) of 
at the indicated extremum. 
at the indicated extremum. 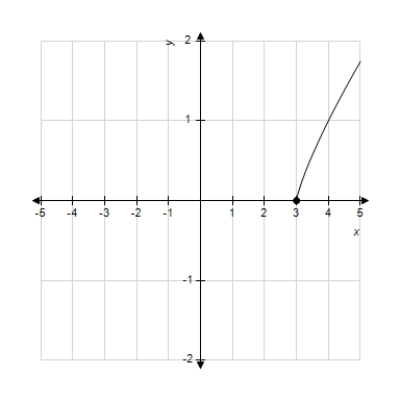(Multiple Choice)
4.8/5 (43)
(43) Assume that the amount of money deposited in a bank is proportional to the square of the interest rate the bank pays on this money. Furthermore, the bank can reinvest this money at 27%. Find the interest rate the bank should pay to maximize profit. (Use the simple interest formula.)
(Multiple Choice)
4.9/5 (45)
(45) Find all points of inflection of the graph of the function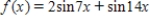 
on the interval 
. Round your answer to three decimal places wherever applicable.
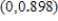on the interval . Round your answer to three decimal places wherever applicable. (Multiple Choice)
4.8/5 (25)
(25) 
with one of the following graphs. 
. Find a function f that has derivative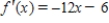 
and with graph passing through the point 
.
and with graph passing through the point 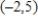. (Multiple Choice)
5.0/5 (38)
(38) Determine whether the Mean Value Theorem can be applied to the function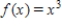 
on the closed interval 
. If the Mean Value Theorem can be applied, find all numbers c in the open interval 
such that 
.
on the closed interval 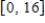. If the Mean Value Theorem can be applied, find all numbers c in the open interval 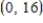such that 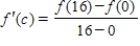. (Multiple Choice)
4.8/5 (47)
(47) The graph of a function f is shown below.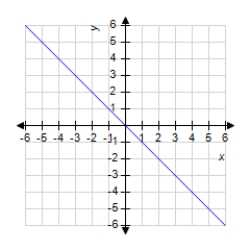 
Sketch the graph of the derivative 
.
Sketch the graph of the derivative .
(Multiple Choice)
4.7/5 (22)
(22) Find all relative extrema of the function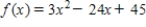 
. Use the Second Derivative Test where applicable.
. Use the Second Derivative Test where applicable. (Multiple Choice)
4.8/5 (33)
(33) Determine the x-coordinate(s) of any relative extrema and inflection points of the function 
.
. (Multiple Choice)
4.7/5 (34)
(34) A company introduces a new product for which the number of units sold S is 
where t is the time in months since the product was introduced. Find the average value of 
during the first year.
where t is the time in months since the product was introduced. Find the average value of 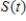during the first year. (Multiple Choice)
4.8/5 (34)
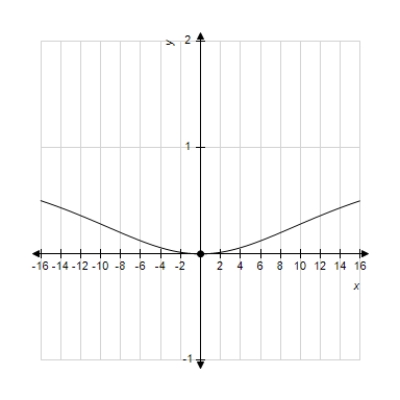
(34) Find the value of the derivative (if it exists) of the function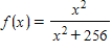 
at the extremum point 
. 
at the extremum point . (Multiple Choice)
4.9/5 (32)
(32) Find all relative extrema of the function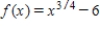 
. Use the Second Derivative Test where applicable.
. Use the Second Derivative Test where applicable. (Multiple Choice)
4.9/5 (28)
(28) Find all relative extrema of the function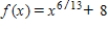 
. Use the Second Derivative Test where applicable.
. Use the Second Derivative Test where applicable. (Multiple Choice)
4.7/5 (33)
(33) The graph of f is shown. Graph f, f' and f'' on the same set of coordinate axes.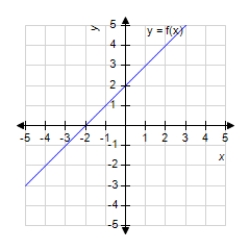 
(Multiple Choice)
4.8/5 (36)
(36) Which of the following functions passes through the point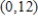 
and satisfies 
?
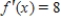and satisfies ? (Multiple Choice)
4.9/5 (38)
(38) The range R of a projectile is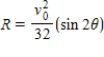 
where ν0 is the initial velocity in feet per second and θ is the angle of elevation. If ν0 = 2200 feet per second and θ is changed from 12° to 13° use differentials to approximate the change in the range. Round your answer to the nearest integer.
where ν0 is the initial velocity in feet per second and θ is the angle of elevation. If ν0 = 2200 feet per second and θ is changed from 12° to 13° use differentials to approximate the change in the range. Round your answer to the nearest integer. (Multiple Choice)
4.9/5 (34)
(34) 
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)