Exam 6: Differential Equations
Exam 1: Preparation for Calculus125 Questions
Exam 2: Limits and Their Properties85 Questions
Exam 3: Differentiation193 Questions
Exam 4: Applications of Differentiation154 Questions
Exam 5: Integration184 Questions
Exam 6: Differential Equations93 Questions
Exam 7: Applications of Integration119 Questions
Exam 8: Integration Techniques and Improper Integrals130 Questions
Exam 9: Infinite Series181 Questions
Exam 10: Conics, Parametric Equations, and Polar Coordinates114 Questions
Exam 11: Vectors and the Geometry of Space130 Questions
Exam 12: Vector-Valued Functions85 Questions
Exam 13: Functions of Several Variables173 Questions
Exam 14: Multiple Integration143 Questions
Exam 15: Vector Anal142 Questions
Select questions type
Each of the following graphs is from a logistic function  . Which one has the smallest value of b?
. Which one has the smallest value of b?
Free
(Multiple Choice)
5.0/5  (33)
(33)
Correct Answer:
E
At time 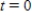 minutes, the temperature of an object is
minutes, the temperature of an object is 
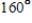 F. The temperature of the object is changing at the rate given by the differential equation
F. The temperature of the object is changing at the rate given by the differential equation 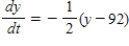 . Use Euler's Method to approximate the particular solutions of this differential equation at
. Use Euler's Method to approximate the particular solutions of this differential equation at 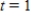 . Use a step size of
. Use a step size of 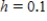 . Round your answer to one decimal place.
. Round your answer to one decimal place.
Free
(Multiple Choice)
4.8/5  (31)
(31)
Correct Answer:
E
The number of bacteria in a culture is increasing according to the law of exponential growth. After 2 hours there are 135 bacteria in the culture and after 4 hours there are 390 bacteria in the culture. Answer the following questions, rounding numerical answers to four decimal places.
(i) Find the initial population.
(ii) Write an exponential growth model for the bacteria population. Let t represent time in hours.
(iii) Use the model to determine the number of bacteria after 8 hours.
(iv) After how many hours will the bacteria count be 25,000?
Free
(Multiple Choice)
4.9/5  (22)
(22)
Correct Answer:
C
Write and solve the differential equation that models the following verbal statement. Evaluate the solution at the specified value of the independent variable, rounding your answer to four decimal places:
The rate of change of Q is proportional to Q. When 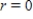 ,
, 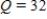 and when
and when 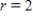 ,
, 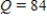 . What is the value of Q when
. What is the value of Q when 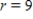 ?
?
(Multiple Choice)
4.8/5  (36)
(36)
Determine whether the function 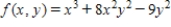 is homogeneous and determine its degree if it is.
is homogeneous and determine its degree if it is.
(Multiple Choice)
4.9/5  (32)
(32)
The logistic function 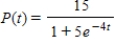 models the growth of a population. Determine when the population reaches one-half of the maximum carrying capacity. Round your answer to three decimal places.
models the growth of a population. Determine when the population reaches one-half of the maximum carrying capacity. Round your answer to three decimal places.
(Multiple Choice)
4.9/5  (35)
(35)
Sketch a few solutions of the differential equation on the slope field and then find the general solution analytically. 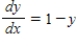
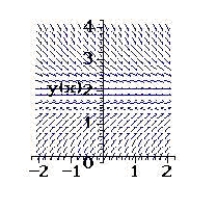
(Multiple Choice)
4.8/5  (43)
(43)
Use Euler's Method to make a table of values for the approximate solution of the following differential equation with specified initial value. Use 5 steps of size 0.05.  ,
, 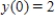
(Multiple Choice)
4.7/5  (39)
(39)
The initial investment in a savings account in which interest is compounded continuously is $803. If the time required to double the amount is 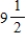 years, what is the annual rate? Round your answer to two decimal places.
years, what is the annual rate? Round your answer to two decimal places.
(Multiple Choice)
4.9/5  (34)
(34)
The logistic function  models the growth of a population. Identify the value of k.
models the growth of a population. Identify the value of k.
(Multiple Choice)
4.9/5  (41)
(41)
Match the logistic differential equation and initial condition with the graph of its solution shown below. 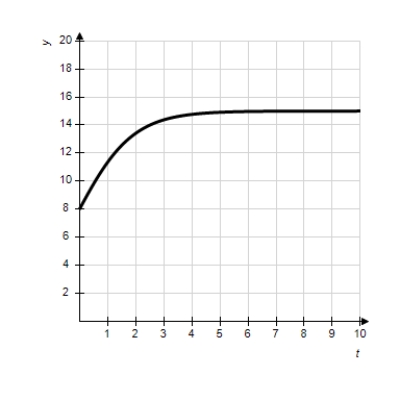
(Multiple Choice)
5.0/5  (36)
(36)
Use integration to find a general solution of the differential equation 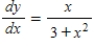 .
.
(Multiple Choice)
4.7/5  (26)
(26)
Sketch a few solutions of the differential equation on the slope field and then find the general solution analytically. 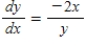
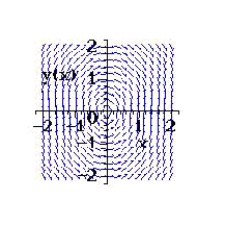
(Multiple Choice)
4.8/5  (33)
(33)
A 300-gallon tank is half full of distilled water. At time 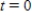 , a solution containing 0.5 pound of concentrate per gallon enters the tank at the rate of 6 gallons per minute, and the well-stirred mixture is withdrawn at the rate of 4 gallons per minute. At the time the tank is full, how many pounds of concentrate will it contain? Round your answer to two decimal places.
, a solution containing 0.5 pound of concentrate per gallon enters the tank at the rate of 6 gallons per minute, and the well-stirred mixture is withdrawn at the rate of 4 gallons per minute. At the time the tank is full, how many pounds of concentrate will it contain? Round your answer to two decimal places.
(Multiple Choice)
4.8/5  (40)
(40)
A 300-gallon tank is full of a solution containing 55 pounds of concentrate. Starting at time 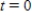 distilled water is added to the tank at a rate of 30 gallons per minute, and the well-stirred solution is withdrawn at the same rate. Find the quantity of the concentrate in the solution as
distilled water is added to the tank at a rate of 30 gallons per minute, and the well-stirred solution is withdrawn at the same rate. Find the quantity of the concentrate in the solution as 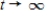 .
.
(Multiple Choice)
5.0/5  (36)
(36)
Find the function 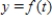 passing through the point
passing through the point  with the first derivative
with the first derivative 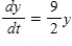 .
.
(Multiple Choice)
4.7/5  (33)
(33)
A conservation organization releases 20 wolves into a preserve. After 2 years, there are 35 wolves in the preserve. The preserve has a carrying capacity of 125. Determine the time it takes for the population to reach 80.
(Multiple Choice)
5.0/5  (38)
(38)
Showing 1 - 20 of 93
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)