Exam 7: Applications of Integration
Exam 1: Functions and Limits54 Questions
Exam 2: Derivatives50 Questions
Exam 3: Inverse Functions: Exponential, Logarithmic, and Inverse Trigonometric Functions43 Questions
Exam 4: Applications of Differentiation68 Questions
Exam 5: Integrals33 Questions
Exam 6: Techniques of Integration46 Questions
Exam 7: Applications of Integration69 Questions
Exam 8: Series51 Questions
Exam 9: Parametric Equations and Polar Coordinates30 Questions
Exam 10: Vectors and the Geometry of Space68 Questions
Exam 11: Partial Derivatives73 Questions
Exam 12: Multiple Integrals59 Questions
Exam 13: Vector Calculus54 Questions
Select questions type
You are given the shape of the vertical ends of a trough that is completely filled with water. Find the force exerted by the water on one end of the trough. (The weight density of water is 62.4 lb/ft3.) 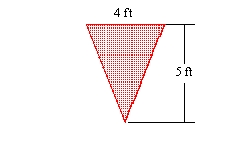
Free
(Multiple Choice)
4.8/5  (35)
(35)
Correct Answer:
B
The base of S is the parabolic region 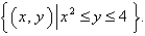 Cross-sections perpendicular to the y axis are squares.Find the volume of S.
Cross-sections perpendicular to the y axis are squares.Find the volume of S.
Free
(Short Answer)
4.9/5  (34)
(34)
Correct Answer:
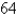
Find the volume of the solid obtained by rotating the region bounded by 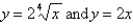 about the line
about the line 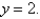
Free
(Multiple Choice)
4.6/5  (26)
(26)
Correct Answer:
C
Find the center of mass of the system comprising masses mk located at the points Pk in a coordinate plane. Assume that mass is measured in grams and distance is measured in centimeters.m1 = 3, m2 = 4, m3 = 5
P1 (-3, 5), P2 (3, 4), P3 (-4, 1)
(Short Answer)
5.0/5  (35)
(35)
Find the volume of the solid obtained by rotating the region bounded by  about the x-axis.
about the x-axis.
(Multiple Choice)
4.8/5  (41)
(41)
True or False?
The volume of a solid torus (the donut-shaped solid shown in the figure) with r =  and R =
and R = 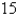 is
is 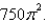
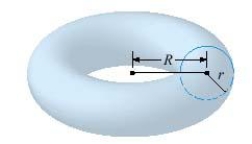
(True/False)
4.8/5  (46)
(46)
Choose the differential equation corresponding to this direction field. 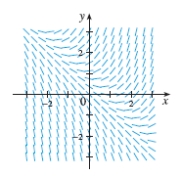
(Multiple Choice)
4.8/5  (42)
(42)
Suppose you make napkin rings by drilling holes with different diameters through two wooden balls (which also have different diameters). You discover that both napkin rings have the same height h as shown in the figure. Use cylindrical shells to compute the volume of a napkin ring created by drilling a hole with radius d through the center of a sphere of radius D and express the answer in terms of 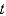 .
. 
(Multiple Choice)
4.9/5  (38)
(38)
Use a computer algebra system to find the exact volume of the solid obtained by rotating the region bounded by the given curves about the specified line. 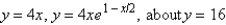
(Short Answer)
4.8/5  (29)
(29)
One model for the spread of an epidemic is that the rate of spread is jointly proportional to the number of infected people and the number of uninfected people. In an isolated town of 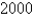 inhabitants,
inhabitants,  people have a disease at the beginning of the week and
people have a disease at the beginning of the week and 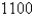 have it at the end of the week. How long does it take for
have it at the end of the week. How long does it take for 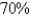 of the population to be infected?
of the population to be infected?
(Multiple Choice)
4.8/5  (33)
(33)
Find the area of the surface obtained by rotating the circle 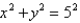 about the line
about the line 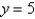 .
.
(Multiple Choice)
4.8/5  (35)
(35)
Set up, but do not evaluate, an integral for the area of the surface obtained by rotating the curve about the given axis. 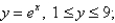 y-axis
y-axis
(Multiple Choice)
4.9/5  (31)
(31)
Find the area of the surface obtained by revolving the given curve about the y-axis.x = ![Find the area of the surface obtained by revolving the given curve about the y-axis.x = on [0, 2]](https://storage.examlex.com/TB8390/11eb7c01_16d2_d7cc_acd2_f7908fadf830_TB8390_11.jpg) on [0, 2]
on [0, 2]
(Short Answer)
4.8/5  (27)
(27)
Use the method of cylindrical shells to find the volume of solid obtained by rotating the region bounded by the given curves about the x-axis. 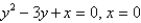
(Short Answer)
4.9/5  (34)
(34)
An aquarium is 4 ft long, 3 ft wide, and 2 ft deep. If the aquarium is filled with water, find the force exerted by the water (a) on the bottom of the aquarium, (b) on the longer side of the aquarium, and (c) on the shorter side of the aquarium. (The weight density of water is 62.4 lb/ft3.)
(Short Answer)
4.8/5  (31)
(31)
Find the volume of the frustum of a pyramid with square base of side  square top of side
square top of side 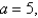 and height
and height 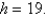
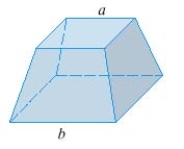
(Short Answer)
4.8/5  (37)
(37)
Find the area of the region bounded by the parabola 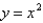 , the tangent line to this parabola at
, the tangent line to this parabola at 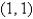 , and the x axis.
, and the x axis.
(Multiple Choice)
4.8/5  (31)
(31)
A steady wind blows a kite due west. The kite's height above ground from horizontal position 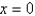 to
to 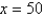 ft is given by
ft is given by 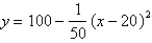 .Find the distance traveled by the kite.Give your answer rounded to two decimal places.
.Find the distance traveled by the kite.Give your answer rounded to two decimal places.
(Short Answer)
4.8/5  (31)
(31)
Find the volume of the solid obtained by rotating the region bounded by the given curves about the specified line. 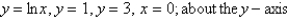
(Multiple Choice)
4.8/5  (30)
(30)
Showing 1 - 20 of 69
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)