based on the following ANOVA summary table (fixed effects): $\begin{array}{cccc} \hline \text { Source } & d f & \text { MS } & F \\ \hline \mathrm{A} & 2 & 15 & 3.0 \\ \mathrm{~B} & 3 & 10 & 2.0 \\ \mathrm{AB} & 6 & 3 & 0.6 \\ \text { Within } & 120 & 5 & \\ \hline \end{array}$ -How many cells are there in the design?

A) 6 B) 8 C) 9 D) None of the above A) 6 B) 8 C) 9 D) None of the above D

based on the following ANOVA summary table (fixed effects): $\begin{array}{cccc} \hline \text { Source } & d f & M S & F \\ \hline \mathrm{A} & 5 & 18.0 & 6.0 \\ \mathrm{~B} & 1 & 13.5 & 4.5 \\ \mathrm{AB} & 5 & 15.0 & 5.0 \\ \text { Within } & 60 & 3.0 & \\ \hline \end{array}$ -How many cells are there in the design?

A) 6 B) 10 C) 12 D) None of the above A) 6 B) 10 C) 12 D) None of the above C

based on the following ANOVA summary table (fixed effects): $\begin{array}{cccc} \hline \text { Source } & d f & M S & F \\ \hline \mathrm{A} & 5 & 18.0 & 6.0 \\ \mathrm{~B} & 1 & 13.5 & 4.5 \\ \mathrm{AB} & 5 & 15.0 & 5.0 \\ \text { Within } & 60 & 3.0 & \\ \hline \end{array}$ -For which source of variation is the null hypothesis rejected at the .05 level of significance?

A) A B) B C) AB D) All of the above. A) A B) B C) AB D) All of the above.

A researcher wanted to examine the effects of types of diet (factor A) and the age (factor B) on the weight gains of sheep. Diet type has four levels (i.e., four types of diet) and age has two levels (younger than one year old and older than one year old). Five sheep were assigned to each of the eight cells. The following are the scores (weight gains) from the individual cells: A1B1: 11.8, 11.7, 11.1, 10.7, 10.4 A1B2: 11.1, 9.8, 9.5, 9.2, 8.8 A2B1: 10.2, 10.0, 8.7, 8.1, 7.3 A2B2: 8.6, 8.2, 7.7, 7.4, 5.6 A3B1: 12.0, 10.8, 10.5, 10.2, 10.2 A3B2: 10.7, 9.8, 9.7, 9.5, 8.9 A4B1: 9.8, 9.6, 9.4, 9.1, 7.9 A4B2: 8.0, 7.4, 7.4, 6.7, 5.8 Use SPSS to conduct a two-factor fixed-effects ANOVA to determine if there are any effects due to diet type, diet amount, or the interaction ($\alpha$= 0.05). Conduct Tukey HSD post hoc comparisons, if necessary. (Presume all assumptions of ANOVA are satisfied.)

Procedure: Create a data set with three

based on the following ANOVA summary table (fixed effects): $\begin{array}{cccc} \hline \text { Source } & d f & M S & F \\ \hline \mathrm{A} & 5 & 18.0 & 6.0 \\ \mathrm{~B} & 1 & 13.5 & 4.5 \\ \mathrm{AB} & 5 & 15.0 & 5.0 \\ \text { Within } & 60 & 3.0 & \\ \hline \end{array}$ -The total sample size for the design is which one of the following?

A) 72 B) 74 C) 77 D) None of the above A) 72 B) 74 C) 77 D) None of the above

Exam 13: Factorial Anova: Fixed-Effects Model

Complete the following ANOVA summary table for a two-factor fixed-effects ANOVA, where there are three levels of factor A (teaching method) and two levels of factor B (time of class). Each cell includes six students and $\alpha$ = .05. Source SS df MS F Critical Value Decision A 6.5 B 5.2 Within 39 Total 65

Free

(Essay)

4.8/5

(33)

Question 1

Correct Answer:

Verified

There are 3 levels of factor A, so J = 3. There are 2 levels of factor B, so K = 2. Each cell has 6 students, so n = 6. N = 3*2*6 = 36.
df_A= J - 1 = 3 - 1 = 2, df_B = K - 1 = 2 - 1 = 1, df_A_B = (J - 1)(K - 1) = 2*1 = 2,
df_with = N - JK = 36 - 3*2 = 30, df_total = N - 1 = 36 - 1 = 35.
SS_A = df_A*MS_A = 2*6.5 = 13, SS_AB = df_AB*MS_AB = 2*5.2 = 10.4,
SS_B = SS_total - SS_A - SS_AB - SS_with = 65 - 13 - 10.4 - 39 = 2.6.
MS_B = SS_B/df_B = 2.6/1 = 2.6; MS_with = SS_with/df_with = 39/30 = 1.3.
F_A = MS_A/MS_with = 6.5/1.3 = 5; critical value = _.05F_2,30 = 3.32 < F_A, reject H₀.
F_B=MS_B/MS_with = 2.6/1.3 = 2; critical value = _.05F₁_,₃₀ = 4.17 > F_B, fail to reject H₀.
F_AB =MS_AB/MS_with =5.2/1.3 = 4; critical value = _.05F_2,₃₀ = 3.32 < F_AB, reject H₀.
$\begin{array}{ccccccc}\hline \text { Source } & S S & d f & M S & F & \text { Critical Value } & \text { Decision } \\\hline \mathrm{A} & 13.0 & 2 & 6.5 & 5 & .05 F_{2,30}=3.32 & \text { Reject } H_{0} \\\mathrm{~B} & 2.6 & 1 & 2.6 & 2 & .05 F_{1,30}=4.17 & \text { Fail to reject } H_{0} \\\mathrm{AB} & 10.4 & 2 & 5.2 & 4 & .05 F_{2,30}=3.32 & \text { Reject } H_{0} \\\text { Within } & 39.0 & 30 & 1.3 & & & \\\text { Total } & 65.0 & 35 & & & & \\\hline\end{array}$

based on the following ANOVA summary table (fixed effects): Source df MS F 2 15 3.0 3 10 2.0 6 3 0.6 Within 120 5 -How many cells are there in the design?

Free

(Multiple Choice)

4.9/5

(40)

Question 2

Correct Answer:

Verified

D

based on the following ANOVA summary table (fixed effects): Source df MS F 5 18.0 6.0 1 13.5 4.5 5 15.0 5.0 Within 60 3.0 -How many cells are there in the design?

Free

(Multiple Choice)

4.8/5

(40)

Question 3

Correct Answer:

Verified

C

based on the following ANOVA summary table (fixed effects): Source df MS F 5 18.0 6.0 1 13.5 4.5 5 15.0 5.0 Within 60 3.0 -For which source of variation is the null hypothesis rejected at the .05 level of significance?

(Multiple Choice)

4.9/5

(39)

Question 4

A researcher wanted to examine the effects of types of diet (factor A) and the age (factor B) on the weight gains of sheep. Diet type has four levels (i.e., four types of diet) and age has two levels (younger than one year old and older than one year old). Five sheep were assigned to each of the eight cells. The following are the scores (weight gains) from the individual cells: A₁B₁: 11.8, 11.7, 11.1, 10.7, 10.4 A₁B₂: 11.1, 9.8, 9.5, 9.2, 8.8 A₂B₁: 10.2, 10.0, 8.7, 8.1, 7.3 A₂B₂: 8.6, 8.2, 7.7, 7.4, 5.6 A₃B₁: 12.0, 10.8, 10.5, 10.2, 10.2 A₃B₂: 10.7, 9.8, 9.7, 9.5, 8.9 A₄B₁: 9.8, 9.6, 9.4, 9.1, 7.9 A₄B₂: 8.0, 7.4, 7.4, 6.7, 5.8 Use SPSS to conduct a two-factor fixed-effects ANOVA to determine if there are any effects due to diet type, diet amount, or the interaction ( $\alpha$ = 0.05). Conduct Tukey HSD post hoc comparisons, if necessary. (Presume all assumptions of ANOVA are satisfied.)

(Essay)

4.8/5

(28)

Question 5

In a two-factor fixed-effects ANOVA, the interaction effect is definitely not present when

(Multiple Choice)

4.9/5

(33)

Question 6

In a three-factor fixed-effects ANOVA, the three-way interaction effect is found to be significant. Which of the following statements is always true?

(Multiple Choice)

4.7/5

(34)

Question 7

In a two-factor ANOVA, df_A = 2, df_B = 3, and each cell has five observations. what is df_AB?

(Multiple Choice)

4.8/5

(39)

Question 8

A researcher used ANOVA to examine the effects of different types of diet on the weight gains of sheep. Specifically, he wants to see if the effect of diet type is different for sheep of different ages. Ten sheep were assigned to each of the four diet groups. Within each diet group, half of the sheep were younger than one year old, and the other half were older than one year old. How many factors are in this experiment and how many levels do these factors have?

(Multiple Choice)

4.9/5

(36)

Question 9

Adding a second factor to a one-factor design may

(Multiple Choice)

4.8/5

(34)

Question 10

based on the following plots of cell means. Assume that the within-cell variation is very small. 1) 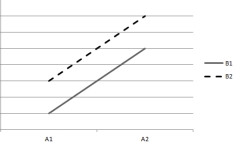2) 3) 4) -Which plot indicates that both main effects are significant but the interaction effect is nonsignificant?

(Multiple Choice)

4.7/5

(38)

Question 11

Complete the following ANOVA summary table for a two-factor fixed-effects ANOVA, where there are two levels of factor A (type of counseling) and four levels of factor B (frequency of counseling). Each cell includes six people and $\alpha$ = .01. Source SS df MS F Critical Value Decision 60 6 60 Within Total 640

(Essay)

4.8/5

(34)

Question 12

Using the same data set (J = 3, K = 2, n = 10), Jane conducted two one-factor ANOVA, whereas Joe conducted a two-factor ANOVA. Which of the following statements is not true?

(Multiple Choice)

4.9/5

(31)

Question 13

In a two-factor fixed-effects ANOVA, F_A = 3, F_B = 3.5, df_A = 3, df_B = 3. If the null hypothesis for factor A is rejected at .05 level of significance, the null hypothesis for factor B will be

(Multiple Choice)

4.9/5

(38)

Question 14

In a two-factor ANOVA, df_A = 2, df_B = 3, and each cell has five observations. what is df_with?

(Multiple Choice)

4.9/5

(34)

Question 15

Which of the following situations would result in the greatest generalizability of the main effect for factor B across the levels of factor A?

(Multiple Choice)

4.8/5

(32)

Question 16

A small-scale taste test is conducted to find out how the levels of moisture (factor A) and sweetness (factor B) of the cakes are related to people's preference to the cake. Participants' gender (factor C) is also considered. Each factor consists of two levels. Thirty-two participants are assigned to eight cells (i.e., four per cell), one for each of the factor combinations. The following are the scores (rating of the cakes by the participants) from the individual cells: A₁B₁C₁: 64, 61, 65, 70 A₁B₁C₂: 63, 60, 64, 68 A₁B₂C₁: 73, 76, 72, 71 A₁B₂C₂: 72, 75, 79, 77 A₂B₁C₁: 72, 78, 81, 75 A₂B₁C₂: 74, 73, 75, 69 A₂B₂C₁: 98, 97, 93, 89 A₂B₂C₂: 86, 93, 88, 94 Use SPSS to conduct a three-factor fixed-effects ANOVA ( $\alpha$ = 0.01). If there is (are) any significant interaction(s), graph and interpret the interaction(s).

(Essay)

4.8/5

(29)

Question 17

The results of a two-factor ANOVA (J = 3, K = 2) show that both main effects are significant, but the interaction is not significant. We need to

(Multiple Choice)

4.9/5

(28)

Question 18

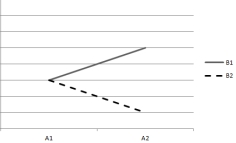
based on the following plots of cell means. Assume that the within-cell variation is very small. 1) 2) 3) 4) -Which plot(s) indicate(s) significant interaction effects?

(Multiple Choice)

4.9/5

(35)

Question 19

based on the following ANOVA summary table (fixed effects): Source df MS F 5 18.0 6.0 1 13.5 4.5 5 15.0 5.0 Within 60 3.0 -The total sample size for the design is which one of the following?

(Multiple Choice)

4.7/5

(34)

Question 20

based on the following ANOVA summary table (fixed effects): Source df MS F 2 15 3.0 3 10 2.0 6 3 0.6 Within 120 5 -How many cells are there in the design?

based on the following ANOVA summary table (fixed effects): Source df MS F 5 18.0 6.0 1 13.5 4.5 5 15.0 5.0 Within 60 3.0 -How many cells are there in the design?

based on the following ANOVA summary table (fixed effects): Source df MS F 5 18.0 6.0 1 13.5 4.5 5 15.0 5.0 Within 60 3.0 -For which source of variation is the null hypothesis rejected at the .05 level of significance?

In a two-factor fixed-effects ANOVA, the interaction effect is definitely not present when

In a three-factor fixed-effects ANOVA, the three-way interaction effect is found to be significant. Which of the following statements is always true?

In a two-factor ANOVA, df_A = 2, df_B = 3, and each cell has five observations. what is df_AB?

Adding a second factor to a one-factor design may

based on the following plots of cell means. Assume that the within-cell variation is very small. 1) 2) 3) 4) -Which plot indicates that both main effects are significant but the interaction effect is nonsignificant?

Using the same data set (J = 3, K = 2, n = 10), Jane conducted two one-factor ANOVA, whereas Joe conducted a two-factor ANOVA. Which of the following statements is not true?

In a two-factor fixed-effects ANOVA, F_A = 3, F_B = 3.5, df_A = 3, df_B = 3. If the null hypothesis for factor A is rejected at .05 level of significance, the null hypothesis for factor B will be

In a two-factor ANOVA, df_A = 2, df_B = 3, and each cell has five observations. what is df_with?

Which of the following situations would result in the greatest generalizability of the main effect for factor B across the levels of factor A?

The results of a two-factor ANOVA (J = 3, K = 2) show that both main effects are significant, but the interaction is not significant. We need to

based on the following plots of cell means. Assume that the within-cell variation is very small. 1) 2) 3) 4) -Which plot(s) indicate(s) significant interaction effects?

based on the following ANOVA summary table (fixed effects): Source df MS F 5 18.0 6.0 1 13.5 4.5 5 15.0 5.0 Within 60 3.0 -The total sample size for the design is which one of the following?

Introduction

Data Representation

Univariate Population Parameters and Sample Statistics

Normal Distribution and Standard Scores

Introduction to Probability and Sample Statistics

Inferences About a Single Mean

Inferences About the Difference Between Two Means

Inferences About Proportions

Inferences About Variances

Bivariate Measures of Association

One-Factor Anova: Fixed-Effects Model

Multiple Comparison Procedures

One-Factor Fixed-Effects Ancova With Single Covariate

Random- and Mixed-Effects Analysis of Variance Models

Hierarchical and Randomized Block Analysis of Variance Models

Simple Linear Regression

Multiple Regression

Logistic Regression

Filters

Exam 13: Factorial Anova: Fixed-Effects Model

based on the following ANOVA summary table (fixed effects): Source df MS F 2 15 3.0 3 10 2.0 6 3 0.6 Within 120 5 -How many cells are there in the design?

based on the following ANOVA summary table (fixed effects): Source df MS F 5 18.0 6.0 1 13.5 4.5 5 15.0 5.0 Within 60 3.0 -How many cells are there in the design?

based on the following ANOVA summary table (fixed effects): Source df MS F 5 18.0 6.0 1 13.5 4.5 5 15.0 5.0 Within 60 3.0 -For which source of variation is the null hypothesis rejected at the .05 level of significance?

In a two-factor fixed-effects ANOVA, the interaction effect is definitely not present when

In a three-factor fixed-effects ANOVA, the three-way interaction effect is found to be significant. Which of the following statements is always true?

In a two-factor ANOVA, dfA = 2, dfB = 3, and each cell has five observations. what is dfAB?

Adding a second factor to a one-factor design may

based on the following plots of cell means. Assume that the within-cell variation is very small. 1) 2) 3) 4) -Which plot indicates that both main effects are significant but the interaction effect is nonsignificant?

Using the same data set (J = 3, K = 2, n = 10), Jane conducted two one-factor ANOVA, whereas Joe conducted a two-factor ANOVA. Which of the following statements is not true?

In a two-factor fixed-effects ANOVA, FA = 3, FB = 3.5, dfA = 3, dfB = 3. If the null hypothesis for factor A is rejected at .05 level of significance, the null hypothesis for factor B will be

In a two-factor ANOVA, dfA = 2, dfB = 3, and each cell has five observations. what is dfwith?

Which of the following situations would result in the greatest generalizability of the main effect for factor B across the levels of factor A?

The results of a two-factor ANOVA (J = 3, K = 2) show that both main effects are significant, but the interaction is not significant. We need to

based on the following plots of cell means. Assume that the within-cell variation is very small. 1) 2) 3) 4) -Which plot(s) indicate(s) significant interaction effects?

based on the following ANOVA summary table (fixed effects): Source df MS F 5 18.0 6.0 1 13.5 4.5 5 15.0 5.0 Within 60 3.0 -The total sample size for the design is which one of the following?

Introduction

Data Representation

Univariate Population Parameters and Sample Statistics

Normal Distribution and Standard Scores

Introduction to Probability and Sample Statistics

Inferences About a Single Mean

Inferences About the Difference Between Two Means

Inferences About Proportions

Inferences About Variances

Bivariate Measures of Association

One-Factor Anova: Fixed-Effects Model

Multiple Comparison Procedures

One-Factor Fixed-Effects Ancova With Single Covariate

Random- and Mixed-Effects Analysis of Variance Models

Hierarchical and Randomized Block Analysis of Variance Models

Simple Linear Regression

Multiple Regression

Logistic Regression

Filters

In a two-factor ANOVA, df_A = 2, df_B = 3, and each cell has five observations. what is df_AB?

In a two-factor fixed-effects ANOVA, F_A = 3, F_B = 3.5, df_A = 3, df_B = 3. If the null hypothesis for factor A is rejected at .05 level of significance, the null hypothesis for factor B will be

In a two-factor ANOVA, df_A = 2, df_B = 3, and each cell has five observations. what is df_with?