Exam 16: Person-Centered Analysis

What is the purpose of a cluster analysis?

Suppose that I performed a latent-class analysis on several "true-false" questions designed to measure students' engagement with school. I got the results presented in the table below: Survey Questions Class 1(n=42) Class 2(n=58) Class 3(n=40) 30\% 41\% 29\% 1. I usually pay close attention in class. .92 .25 .78 2. I participate often in class .45 75 .72 discussions. 3. I always bring my materials (book, .24 .88 .83 pencils, paper) to class. 4. I'm often excited to be in class. .77 .14 .63 5. I often talk with my friends and .85 .32 .61 parents about ideas we learn about in school. 6. I almost always turn in my .35 .92 .45 homework and other assignments on time. a. How would you describe these three latent classes?

What is a "conditional probability" in a latent-class analysis?

What kind of variables are appropriate for use in a latent-class analysis?

What does "person-centered analysis" mean and how does it differ from "variable-centered analysis?"

Suppose I performed a cluster analysis and I got a 3-cluster solution and a 4-cluster solution. Each solution is presented in the graphs below: 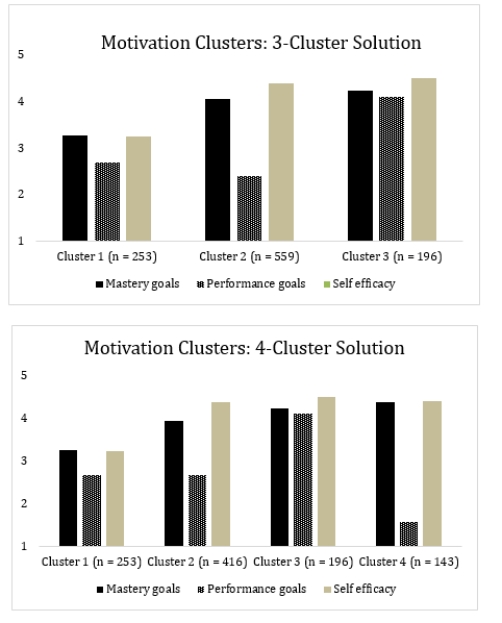a. What is the main difference between these solutions? b. Which solution do you think is best? Why?

Suppose that I happen to know that in the population of current college students in the U.S., the average level of satisfaction with life is 8.00, with a standard deviation of 3.00. (Satisfaction with life is measured on a scale from 1 to 15 with 1 = "totally unsatisfied" and 15 = "totally satisfied".) I suspect that college students in California have higher satisfaction with life. We have the sun, we have the mountains, we have the great stats professors. What's not to like? So I select a random sample of 36 college students from California and measure their satisfaction with life. This sample has a mean of 9.00 and a standard deviation of 2.5. Please answer the following questions based on this research question and data. a. Suppose that one of the students in the California sample scored a 5.00 on his life satisfaction. Calculate two z scores for him, one for his score in the sample and one for his score in the population. Wrap words around each of these z scores. What do they tell you? b. What is the probability of me reaching into the population and randomly selecting a student with a life-satisfaction score of 10 or higher? c. What is/are the score(s) that mark the extreme 10% of the distribution for the population on the life-satisfaction measure? d. Please write the null and alternative hypotheses for my research question and tell me why you wrote them as you did. e. Calculate the standard error of the mean for the sample and wrap words around it. f. Wrap words around the standard deviation for the sample. What does it tell us? g. What is the probability of getting this sample mean by chance h. When I say that I have an alpha level of .05 (i.e., $\alpha$ = .05), what exactly do I mean? i. What proportion of randomly selected samples of n = 36 from this population would be expected to have a mean between 6.5 and 9.0? j. In the term standard error, what exactly does error mean? Error in what?