Exam 13: Factorial Anova: Fixed-Effects Mode
Exam 1: Introduction9 Questions
Exam 2: Data Representation7 Questions
Exam 3: Univariate Population Parameters and Sample Statistics8 Questions
Exam 4: The Normal Distribution and Standard Scores5 Questions
Exam 5: Introduction to Probability and Sample Statistics4 Questions
Exam 6: Introduction to Hypothesis Testing: Inferences About a Single Mean7 Questions
Exam 7: Inferences About the Difference Between Two Means7 Questions
Exam 8: Inferences About Proportions6 Questions
Exam 9: Inferences About Variances8 Questions
Exam 10: Bivariate Measures of Association5 Questions
Exam 11: One-Factor Anova: Fixed-Effects Mode8 Questions
Exam 12: Multiple Comparison Procedures13 Questions
Exam 13: Factorial Anova: Fixed-Effects Mode16 Questions
Exam 14: One Factor Fixed-Effects Ancova With Single Covariate19 Questions
Exam 15: Random- and Mixed-Effects Analysis of Variance Models7 Questions
Exam 16: Hierarchical and Randomized Block Analysis of Variance Models12 Questions
Exam 17: Simple Linear Regression8 Questions
Exam 18: Multiple Linear Regression10 Questions
Exam 19: Logistic Regression15 Questions
Exam 20: Mediation and Moderation33 Questions
Select questions type
Complete the following ANOVA summary table for a two-factor fixed-effects ANOVA, where there are two levels of factor A (type of counseling) and four levels of factor B (frequency of counseling). Each cell includes six people and = .01.
Source SS df MS F Critical Value Decision 60 6 60 Within Total 640
Free
(Essay)
4.9/5  (39)
(39)
Correct Answer:
A has 2 levels, so J = 2. B has 4 levels, so K = 4.
Each cell has 6 people, so n = 6. N = 2*4*6 = 48.
dfA = J - 1 = 2 - 1 = 1, dfB = K - 1 = 4 - 1 = 3, dfAB = (J - 1)(K - 1) = 1*3 = 3, dfwith = N - JK = 48 - 2*4 = 40, dftotal = N - 1 = 48 - 1 = 47.
MSA = SSA/dfA = 60/1 = 60, MSAB = SSAB/dfAB = 60/3 = 20,
MSwith = MSA/FA = 60/6 = 10; SSwith = MSwith*dfwith = 10*40 = 400,
SSB = SStotal - SSA - SSAB - SSwith = 640 - 60 - 60 - 400 = 120;
MSB = MSB/FB = 120/3 = 40
FA = MSA/MSwith = 60/10 = 6; critical value = .01F1,40 = 7.31 > FA, fail to reject H0.
FB = MSB/MSwith = 40/10 = 4; critical value = .01F3,40 = 4.31 > FB, fail to reject H0.
FAB = MSAB/MSwith = 20/10 = 2; critical value = .01F3,40 = 4.31 > FAB, fail to reject H0.
Questions are based on the following plots of cell means. Assume that the within-cell variation is very small.
(1) 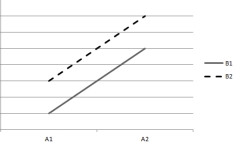 (2)
(2) 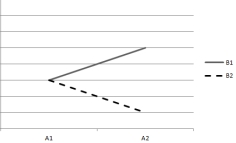 (3)
(3) 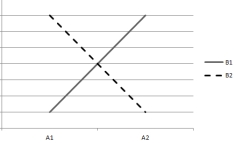 ( 4)
( 4)  -Which plot indicates that both main effects are significant but the interaction effect is nonsignificant?
-Which plot indicates that both main effects are significant but the interaction effect is nonsignificant?
Free
(Multiple Choice)
4.7/5  (31)
(31)
Correct Answer:
A
The results of a two-factor ANOVA (J = 3, K = 2) show that both main effects are significant, but the interaction is not significant. We need to
Free
(Multiple Choice)
4.9/5  (33)
(33)
Correct Answer:
D
Questions are based on the following ANOVA summary table (fixed effects):
Source df MS F 5 18.0 6.0 1 13.5 4.5 5 15.0 5.0 Within 60 3.0
-For which source of variation is the null hypothesis rejected at the .05 level of significance?
(Multiple Choice)
4.8/5  (30)
(30)
Questions are based on the following ANOVA summary table (fixed effects):
Source df MS F 5 18.0 6.0 1 13.5 4.5 5 15.0 5.0 Within 60 3.0
-The total sample size for the design is which one of the following?
(Multiple Choice)
4.9/5  (37)
(37)
Questions are based on the following plots of cell means. Assume that the within-cell variation is very small.
(1)  (2)
(2)  (3)
(3)  ( 4)
( 4)  -Which plot(s) indicate(s) significant interaction effects?
-Which plot(s) indicate(s) significant interaction effects?
(Multiple Choice)
4.8/5  (25)
(25)
Questions are based on the following ANOVA summary table (fixed effects):
Source df F 2 15 3.0 3 10 2.0 6 3 0.6 Within 120 5
-The total sample size for the design is which one of the following?
(Multiple Choice)
4.8/5  (23)
(23)
In a two-factor fixed-effects ANOVA with factors A and B, each of which have four categories, which one of the following occurs?
(Multiple Choice)
4.7/5  (30)
(30)
Questions are based on the following ANOVA summary table (fixed effects):
Source df F 2 15 3.0 3 10 2.0 6 3 0.6 Within 120 5
-For which source of variation is the null hypothesis rejected at the .10 level of significance?
(Multiple Choice)
4.8/5  (38)
(38)
Questions are based on the following plots of cell means. Assume that the within-cell variation is very small.
(1)  (2)
(2)  (3)
(3)  ( 4)
( 4)  -Which plot indicates that neither of the main effects are significant but the interaction effect is significant?
-Which plot indicates that neither of the main effects are significant but the interaction effect is significant?
(Multiple Choice)
4.8/5  (32)
(32)
Which of the following would be appropriate for a factorial ANOVA?
(Multiple Choice)
4.8/5  (32)
(32)
A researcher is interested in examining the extent to which there is a mean difference in lower-class undergraduate students' attitude toward instruction (interval) based on class modality (face-to-face, hybrid, online) and class standing (freshman or sophomore). Would conducting a factorial ANOVA be appropriate for this study?
(True/False)
4.8/5  (30)
(30)
Questions are based on the following ANOVA summary table (fixed effects):
Source df F 2 15 3.0 3 10 2.0 6 3 0.6 Within 120 5
-How many cells are there in the design?
(Multiple Choice)
4.9/5  (43)
(43)
Complete the following ANOVA summary table for a two-factor fixed-effects ANOVA, where there are three levels of factor A (teaching method) and two levels of factor B (time of class).
Each cell includes six students and = .05.
Source SS df MS F Critical Value Decision A 6.5 B 5.2 Within 39 Total 65
(Essay)
4.8/5  (25)
(25)
Complete the following ANOVA summary table for a two-factor fixed-effects ANOVA, where there are four levels of factor A (grade level) and three levels of factor B (textbook). Each cell includes 11 students and = .05.
Source SS df MS F Critical Value Decision 5 42 25 Within 240 Total Win
(Essay)
4.9/5  (38)
(38)
Questions are based on the following ANOVA summary table (fixed effects):
Source df MS F 5 18.0 6.0 1 13.5 4.5 5 15.0 5.0 Within 60 3.0
-How many cells are there in the design?
(Multiple Choice)
4.8/5  (36)
(36)
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)