Exam 4: Systems of Linear Equations
Exam 1: Review of the Real Number System431 Questions
Exam 2: Linear Equations, Inequalities, and Applications338 Questions
Exam 3: Linear Equations, Graphs, and Functions347 Questions
Exam 4: Systems of Linear Equations166 Questions
Exam 5: Exponents, Polynomials, and Polynomial Functions327 Questions
Exam 6: Factoring180 Questions
Exam 7: Rational Expressions and Functions233 Questions
Exam 8: Roots, Radicals, and Root Functions358 Questions
Exam 9: Quadratic Equations, Inequalities, and Functions339 Questions
Exam 10: Inverse, Exponential, and Logarithmic Functions292 Questions
Exam 11: Nonlinear Functions, Conic Sections, and Nonlinear Systems233 Questions
Exam 12: Further Topics in Algebra190 Questions
Exam 13: Appendices29 Questions
Select questions type
Write the word or phrase that best completes each statement or answers the question.
Provide an appropriate response
-What is indicated by the occurrence of a false statement such as " " when you solve a system of two linear equation (in two variables) using substitution?
Free
(Short Answer)
4.9/5  (34)
(34)
Correct Answer:
The system is inconsistent.
Solve the system by substitution. If the system is inconsistent or has dependent equations, say so.
-
Free
(Multiple Choice)
4.8/5  (45)
(45)
Correct Answer:
B
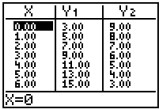
The table shown was generated by a graphing calculator. The functions defined by and y2 are linear. Based on the table, find the equation for .
(Multiple Choice)
4.8/5  (32)
(32)
Jill is away from Joe. Both begin to walk toward each other at the same time. Jill walks at per hour. If they meet in 3 hours, how fast is Joe walking?
(Multiple Choice)
4.8/5  (37)
(37)
Solve the problem using a system of equations.
-A company sells nuts in bulk quantities. When bought in bulk, peanuts sell for per pound, almonds for per pound, and cashews for per pound. Suppose a specialty shop wants a mixture of 210 pounds that will cost per pound. Find the number of pounds of each type of nut if the sum of the number of pounds of almonds and cashews is twice the number of pounds of peanuts. Round your answers to the nearest pound.
(Multiple Choice)
4.9/5  (40)
(40)
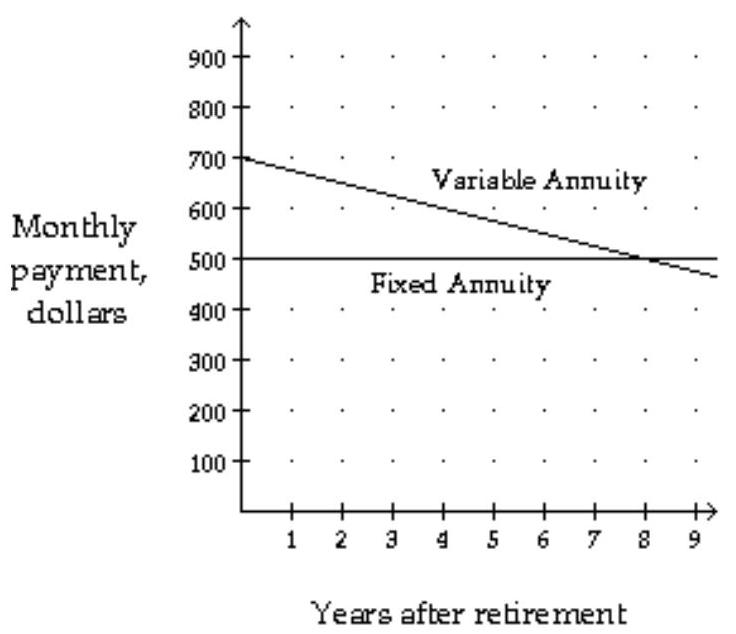
After retirement, Kelly's company offers her two options for receiving her retirement pension. According the the first plan, she will receive monthly payments from a variable annuity that initially pays per month then decreases each month at a rate of per month per year. Optionally, she may choose a plan that pays her a fixed amount of per month for the rest of her life. The monthly payments for the two plans are illustrated in the graph below. If Kelly's remaining life expectancy is 20 years, which plan would be the better choice?
(Multiple Choice)
4.7/5  (38)
(38)
Solve the system by substitution. If the system is inconsistent or has dependent equations, say so.
-

(Multiple Choice)
4.9/5  (34)
(34)
Tell how many solutions the system has. Do not actually solve.
-
(Multiple Choice)
4.8/5  (34)
(34)
A cabin cruiser travels 20 miles in the same time that a power boat travels 40 miles. The cruiser travels slower than the power boat. Find the speed of each boat.
(Multiple Choice)
4.9/5  (36)
(36)
John and Tony start from Grays lake at the same time and head for a town 10 miles away. John walks twice as fast as Tony and arrives 3 hours before Tony. Find how fast each walks.
(Multiple Choice)
4.8/5  (37)
(37)
Solve the system of equations. If the system is inconsistent or has dependent equations, say so.
-
(Multiple Choice)
5.0/5  (38)
(38)
Solve the system by elimination. If the system is inconsistent or has dependent equations, say so.
-
(Multiple Choice)
4.8/5  (41)
(41)
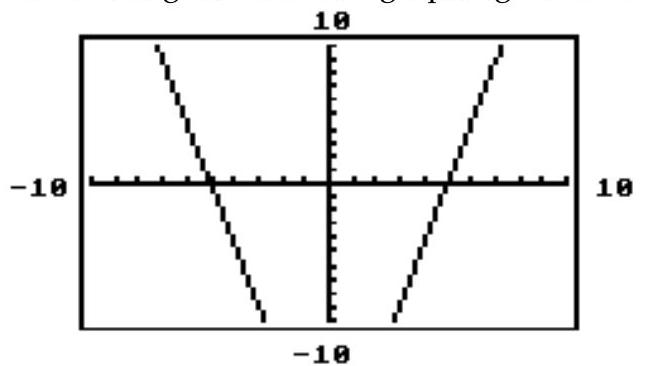
Which of the ordered pairs is the only possible solution for the system whose graphs are shown in the viewing window of a graphing calculator?
(Multiple Choice)
4.9/5  (37)
(37)
During the 1998-1999 Junior Hockey League season, the Sharks played 57 games. Together, their wins and losses totaled 51 . They tied 14 fewer games than they lost. How many games did they win that season?
(Multiple Choice)
4.8/5  (36)
(36)
Solve by any method. Assume and represent nonzero constants.
-
(Multiple Choice)
4.7/5  (40)
(40)
Which of the ordered pairs listed is the only possible solution for the system whose graphs are shown in the viewing window of a graphing calculator?

(Multiple Choice)
4.8/5  (32)
(32)
Michael's bank contains only nickels, dimes, and quarters. There are 58 coins in all, valued at . The number of nickels is 6 short of being three times the sum of the number of dimes and quarters together. How many dimes are in the bank?
(Multiple Choice)
4.8/5  (40)
(40)
Solve the system. Express the solution in the form .
-
-x+5y+z-5w =20 3x-y+5z+3w =20 x+y-5z-w =-26
(Multiple Choice)
4.8/5  (32)
(32)
Showing 1 - 20 of 166
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)