Exam 11: Conic Sections
Exam 1: Fundamentals229 Questions
Exam 2: Functions98 Questions
Exam 3: Polynomial and Rational Functions145 Questions
Exam 4: Exponential and Logarithmic Functions99 Questions
Exam 5: Trigonometric Functions: Unit Circle Approach100 Questions
Exam 6: Trigonometric Functions: Right Triangle Approach119 Questions
Exam 7: Analytic Trigonometry119 Questions
Exam 8: Polar Coordinates and Parametric Equations109 Questions
Exam 9: Vectors in Two and Three Dimensions96 Questions
Exam 10: Systems of Equations and Inequalities140 Questions
Exam 11: Conic Sections99 Questions
Exam 12: Sequences and Series100 Questions
Exam 13: Limits: a Preview of Calculus66 Questions
Select questions type
Write a polar equation of an ellipse with eccentricity and directrix .
Free
(Essay)
4.8/5  (37)
(37)
Correct Answer:
Use the discriminant to determine if the graph of the equation, is a parabola, an ellipse or a hyperbola, then use a rotation of axes to eliminate the - term, and sketch the graph.
Free
(Essay)
4.8/5  (38)
(38)
Correct Answer:
, so the graph is a hyperbola. To eliminate the - term, rotate through , such that , so . , . So 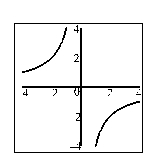
Find an equation for the ellipse whose foci are , and whose vertices are .
Free
(Essay)
4.8/5  (31)
(31)
Correct Answer:
, since the fociare , and since the vertices are , so
The major axis is horizontal, so the equation of the ellipse is
Use the discriminant to determine if the graph of the equation, is a parabola, an ellipse or a hyperbola, then use a rotation of axes to eliminate the - term, and sketch the graph.
(Essay)
4.9/5  (37)
(37)
(a) Find the eccentricity and directrix of the conic and graph the conic and its directrix.
(b) If this conic is rotated about the origin through and angle
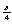 , write the resulting equation and draw its graph.
, write the resulting equation and draw its graph.
(Essay)
4.8/5  (34)
(34)
Use the discriminant to determine if the graph of the equation is a parabola, an ellipse or a hyperbola, then use a rotation of axes to eliminate the - term, and sketch the graph.
(Essay)
4.7/5  (35)
(35)
Find the focus, directrix, and focal diameter of the parabola , and sketch its graph.
(Essay)
4.7/5  (38)
(38)
Find an equation for the ellipse with endpoints of the major axis at , and a distance of between the foci.
(Essay)
4.8/5  (35)
(35)
By placing the origin at the center of Mercury's orbit and the Sun on the - axis at one of the foci, find the equation of Mercury's orbit given that the length of its major axis is m and the elliptical orbit has eccentricity .
(Essay)
4.8/5  (34)
(34)
Find an equation for the hyperbola that has foci and a transverse axis of length .
(Essay)
4.9/5  (34)
(34)
Find an equation for the hyperbola that has vertices and passes through the point .
(Essay)
4.7/5  (35)
(35)
Complete the square to determine whether the equation represents an ellipse, a parabola, a hyperbola, or a degenerate conic. Then sketch the graph of the equation. If the graph is an ellipse, find the center, foci, vertices, and lengths of the major and minor axes. If it is a parabola, find the vertex, focus and directrix. If it is a hyperbola, find the center, foci, vertices, and asymptotes. If the equation has no graph, explain why.
(Essay)
4.8/5  (37)
(37)
Use the discriminant to determine if the graph of the equation, is a parabola, an ellipse or a hyperbola, then use a rotation of axes to eliminate the - term, and sketch the graph.
(Essay)
4.8/5  (44)
(44)
Determine the - coordinates of if the axes are rotated through an angle .
(Essay)
4.8/5  (31)
(31)
Determine the - coordinates of if the axes are rotated through an angle .
(Essay)
5.0/5  (34)
(34)
Find the focus, directrix, and focal diameter of the parabola , and sketch its graph.
(Essay)
4.8/5  (38)
(38)
Showing 1 - 20 of 99
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)