Exam 7: Additional Topics in Integration
Exam 1: Preliminaries205 Questions
Exam 2: Functions, Limits and the Derivative269 Questions
Exam 3: Differentiation330 Questions
Exam 4: Applications of the Derivative182 Questions
Exam 5: Exponential and Logarithmic Functions278 Questions
Exam 6: Integration314 Questions
Exam 7: Additional Topics in Integration250 Questions
Exam 8: Calculus of Several Variables206 Questions
Select questions type
Find a bound on the error in approximating the given definite integral using the trapezoidal rule with n intervals. 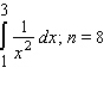 If necessary, round the answer to seven decimal places.
Find a bound on the error in approximating the given definite integral using the Simpson's rule with n intervals.
If necessary, round the answer to seven decimal places.
Find a bound on the error in approximating the given definite integral using the Simpson's rule with n intervals. 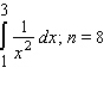 If necessary, round the answer to seven decimal places.
If necessary, round the answer to seven decimal places.
(Essay)
4.9/5  (37)
(37)
Tracy purchased a 15-year franchise for a computer outlet store that is expected to generate income at the rate of 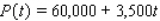 dollars/year. If the prevailing interest rate is 10% / year compounded continuously, find the present value of the franchise. (Hint: Use the formula of the present value
dollars/year. If the prevailing interest rate is 10% / year compounded continuously, find the present value of the franchise. (Hint: Use the formula of the present value 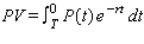 .) Please round the answer to the nearest cent.
.) Please round the answer to the nearest cent.
(Multiple Choice)
4.8/5  (36)
(36)
Determine whether the given function is a probability density function on the specified interval. 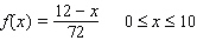
(Multiple Choice)
4.9/5  (33)
(33)
Determine whether the given function is a probability density function on the specified interval. 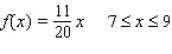
(Essay)
4.8/5  (33)
(33)
Evaluate the following improper integral whenever it is convergent. 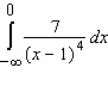 Enter divergent if the integral is divergent.
Enter divergent if the integral is divergent.
(Essay)
4.8/5  (36)
(36)
Determine whether the given function is a probability density function on the specified interval. 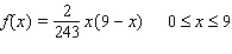
(Essay)
4.8/5  (36)
(36)
Determine whether the statement is true.
If 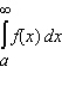 exists, then
exists, then 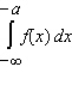 exists and
exists and 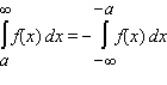 .
Answer yes or no.
.
Answer yes or no.
(Essay)
4.9/5  (39)
(39)
Use the table of integrals to find the integral. 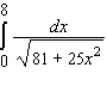 Use C as the constant of integration.
Use C as the constant of integration.
(Essay)
5.0/5  (31)
(31)
Use the trapezoidal rule and Simpson's rule to approximate the value of the definite integral. 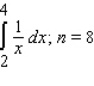
(Multiple Choice)
4.9/5  (37)
(37)
Use the trapezoidal rule and Simpson's rule to approximate the value of the definite integral. Please give the answer to four decimal places. 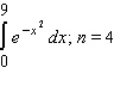 Trapezoidal rule: __________
Simpson's rule: __________
Trapezoidal rule: __________
Simpson's rule: __________
(Essay)
4.9/5  (35)
(35)
Evaluate the following improper integral whenever it is convergent. 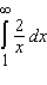 Enter divergent if the integral is divergent.
Enter divergent if the integral is divergent.
(Essay)
4.8/5  (31)
(31)
The revenue of Virtual Reality, a video-game arcade, is generated at the rate of 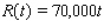 dollars. If the revenue is invested t years from now in a business earning interest at the rate of 18% / year compounded continuously, find the accumulated value of this stream of income at the end of 2 years . Round to the nearest dollar.
$__________
dollars. If the revenue is invested t years from now in a business earning interest at the rate of 18% / year compounded continuously, find the accumulated value of this stream of income at the end of 2 years . Round to the nearest dollar.
$__________
(Short Answer)
4.8/5  (35)
(35)
National Welding uses industrial robots in some of its assembly-line operations. Management has determined that, on average, a robot breaks down after 1,000 hours of use and that the lengths of time between breakdowns are exponentially distributed.
a. What is the probability that a robot selected at random will break down between 300 and 600 hours of use?
Round your answer to two decimal places.
b. What is the probability that a robot will break down after 1,100 hours of use?
Round your answer to two decimal places.
(Essay)
4.7/5  (35)
(35)
Evaluate the following improper integral whenever it is convergent. 
(Multiple Choice)
4.8/5  (34)
(34)
Showing 121 - 140 of 250
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)