Exam 7: Additional Topics in Integration
Exam 1: Preliminaries205 Questions
Exam 2: Functions, Limits and the Derivative269 Questions
Exam 3: Differentiation330 Questions
Exam 4: Applications of the Derivative182 Questions
Exam 5: Exponential and Logarithmic Functions278 Questions
Exam 6: Integration314 Questions
Exam 7: Additional Topics in Integration250 Questions
Exam 8: Calculus of Several Variables206 Questions
Select questions type
The quantity demanded x (in thousands of pounds) of a certain brand of butter each week is a continuous random variable with probability density function 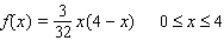 What is the expected demand for this brand of butter each week?
__________ lb
What is the expected demand for this brand of butter each week?
__________ lb
(Short Answer)
4.7/5  (33)
(33)
A manmade lake located in Lake View Condominiums has the shape depicted in the following figure. The measurements shown were taken at 10-foot intervals. Using Simpson's rule with  , estimate the surface area of the lake. Round the result to the nearest tenth.
, estimate the surface area of the lake. Round the result to the nearest tenth.  __________
__________ 
(Short Answer)
4.7/5  (36)
(36)
Evaluate the following improper integral whenever it is convergent. 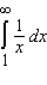
(Multiple Choice)
4.8/5  (43)
(43)
Evaluate the definite integral by using the method of integration by parts. 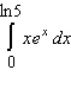
(Essay)
4.8/5  (32)
(32)
Evaluate the following improper integral whenever it is convergent. 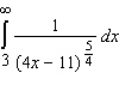
(Multiple Choice)
4.7/5  (41)
(41)
In a study conducted by a certain country's Economic Development Board regarding the income distribution of certain segments of the country's workforce, it was found that the Lorentz curve for the distribution of income of college professors is described by the function 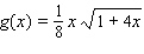 Compute the coefficient of inequality of the Lorentz curve. Round to the nearest thousandth. Hint: Use the formula
Compute the coefficient of inequality of the Lorentz curve. Round to the nearest thousandth. Hint: Use the formula 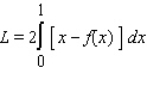 .
.
(Multiple Choice)
4.9/5  (32)
(32)
Determine whether the given function is a probability density function on the specified interval. 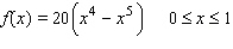
(Multiple Choice)
4.9/5  (35)
(35)
Find the area of the region under the given curve 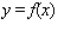 over the indicated interval.
over the indicated interval. 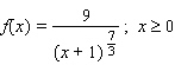
(Multiple Choice)
4.8/5  (40)
(40)
In a submerged trial run of an attack submarine, a reading of the submarine's velocity was made every quarter hour, as shown in the accompanying table. Use the trapezoidal rule to estimate the distance traveled by the submarine during the 2-hour period. Please round the answer to the nearest hundredth if necessary. 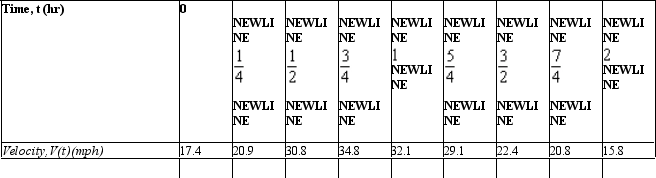
(Multiple Choice)
4.7/5  (37)
(37)
Determine the value of the constant 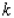 so that the function
so that the function  is a probability density function on the interval
is a probability density function on the interval 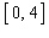 . If
. If 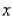 is a continuous random variable, compute the probability that
is a continuous random variable, compute the probability that 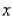 will assume a value between
will assume a value between 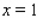 and
and 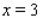 .
.
(Multiple Choice)
4.8/5  (34)
(34)
Determine the value of the constant k so that the function 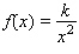 is a probability density function on the interval
is a probability density function on the interval 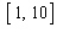 .
.
(Multiple Choice)
4.9/5  (45)
(45)
The number of streets in the downtown section of a certain city that need repairs in a given year is a random variable with a distribution described by the probability density function 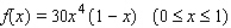 Find the probability that at most half of the streets will need repairs in any given year.
Find the probability that at most half of the streets will need repairs in any given year.
(Multiple Choice)
4.9/5  (41)
(41)
The amount of nitrogen dioxide, a brown gas that impairs breathing, present in the atmosphere on a certain May day in a city has been approximated by 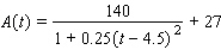 where
where 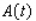 is measured in pollutant standard index (PSI), and
is measured in pollutant standard index (PSI), and 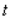 is measured in hours, with
is measured in hours, with 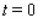 corresponding to 7 a.m. Use the trapezoidal rule with
corresponding to 7 a.m. Use the trapezoidal rule with 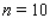 to estimate the average PSI between 7 a.m. and noon. Please round the answer to the nearest hundredth. (Hint:
to estimate the average PSI between 7 a.m. and noon. Please round the answer to the nearest hundredth. (Hint: 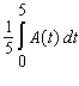 .)
.)
(Multiple Choice)
4.8/5  (34)
(34)
Evaluate the following improper integral whenever it is convergent. 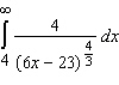
(Short Answer)
4.9/5  (29)
(29)
Showing 221 - 240 of 250
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)