Exam 4: Linear Programming
Exam 1: Urban Services107 Questions
Exam 2: Business Efficiency104 Questions
Exam 3: Planning and Scheduling108 Questions
Exam 4: Linear Programming111 Questions
Exam 5: Exploring Data: Distributions115 Questions
Exam 6: Exploring Data: Relationships104 Questions
Exam 7: Data for Decisions99 Questions
Exam 8: Probability: the Mathematics of Chance108 Questions
Exam 9: Social Choice: the Impossible Dream103 Questions
Exam 10: The Manipulability of Voting Systems106 Questions
Exam 11: Weighted Voting Systems111 Questions
Exam 12: Electing the President93 Questions
Exam 13: Fair Division121 Questions
Exam 14: Apportionment112 Questions
Exam 15: Game Theory: the Mathematics of Competition113 Questions
Exam 16: Identification Numbers110 Questions
Exam 17: Information Science94 Questions
Exam 18: Growth and Form111 Questions
Exam 19: Symmetry and Patterns115 Questions
Exam 20: Tilings112 Questions
Exam 21: Savings Models113 Questions
Exam 22: Borrowing Models113 Questions
Exam 23: The Economics of Resources119 Questions
Select questions type
Given below is the sketch of the feasible region in a linear programming problem. Which point is NOT in the feasible region? 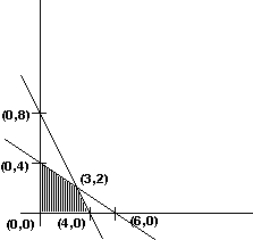
(Multiple Choice)
4.8/5  (29)
(29)
Explain why the feasible region for a linear programming mixture problem must be in the first quadrant of the Cartesian plane.
(Essay)
4.8/5  (35)
(35)
Find the point of intersection for the lines represented by the equations 3x + 2y = 16 and 4x + 3y = 23.
(Short Answer)
4.7/5  (44)
(44)
Use the following to answer the Questions:
concern the following tableau for a shipping problem. 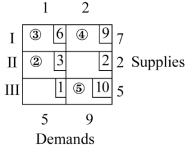 -What is the indicator value of cell (III, 1)?
-What is the indicator value of cell (III, 1)?
(Essay)
4.7/5  (36)
(36)
The simplex algorithm always gives optimal solutions to linear programming problems.
(True/False)
4.8/5  (32)
(32)
The graph of the feasible region for a mixture problem is shown below. Find the point that maximizes the profit function P = 3x + 6y. 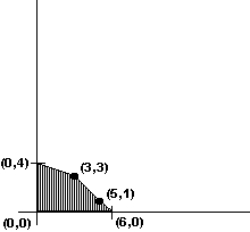
(Multiple Choice)
4.7/5  (38)
(38)
Given below is the sketch of the feasible region in a linear programming problem. Which point is NOT in the feasible region? 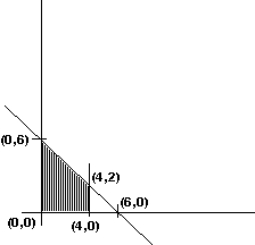
(Multiple Choice)
4.8/5  (33)
(33)
Solve this linear programming mixture problem: A small stereo manufacturer makes a receiver and a CD player. Each receiver takes eight hours to assemble, one hour to test and ship, and earns a profit of $30. Each CD player takes 15 hours to assemble, two hours to test and ship, and earns a profit of $50. There are 160 hours available in the assembly department and 26 hours available in the testing and shipping department. What should the production schedule be to maximize profit?
(Essay)
4.9/5  (38)
(38)
Find the point of intersection of the lines with equations 2x + 5y = 6 and 3x + 2y = 9.
(Multiple Choice)
4.8/5  (34)
(34)
Consider the feasible region identified by the inequalities below. x 0; y 0; x + y 4; x + 3y 6 Which point is NOT a corner of the region?
(Multiple Choice)
4.8/5  (40)
(40)
Explain what the real world implications are if the optimal production policy for a linear programming mixture problem is represented by a point on the x-axis of the Cartesian plane.
(Essay)
5.0/5  (41)
(41)
With the given constraints for the following linear programming mixture problem, graph the feasible region.
2 x + 3y 1800
x 0
y 0
(Essay)
4.8/5  (34)
(34)
Use the following tableau to answer the questions:
concern the following tableau for a shipping problem. 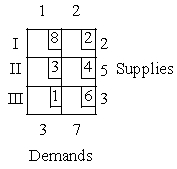 -In the tableau produced by the Northwest Corner Rule, what is the indicator value of cell (I, 2)?
-In the tableau produced by the Northwest Corner Rule, what is the indicator value of cell (I, 2)?
(Multiple Choice)
4.9/5  (30)
(30)
Graph the constraint inequalities for a linear programming problem shown below. Which feasible region shown is correct? 6x+4y\leq12 x\geq0,y\geq0
(Multiple Choice)
4.9/5  (25)
(25)
Graph the constraint inequalities for a linear programming problem shown below. Which feasible region shown is correct? 4x+3y\leq24 x\geq0,y\geq0
(Multiple Choice)
4.8/5  (28)
(28)
Write the constraint inequalities for this situation: Kim and Lynn produce pottery vases and bowls. A vase requires 35 oz of clay and 5 oz of glaze. A bowl requires 20 oz of clay and 10 oz of glaze. There are 500 oz of clay available and 200 oz of glaze available. The profit on one vase is $5 and the profit on one bowl is $4.
(Multiple Choice)
4.8/5  (30)
(30)
Find the point of intersection of the lines with equations 4x + 2y = 12 and 3x + 9y = 39.
(Multiple Choice)
4.9/5  (43)
(43)
Showing 21 - 40 of 111
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)