Exam 4: Sensitivity Analysis and the Simplex Method
Exam 1: Introduction to Modeling and Decision Analysis78 Questions
Exam 2: Introduction to Optimization and Linear Programming76 Questions
Exam 3: Modeling and Solving Lp Problems in a Spreadsheet93 Questions
Exam 4: Sensitivity Analysis and the Simplex Method76 Questions
Exam 5: Network Modeling92 Questions
Exam 6: Integer Linear Programming102 Questions
Exam 7: Goal Programming and Multiple Objective Optimization81 Questions
Exam 8: Nonlinear Programming Evolutionary Optimization87 Questions
Exam 9: Regression Analysis93 Questions
Exam 10: Data Mining125 Questions
Exam 11: Time Series Forecasting125 Questions
Exam 12: Introduction to Simulation Using Analytic Solver Platform85 Questions
Exam 13: Queuing Theory97 Questions
Exam 14: Decision Analysis125 Questions
Exam 15: Project Management Online82 Questions
Select questions type
When a manager considers the effect of changes in an LP model's coefficients he/she is performing
(Multiple Choice)
4.8/5  (37)
(37)
Given the following Analytic Solver Platform sensitivity output what range of values can the objective function coefficient for variable X1 assume without changing the optimal solution? 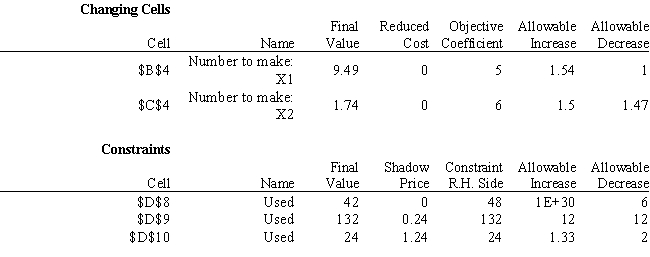
(Short Answer)
4.8/5  (27)
(27)
Which of the constraints are binding at the optimal solution for the following problem and Analytic Solver Platform sensitivity output? 
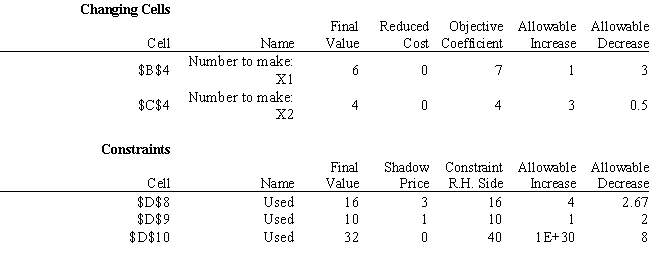
(Essay)
4.7/5  (30)
(30)
A formulation has 20 variables and 8 constraints (not counting non-negativity). How many variables are nonbasic?
(Multiple Choice)
4.8/5  (41)
(41)
Given an objective function value of 150 and a shadow price for resource 1 of 5, if 10 more units of resource 1 are added (assuming the allowable increase is greater than 10), what is the impact on the objective function value?
(Multiple Choice)
4.8/5  (30)
(30)
Exhibit 4.2
The following questions correspond to the problem below and associated Analytic Solver Platform sensitivity report.
Robert Hope received a welcome surprise in this management science class; the instructor has decided to let each person define the percentage contribution to their grade for each of the graded instruments used in the class. These instruments were: homework, an individual project, a mid-term exam, and a final exam. Robert's grades on these instruments were 75, 94, 85, and 92, respectively. However, the instructor complicated Robert's task somewhat by adding the following stipulations: 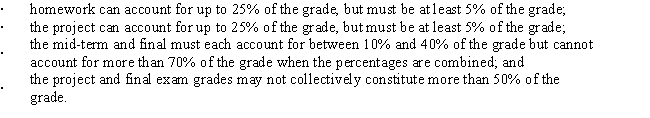 The following LP model allows Robert to maximize his numerical grade.
The following LP model allows Robert to maximize his numerical grade. 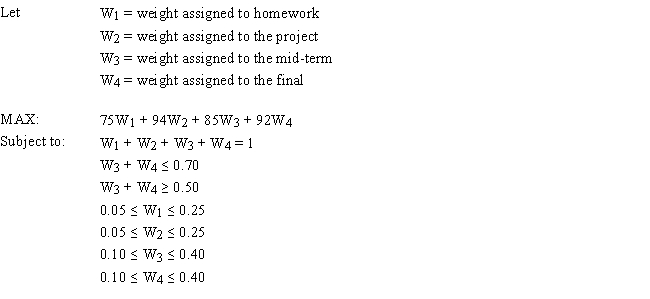
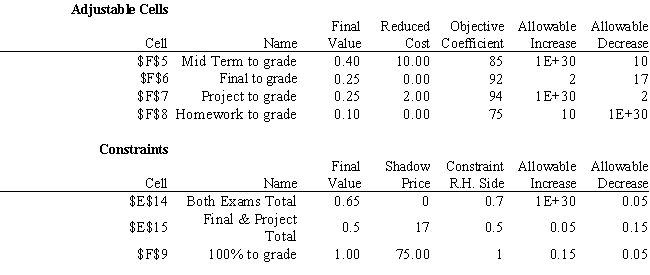 -Refer to Exhibit 4.2. Constraint cell F9 corresponds to the constraint, W1 + W2 + W3 + W4 = 1, and has a shadow price of 75. Armed with this information, what can Robert request of his instructor regarding this constraint?
-Refer to Exhibit 4.2. Constraint cell F9 corresponds to the constraint, W1 + W2 + W3 + W4 = 1, and has a shadow price of 75. Armed with this information, what can Robert request of his instructor regarding this constraint?
(Essay)
4.8/5  (36)
(36)
Identify the different sets of basic variables that might be used to obtain a solution to this problem. 
(Essay)
4.8/5  (33)
(33)
When a solution is degenerate the shadow prices and their ranges
(Multiple Choice)
4.8/5  (35)
(35)
What is the value of the objective function if X1 is set to 0 in the following Limits Report? 
(Multiple Choice)
5.0/5  (32)
(32)
A solution to the system of equations using a set of basic variables is called
(Multiple Choice)
4.9/5  (32)
(32)
What is the smallest value of the objective function coefficient X1 can assume without changing the optimal solution? 

(Essay)
4.8/5  (40)
(40)
If the shadow price for a resource is 0 and 150 units of the resource are added what happens to the objective function value?
(Multiple Choice)
4.9/5  (36)
(36)
Analytic Solver Platform provides all of the following reports except
(Multiple Choice)
4.9/5  (37)
(37)
Identify the different sets of basic variables that might be used to obtain a solution to this problem. 
(Essay)
4.8/5  (27)
(27)
What needs to be done to the two constraints in order to convert the problem to a standard form? 
(Multiple Choice)
4.9/5  (38)
(38)
When a solution is degenerate the reduced costs for the changing cells
(Multiple Choice)
4.7/5  (31)
(31)
Given the following Analytic Solver Platform sensitivity output how much does the objective function coefficient for X2 have to increase before it enters the optimal solution at a strictly positive value? 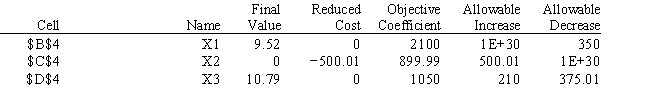
(Short Answer)
4.9/5  (31)
(31)
For a minimization problem, if a decision variable's final value is 0, and its reduced cost is negative, which of the following is true?
(Multiple Choice)
4.8/5  (35)
(35)
Which of the following statements is false concerning either of the Allowable Increase and Allowable Decrease columns in the Sensitivity Report?
(Multiple Choice)
4.8/5  (45)
(45)
Showing 21 - 40 of 76
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)