Exam 3: Vector
Exam 1: Measurement37 Questions
Exam 2: Motion Along a Straight Line90 Questions
Exam 3: Vector37 Questions
Exam 4: Motion in Two and Three Dimensions56 Questions
Exam 5: Force and Motion I73 Questions
Exam 6: Force and Motion II74 Questions
Exam 7: Kinetic Energy and Work73 Questions
Exam 8: Potential Energy and Conservation of Energy63 Questions
Exam 9: Center of Mass and Linear Momentum99 Questions
Exam 10: Rotation102 Questions
Exam 11: Rolling, Torque, and Angular Momentum66 Questions
Exam 12: Equilibrium and Elasticity57 Questions
Exam 13: Gravitation55 Questions
Exam 14: Fluids88 Questions
Exam 15: Oscillations75 Questions
Exam 16: Waves I82 Questions
Exam 17: Waves II71 Questions
Exam 18: Temperature, Heat, and the First Law of Thermodynamics96 Questions
Exam 19: The Kinetic Theory of Gases113 Questions
Exam 20: Entropy and the Second Law of Thermodynamics61 Questions
Exam 21: Electric Charge52 Questions
Exam 22: Electric Fields55 Questions
Exam 23: Gauss Law38 Questions
Exam 24: Electric Potential52 Questions
Exam 25: Capacitance61 Questions
Exam 26: Current and Resistance55 Questions
Exam 27: Circuits73 Questions
Exam 28: Magnetic Fields55 Questions
Exam 29: Magnetic Fields Due to Currents49 Questions
Exam 30: Induction and Inductance90 Questions
Exam 31: Electromagnetic Oscillations and Alternating Current88 Questions
Exam 32: Maxwells Equations; Magnetism of Matter81 Questions
Exam 33: Electromagnetic Waves83 Questions
Exam 34: Images79 Questions
Exam 35: Interference46 Questions
Exam 36: Diffraction77 Questions
Exam 37: Relativity68 Questions
Exam 38: Photons and Matter Waves57 Questions
Exam 39: More About Matter Waves41 Questions
Exam 40: All About Atoms79 Questions
Exam 41: Conduction of Electricity in Solids51 Questions
Exam 42: Nuclear Physics68 Questions
Exam 43: Energy From the Nucleus50 Questions
Exam 44: Quarks, Leptons, and the Big Bang55 Questions
Select questions type
Two vectors lie with their tails at the same point. When the angle between them is increased by 20 their scalar product has the same magnitude but changes from positive to negative. The original angle between them was:
(Multiple Choice)
4.8/5  (27)
(27)
A vector of magnitude 6 and another vector have a resultant of magnitude 12. The vector :
(Multiple Choice)
4.8/5  (37)
(37)
Let = (2 m) + (6 m) - (3 m) and = (4 m) + (2 m) + (1 m). The vector sum is:
(Multiple Choice)
4.8/5  (32)
(32)
Let = (1 m) + (2 m) + (2 m) and = (3 m) + (4 m). The angle between these two vectors is given by:
(Multiple Choice)
4.7/5  (34)
(34)
Two vectors lie with their tails at the same point. When the angle between them is increased by 20 the magnitude of their vector product doubles. The original angle between them was about:
(Multiple Choice)
4.8/5  (23)
(23)
Four vectors 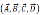 all have the same magnitude. The angle between adjacent vectors is 45 as shown. The correct vector equation is:
all have the same magnitude. The angle between adjacent vectors is 45 as shown. The correct vector equation is: 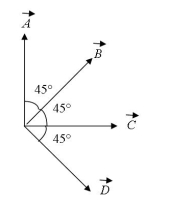
(Multiple Choice)
4.7/5  (26)
(26)
Two vectors have magnitudes of 10 and 15. The angle between them when they are drawn with their tails at the same point is 65 . The component of the longer vector along the line of the shorter is:
(Multiple Choice)
4.8/5  (38)
(38)
If the magnitude of the sum of two vectors is greater than the magnitude of either vector, then:
(Multiple Choice)
4.8/5  (30)
(30)
A vector of magnitude 20 is added to a vector of magnitude 25. The magnitude of this sum can be:
(Multiple Choice)
4.8/5  (33)
(33)
A vector has a magnitude of 12. When its tail is at the origin it lies between the positive x axis and negative y axis and makes an angle of 30 with the x axis. Its y component is:
(Multiple Choice)
4.9/5  (43)
(43)
If the magnitude of the sum of two vectors is less than the magnitude of either vector, then:
(Multiple Choice)
4.8/5  (34)
(34)
The two vectors (3 m) − (7 m) and (2 m) + (3 m) − (2 m) define a plane (it is the plane of the triangle with both tails at one vertex and each head at one of the other vertices). Which of the following vectors is perpendicular to the plane?
(Multiple Choice)
4.9/5  (30)
(30)
The angle between = (25 m) + (45 m) and the positive x axis is:
(Multiple Choice)
4.8/5  (37)
(37)
Showing 21 - 37 of 37
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)