Essay
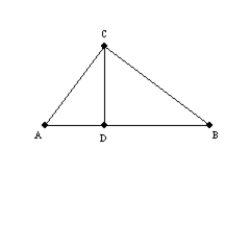
-Supply missing statements and missing reasons for the proof of this theorem.
"The altitude drawn to the hypotenuse of a right triangle separates the right triangle into two
right triangles that are similar to each other."
Given: Right triangle ABC with rt. 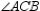 ;
; 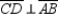 Prove:
Prove: 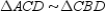 S1. R1.
S1. R1.
S2. 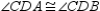 R2.
R2.
S3.  and
and 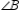 are comp. R3. The acute angles of a rt.
are comp. R3. The acute angles of a rt. 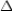 are comp.
are comp.
S4.  and
and 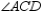 are comp. R4.
are comp. R4.
S5. R5. If 2  s are comp. to the same
s are comp. to the same  , these
, these  are
are 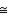 .
.
S6. R6.
Correct Answer:

Verified
S1. Right triangle ABC with rt...View Answer
Unlock this answer now
Get Access to more Verified Answers free of charge
Correct Answer:
Verified
View Answer
Unlock this answer now
Get Access to more Verified Answers free of charge
Q2: Where <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB7237/.jpg" alt="Where and
Q3: <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB7237/.jpg" alt=" -Supply missing statements
Q4: <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB7237/.jpg" alt=" -Supply the missing
Q5: Explain (prove) the following property of proportions.<br>"If
Q6: <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB7237/.jpg" alt=" -Supply missing statements
Q7: <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB7237/.jpg" alt=" -Provide all statements
Q8: <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB7237/.jpg" alt=" -Use the drawing
Q9: <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB7237/.jpg" alt=" -Use the drawing(s)
Q10: <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB7237/.jpg" alt=" -Provide the missing
Q11: <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB7237/.jpg" alt=" -Supply missing statements