Find the Particular Solution of the Differential Equation $\frac { w } { g } y ^ { tt } ( t ) + b y ^ { t } ( t ) + k y ( t ) = \frac { w } { g } F ( t )$

Multiple Choice

Find the particular solution of the differential equation $\frac { w } { g } y ^ { tt } ( t ) + b y ^ { t } ( t ) + k y ( t ) = \frac { w } { g } F ( t )$ for the oscillating motion of an object on the end of a spring. In the equation, $y$ is the displacement from equilibrium (positive direction is downward) measured in feet, and $t$ is the time in seconds (see figure) . The constant $w = 32$ is the weight of the object, $g = 72$ is the acceleration due to gravity, $b = 0$ is the magnitude of the resistance to the motion, $k = 64$ is the spring constant from Hooke's Law, $F ( t ) = 64 \sin 6 t$ is the acceleration imposed on the system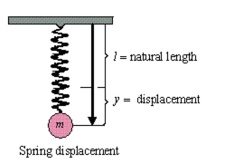, $y ( 0 ) = \frac { 1 } { 6 }$ and $y ^ { t } ( 0 ) = 0$

A) $y = \frac { 1 } { 6 } \cos 12 t - \frac { 8 } { 27 } \sin 12 t + \frac { 14 } { 27 } \sin 6 t$
B) $y = \frac { 1 } { 12 } \cos 12 t - \frac { 16 } { 63 } \sin 12 t + \frac { 14 } { 27 } \cos 6 t$
C) $y = \frac { 1 } { 12 } \cos 12 t - \frac { 16 } { 63 } \sin 12 t - \frac { 14 } { 27 } \sin 6 t$
D) $y = \frac { 1 } { 6 } \cos 12 t - \frac { 8 } { 27 } \sin 12 t + \frac { 16 } { 27 } \cos 6 t$
E) $y = \frac { 1 } { 6 } \cos 12 t - \frac { 8 } { 27 } \sin 12 t + \frac { 16 } { 27 } \sin 6 t$