Multiple Choice
The following data show the cooling temperatures of a freshly brewed cup of coffee after it is poured from the brewing pot into a serving cup. The brewing pot temperature is approximately 180º F. 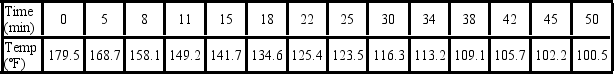 The output for an exponential model, ln(Temp) = β0 + β1Time + ε, is below.
The output for an exponential model, ln(Temp) = β0 + β1Time + ε, is below. 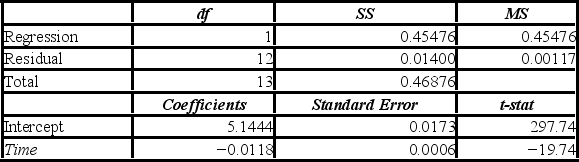 Which of the following is the regression equation for making predictions concerning the coffee temperature?
Which of the following is the regression equation for making predictions concerning the coffee temperature?
A) 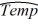 = exp (5.1444 - 0.0118Time
= exp (5.1444 - 0.0118Time
B) 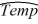 = exp (5.1450 - 0.0118Time
= exp (5.1450 - 0.0118Time
C) 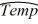 = 5.1444 - 0.0118Time
= 5.1444 - 0.0118Time
D) 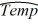 = 5.1450 - 0.0118Time
= 5.1450 - 0.0118Time
Correct Answer:

Verified
Correct Answer:
Verified
Q29: The following data show the cooling temperatures
Q30: The scatterplot shown below represents a typical
Q31: The following data show the cooling temperatures
Q32: Thirty employed single individuals were randomly selected
Q33: The following data show the demand for
Q35: Typically, the sales volume declines with an
Q36: The quadratic and logarithmic models, y =
Q37: The following data show the cooling temperatures
Q38: The following data show the cooling temperatures
Q39: The following data show the demand for