Exam 17: The Nature of Voting and Apportionment
Exam 1: The Nature of Problem Solving53 Questions
Exam 2: The Nature of Sets64 Questions
Exam 3: The Nature of Logic160 Questions
Exam 4: The Nature of Numeration Systems102 Questions
Exam 5: The Nature of Numbers139 Questions
Exam 6: The Nature of Algebra173 Questions
Exam 7: The Nature of Geometry139 Questions
Exam 8: The Nature of Measurement50 Questions
Exam 9: The Nature of Networks and Graph Theory76 Questions
Exam 10: The Nature of Growth57 Questions
Exam 11: The Nature of Sequences, Series, and Financial Management130 Questions
Exam 12: The Nature of Counting78 Questions
Exam 13: The Nature of Probability97 Questions
Exam 14: The Nature of Statistics82 Questions
Exam 15: The Nature of Graphs and Functions74 Questions
Exam 16: The Nature of Mathematical Systems97 Questions
Exam 17: The Nature of Voting and Apportionment52 Questions
Select questions type
An elderly rancher died and left her estate to her three children. She bequeathed her 17 prize horses in the following manner: 1/2 to the eldest, 1/3 to the second child, and 1/9 to the youngest.
The children decided to call in a very wise judge to help in the distribution of the rancher's estate. They informed the judge that the 17 horses were not of equal value. The children agreed on a ranking of the 17 horses (#1 being the best and #17 being a real dog of a horse). They asked the judge to divide the estate fairly so that each child would receive not only the correct number of horses but horses whose average rank would also be the same. For example, if a child received horses 1 and 17, the number of horses is two and the average value is 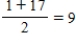 . How did the judge apportion the horses?
. How did the judge apportion the horses?
Free
(Multiple Choice)
4.8/5  (38)
(38)
Correct Answer:
D
Use Hamilton's plan to apportion the new seats to the existing states. Then increase the number of seats by one and decide whether the Alabama paradox occurs. Assume that the populations are in thousands.  Number of seats:
Number of seats:  86
86
Free
(Multiple Choice)
4.8/5  (34)
(34)
Correct Answer:
D
A group of fun-loving people have decided to play a practical joke on one of their friends, but they can't decide which friend, Alice (A), Betty (B), or Connie (C). Their preferences are: 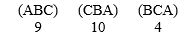
Who wins the election using the Hare method? Answer Alice, Betty, or Connie.
Does this violate the Condorcet criterion? Answer yes or no.
Who wins the election using the Hare method? Answer Alice, Betty, or Connie.
Does this violate the Condorcet criterion? Answer yes or no.
Free
(Short Answer)
4.8/5  (28)
(28)
Correct Answer:
Connie; Yes
A group of fun-loving people have decided to play a practical joke on one of their friends, but they can't decide which friend, Alice (A), Betty (B), or Connie (C). Their preferences are:  Who wins the election using the Hare method? Does this violate the Condorcet criterion?
Who wins the election using the Hare method? Does this violate the Condorcet criterion?
(Multiple Choice)
4.8/5  (39)
(39)
Use Jefferson's plan. Which state does violate the quota rule?  Number of seats:
Number of seats:  200
__________ (A, B, C, D, none)
200
__________ (A, B, C, D, none)
(Short Answer)
4.8/5  (31)
(31)
The fraternity is electing a national president and there are four candidates: Alberto (A), Bate (B), Carl (C), and Dave (D). The voter preferences are: 
Who wins the election using the Hare method? Answer Alberto, Bate, Carl, or Dave.
Does this violate any of the fairness criteria? Answer Yes. Irrelevant alternative criterion.; Yes. Condition of decisiveness.; Yes. Condorcet criterion.; or No.
Who wins the election using the Hare method? Answer Alberto, Bate, Carl, or Dave.
Does this violate any of the fairness criteria? Answer Yes. Irrelevant alternative criterion.; Yes. Condition of decisiveness.; Yes. Condorcet criterion.; or No.
(Short Answer)
4.8/5  (31)
(31)
Consider an election with three candidates with the results: 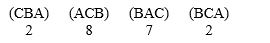 Who wins the election using the Borda count method?
Who wins the election using the Borda count method?
(Multiple Choice)
5.0/5  (32)
(32)
Apportion the indicated number of representatives to two states, A, and B, using Hamilton's plan. Next, recalculate the apportionment using Hamilton's plan for the three states, C and the original states. Decide whether the new states paradox occurs. 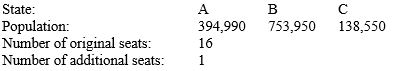
__________ (A illustrates the new states paradox.; B illustrates the new states paradox.; C illustrates the new states paradox.; A and B illustrate the new states paradox.; The paradox does not occur.)
__________ (A illustrates the new states paradox.; B illustrates the new states paradox.; C illustrates the new states paradox.; A and B illustrate the new states paradox.; The paradox does not occur.)
(Short Answer)
4.8/5  (36)
(36)
A fair apportionment of dividing a leftover piece of cake between two children is to let child #1 cut the cake into two pieces and then to let child #2 pick which piece he or she wants. Consider the following apportionment of dividing the leftover piece of cake among three children. Let the first child cut the cake into two pieces. Then the second child is permitted to cut one of those pieces into two parts. Child #3 can select any of the pieces, followed by child #1 selecting one of the remaining pieces, followed by child #2 who gets the remaining piece. Is this allocation process fair if each child's goal is to maximize the size of his or her own piece of cake?
__________ (Yes, No)
(Short Answer)
4.9/5  (43)
(43)
Suppose the annual salaries of three people are: 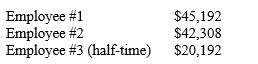 What are their salaries if they are given a 4% raise, and then the result is rounded to the nearest $1,000 using Hamilton's plan with a cap on the total salaries of $113,000?
What are their salaries if they are given a 4% raise, and then the result is rounded to the nearest $1,000 using Hamilton's plan with a cap on the total salaries of $113,000?
(Multiple Choice)
4.8/5  (35)
(35)
Consider an election with three candidates with the results: 
Is there a majority winner? If not, who is the plurality winner? Answer No, B; Yes, C; No, C; No, A; or Yes, B
Who wins using the pairwise comparison method? Answer A, C, or
B.
Is the ordering for the choices for candidates in the previous question transitive? Answer yes or no.
Is there a majority winner? If not, who is the plurality winner? Answer No, B; Yes, C; No, C; No, A; or Yes, B
Who wins using the pairwise comparison method? Answer A, C, or
B.
Is the ordering for the choices for candidates in the previous question transitive? Answer yes or no.
(Short Answer)
4.8/5  (39)
(39)
Use Jefferson's plan. Which state does violate the quota rule? 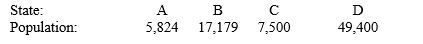 Number of seats:
Number of seats:  200
200
(Multiple Choice)
4.8/5  (36)
(36)
A focus group of 41 people for ABC TV were asked to rank the government spending priorities of education (E), military spending (M), health care (H), immigration (I), and lowering taxes (T). Here are the preferences: 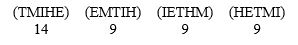
Suppose that the losing issues of immigration, health care, and military spending are removed from the table. Now, who is the winner using the Borda count method?
Suppose that the losing issues of immigration, health care, and military spending are removed from the table. Now, who is the winner using the Borda count method?
(Multiple Choice)
4.9/5  (36)
(36)
The race for the governor of Vermont, suppose the state vote was as follows: 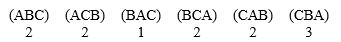
Was there a majority winner in this election, and if so, who was it?
Was there a majority winner in this election, and if so, who was it?
(Multiple Choice)
4.9/5  (28)
(28)
Round the given modified quota
3)57
By comparing it first with the arithmetic mean, and then with the geometric mean of the lower and upper quotas.
(Multiple Choice)
4.7/5  (35)
(35)
Twelve people serve on a board and are considering three alternatives A, B, and C. Here are the choices followed by vote: 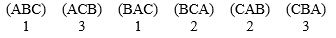 Determine the winner using Hare method.
Answer A, B, C, or none of them.
Determine the winner using Hare method.
Answer A, B, C, or none of them.
(Short Answer)
4.9/5  (37)
(37)
The seniors at a high school are voting for where to go for their senior trip. They are deciding on Angel Falls (A), Bear Valley (B), Clear Canyon (C), or Dragon Cave (D). The  Determine who wins using the pairwise comparison method.
Determine who wins using the pairwise comparison method.
(Multiple Choice)
4.9/5  (37)
(37)
The Adobe School District is hiring a vice principal and has interviewed four candidates: Alicia (A), Ben (B), Carmelia (C), and Diana (D). The hiring committee has indicated their preferences. 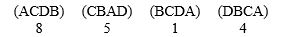 Determine who is the winner using the plurality method. Then suppose that Diana drops out of the running before the vote is taken. Do the results you obtain violate the irrelevant alternatives criterion?
Determine who is the winner using the plurality method. Then suppose that Diana drops out of the running before the vote is taken. Do the results you obtain violate the irrelevant alternatives criterion?
(Multiple Choice)
4.7/5  (35)
(35)
Consider an election with three candidates with the results: 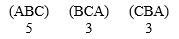 Who wins using the pairwise comparison method?
Who wins using the pairwise comparison method?
(Multiple Choice)
4.8/5  (29)
(29)
In voting among four candidates, the outcomes are reported as: 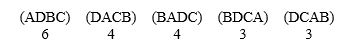
What does the notation (BADC) mean?
"(BADC)" means that the voter ranks three candidates in the order of __________ first, __________ second, __________ third, and candidate __________ last.
What does the notation (BADC) mean?
"(BADC)" means that the voter ranks three candidates in the order of __________ first, __________ second, __________ third, and candidate __________ last.
(Short Answer)
4.8/5  (34)
(34)
Showing 1 - 20 of 52
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)