Exam 6: Applications of the Definite Integral in Geometry, Science and Engineering
Exam 1: Limits and Continuity186 Questions
Exam 2: The Derivative198 Questions
Exam 3: Topics in Deifferentiation171 Questions
Exam 4: The Derivative in Graphing and Applications656 Questions
Exam 5: Integration323 Questions
Exam 6: Applications of the Definite Integral in Geometry, Science and Engineering314 Questions
Exam 7: Principle of Integral Evaluation269 Questions
Exam 8: Mathematical Modeling With Differential Equations77 Questions
Exam 9: Infinte Series288 Questions
Exam 10: Parametric and Polar Curves; Conic Sections199 Questions
Exam 11: Three-Dimensional Space; Vectors173 Questions
Exam 12: Vector-Valued Functions147 Questions
Exam 13: Partial Derivatives194 Questions
Exam 14: Multiple Integrals117 Questions
Exam 15: Topics in Vector Calculus149 Questions
Select questions type
Use a graphing utility to find the area of the region enclosed by the curves x = sin 4 y, x = 0, y = 0, y = - /4.
(Short Answer)
4.8/5  (27)
(27)
A spring whose natural length is 18 inches exerts a force of 7.2 pounds when stretched to 26 inches. How much work is required to stretch the spring 16 inches beyond its natural length?
(Essay)
4.8/5  (33)
(33)
Find the value of the solid that is obtained when the region enclosed by the curves x = y3 , x = 0, y = 1, y = 3 is revolved about the y-axis.
(Multiple Choice)
4.7/5  (36)
(36)
Find the centroid of the region enclosed by y = -x2 + 8 and 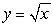 from x = 0 to x = 1.
from x = 0 to x = 1.
(Essay)
4.9/5  (38)
(38)
Use the method of disks to find the volume of the solid that results by revolving the region enclosed by the curves y = cos6x, x = 0, 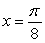 about the x-axis. Approximate area to three decimal places.
about the x-axis. Approximate area to three decimal places.
(Multiple Choice)
4.7/5  (35)
(35)
Find the area of the surface that is generated by revolving the portion of the curve y = x2 - 2 between x = 0 and x = 1 about the x-axis. Approximate area to three decimal places.
(Essay)
4.9/5  (22)
(22)
A horizontal cylindrical tank of diameter 12 feet is half full of a chemical (weight density 55 lbs/ft3). Calculate the force against one end.
(Essay)
4.9/5  (44)
(44)
Let a hemisphere of radius 5 be cut by a plane parallel to the base of the hemisphere thus forming a segment of height 1 (in the top portion of the hemisphere). Find its volume using cylindrical shells.
(Essay)
4.9/5  (39)
(39)
Assume 10 J of work stretch a spring 2 cm. Find the spring constant in J/cm.
(Multiple Choice)
4.9/5  (35)
(35)
The steeple of a church is constructed in the form of a pyramid 45 feet high. The cross sections are all squares and the base is a square of side 15 feet. Find the volume of the steeple. 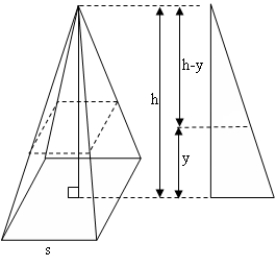
(Essay)
4.8/5  (33)
(33)
Use cylindrical shells to find the volume of the cone generated when the triangle with vertices (0, 0), (3, 0), (0, 12), where r > 0 and h > 0 is revolved about the y-axis.
(Essay)
4.9/5  (29)
(29)
Use cylindrical shells to find the volume of the solid when the region enclosed by y = x - 8, y = -x, x = 8, y = 0 is revolved about the y-axis.
(Multiple Choice)
5.0/5  (44)
(44)
A flat triangular plate whose dimensions are 13, 13, and 10 feet is submerged in water (weight density 62.4 lbs/ft3) so that its longer side is at the surface and parallel to it. Find the force against the surface of the plate. 
(Essay)
4.7/5  (30)
(30)
Answer true or false. The volume of the solid that results when the region enclosed by the curves y = x2 and x = y is revolved about x = 1, correct to three decimals, is 0.524.
(True/False)
4.7/5  (28)
(28)
Use cylindrical shells to find the volume of the solid that results when the area of the region enclosed by y2 = x3, x = 1, and y = 0 is revolved about the x-axis.
(Essay)
4.8/5  (37)
(37)
Find the area of the region enclosed by y = x2 - 4x and y = 16 - x2.
(Essay)
4.9/5  (38)
(38)
The base of a solid is a circle of radius 5. All sections that are perpendicular to the diameter are squares. Find the volume of the solid. 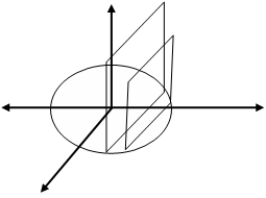
(Essay)
4.9/5  (34)
(34)
Showing 261 - 280 of 314
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)