Exam 14: Multiple Integrals
Exam 1: Limits and Continuity186 Questions
Exam 2: The Derivative198 Questions
Exam 3: Topics in Deifferentiation171 Questions
Exam 4: The Derivative in Graphing and Applications656 Questions
Exam 5: Integration323 Questions
Exam 6: Applications of the Definite Integral in Geometry, Science and Engineering314 Questions
Exam 7: Principle of Integral Evaluation269 Questions
Exam 8: Mathematical Modeling With Differential Equations77 Questions
Exam 9: Infinte Series288 Questions
Exam 10: Parametric and Polar Curves; Conic Sections199 Questions
Exam 11: Three-Dimensional Space; Vectors173 Questions
Exam 12: Vector-Valued Functions147 Questions
Exam 13: Partial Derivatives194 Questions
Exam 14: Multiple Integrals117 Questions
Exam 15: Topics in Vector Calculus149 Questions
Select questions type
A lamina with density (x, y) = 2xy + 11 is bounded by x = 2, x = 0, y = 0, y = x. Find its center of mass.
(Multiple Choice)
4.9/5  (36)
(36)
Find the volume of the solid formed by the right hemisphere of 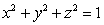 .
.
(Multiple Choice)
4.9/5  (36)
(36)
Find the volume of the solid formed by the right hemisphere of 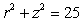 .
.
(Multiple Choice)
4.9/5  (31)
(31)
A uniform beam 1 m in length is supported at its center by a fulcrum. A mass of 20kg is placed at the left end, a mass of 8kg is placed on the beam 10 m from the left end, and a third mass is placed 4 m from the right end. What mass should the third mass be to achieve equilibrium?
(Multiple Choice)
4.9/5  (34)
(34)
The vector normal to the surface given by 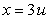 ,
,  , and
, and 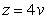 when
when 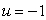 and
and 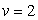 is
is
(Multiple Choice)
4.9/5  (32)
(32)
Use cylindrical coordinates to evaluate  , where R is the solid enclosed by
, where R is the solid enclosed by  and
and 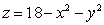 .
.
(Multiple Choice)
4.8/5  (31)
(31)
Find a parametric representation of the surface in terms of the parameters r and , where (r, , z) are the cylindrical coordinates of a point on the surface 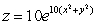 .
.
(Essay)
4.8/5  (44)
(44)
Find the volume of the region given by 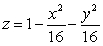 lying above the xy-plane.
lying above the xy-plane.
(Multiple Choice)
4.9/5  (41)
(41)
Evaluate the double integral 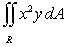 where R is the rectangular region bounded by the lines x = *1, x = 2, y = 0, and y = 4.
where R is the rectangular region bounded by the lines x = *1, x = 2, y = 0, and y = 4.
(Short Answer)
4.7/5  (32)
(32)
Use a triple integral to find the volume of the solid enclosed by x2 = 4y, y + z = 1, and z = 0.
(Essay)
4.9/5  (27)
(27)
The equation of the tangent plane to x = u, y = v, z = u + v2 where u = 2 and v = 2 is
(Multiple Choice)
4.7/5  (39)
(39)
Evaluate 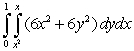 , by first sketching R then reversing the order of integration.
, by first sketching R then reversing the order of integration.
(Essay)
4.9/5  (28)
(28)
Evaluate the double integral 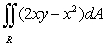 where R is the rectangle bounded by -1 x 3 and 0 y 3.
where R is the rectangle bounded by -1 x 3 and 0 y 3.
(Short Answer)
4.9/5  (36)
(36)
Use polar coordinates to evaluate 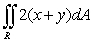 where R is the region enclosed by
where R is the region enclosed by 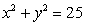 and x 0.
and x 0.
(Essay)
4.9/5  (32)
(32)
Showing 101 - 117 of 117
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)