Exam 6: Additional Topics In Trigonometery
Exam 1: Functions and Their Graphs513 Questions
Exam 2: Polynomial and Rational Functions456 Questions
Exam 3: Exponential and Logarithmic Functions266 Questions
Exam 4: Trigonometry384 Questions
Exam 5: Analytic Trigonometry265 Questions
Exam 6: Additional Topics In Trigonometery304 Questions
Exam 7: Systems Of Equations and Inequalities305 Questions
Exam 8: Matrices and Determinants283 Questions
Exam 9: Sequences Series and Probability405 Questions
Exam 10: Topics In Analytic Geometry556 Questions
Exam 11: Analytic Geometry In Three Dimensions256 Questions
Exam 12: Limits and An Introduction To Calculus259 Questions
Select questions type
Use the Law of Sines to solve for C and B.Round your answer to two decimal places.
A = 60°, a = 36, c = 40
(Multiple Choice)
4.9/5  (37)
(37)
Use the law of Cosines to solve the given triangle.Round your answer to two decimal places.
A = 14, b = 7, C = 118°
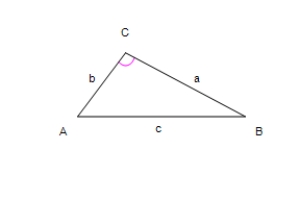
(Multiple Choice)
4.9/5  (38)
(38)
Find values for b such that the triangle has one solution.
A = 62°, a = 304.6
(Multiple Choice)
4.8/5  (41)
(41)
Determine whether u are v and orthogonal, parallel, or neither.
(Multiple Choice)
4.8/5  (41)
(41)
Determine whether the Law of Sines or the Law of Cosines is needed to solve the triangle.Then solve the triangle.Round your answer to two decimal places.
A = 46°, B = 39°, c = 1.4
(Multiple Choice)
4.8/5  (31)
(31)
Find the vector v that has a magnitude of 9 and is in the same direction as u, where .
(Multiple Choice)
4.8/5  (26)
(26)
Determine the angle in the design of the streetlight shown in the following figure.Round your answer upto decimal place. 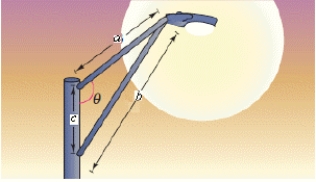
(Multiple Choice)
4.8/5  (34)
(34)
Use the Law of Sines to solve (if possible) the triangle.Round your answers to two decimal places.
A = 120°, a = b = 36
(Multiple Choice)
4.8/5  (37)
(37)
Determine the area of a triangle having the following measurements.Round your answer to two decimal places. B = 64 31 , a = 10 and c = 8.
(Multiple Choice)
5.0/5  (36)
(36)
Find the angle between the vectors. =\langle6,2\rangle =\langle7,0\rangle
(Round the answer to 2 decimal places.)
(Multiple Choice)
4.8/5  (35)
(35)
Use the vectors , to find the indicated quantity.State whether the result is a vector or a scalar.
(Multiple Choice)
4.9/5  (33)
(33)
A force of F pounds is required to pull an object weighing W pounds up a ramp inclined at θ degrees from the horizontal.
Find F if W = 100 pounds and θ = 11°.Approximate the answer to one decimal place.
(Multiple Choice)
4.8/5  (30)
(30)
Using the figure below, sketch a graph of the given vector.[The graphs in the answer choices are drawn to the same scale as the graph below.]
-u ![Using the figure below, sketch a graph of the given vector.[The graphs in the answer choices are drawn to the same scale as the graph below.] -u](https://storage.examlex.com/TB7698/11eae838_9cbc_e940_a6c3_c1522820b23e_TB7698_00.jpg)
(Multiple Choice)
4.9/5  (32)
(32)
Because of prevailing winds, a tree grew so that it was leaning 6° from the vertical.At a point 43 meters from the tree, the angle of elevation to the top of the tree is 30° (see figure).Find the height a of the tree. 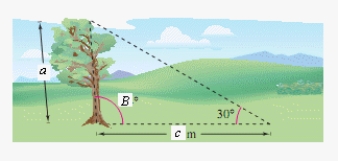 c = 43 m
B = 96°
(Round your answer to two decimal places.)
c = 43 m
B = 96°
(Round your answer to two decimal places.)
(Multiple Choice)
4.8/5  (33)
(33)
To determine the distance between two aircraft, a tracking station continuously determines the distance to each aircraft and the angle A between them (see figure).Determine the distance a between the planes when A = 44°, b = 37 miles, and c = 22 miles. 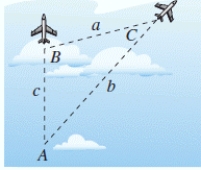
(Multiple Choice)
4.8/5  (37)
(37)
Use the vectors , to find the indicated quantity.State whether the result is a vector or a scalar.
(Multiple Choice)
4.8/5  (31)
(31)
Showing 121 - 140 of 304
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)