Exam 12: Limits and An Introduction To Calculus
Exam 1: Functions and Their Graphs513 Questions
Exam 2: Polynomial and Rational Functions456 Questions
Exam 3: Exponential and Logarithmic Functions266 Questions
Exam 4: Trigonometry384 Questions
Exam 5: Analytic Trigonometry265 Questions
Exam 6: Additional Topics In Trigonometery304 Questions
Exam 7: Systems Of Equations and Inequalities305 Questions
Exam 8: Matrices and Determinants283 Questions
Exam 9: Sequences Series and Probability405 Questions
Exam 10: Topics In Analytic Geometry556 Questions
Exam 11: Analytic Geometry In Three Dimensions256 Questions
Exam 12: Limits and An Introduction To Calculus259 Questions
Select questions type
Use the graph to determine (if it exists). 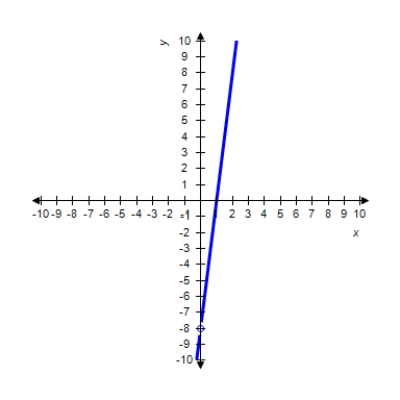
Free
(Multiple Choice)
4.7/5  (37)
(37)
Correct Answer:
C
Select the correct graph of the following function.
Free
(Multiple Choice)
4.8/5  (35)
(35)
Correct Answer:
C
Use the graph to determine the limit visually (if it exists).Then identify another function g2(x)that agrees with the given function at all but one point.
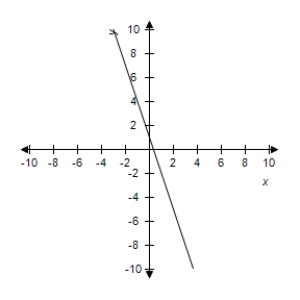
Free
(Multiple Choice)
4.8/5  (33)
(33)
Correct Answer:
D
Find the limit (if it exists).Use a graphing utility to verify your result graphically.
(Multiple Choice)
4.9/5  (34)
(34)
Find a formula for the slope of the graph of at the point .Then use it to find the slope at the given point.
(Multiple Choice)
4.8/5  (47)
(47)
Use the first six terms to predict the limit of the sequence (assume n begins with 1).
(Multiple Choice)
4.8/5  (28)
(28)
Use the limit process to find the slope of the graph of the function at the specified point.Use a graphing utility to confirm your result.
(Multiple Choice)
4.9/5  (33)
(33)
Select the correct graph for the following function and find the limit (if it exists)as x approaches 2.
(Multiple Choice)
4.9/5  (34)
(34)
Select the correct graph for the following function using a graphing utility.Determine whether the limit exists or not.
(Multiple Choice)
4.9/5  (37)
(37)
Find a formula for the slope of the graph of at the point .Then use it to find the slope at the given point.
(Multiple Choice)
4.7/5  (36)
(36)
Use the position function to find the velocity in feet/second at time seconds.The velocity at time seconds is given by .
(Multiple Choice)
4.8/5  (34)
(34)
Showing 1 - 20 of 259
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)