Exam 9: Sequences Series and Probability
Exam 1: Functions and Their Graphs513 Questions
Exam 2: Polynomial and Rational Functions456 Questions
Exam 3: Exponential and Logarithmic Functions266 Questions
Exam 4: Trigonometry384 Questions
Exam 5: Analytic Trigonometry265 Questions
Exam 6: Additional Topics In Trigonometery304 Questions
Exam 7: Systems Of Equations and Inequalities305 Questions
Exam 8: Matrices and Determinants283 Questions
Exam 9: Sequences Series and Probability405 Questions
Exam 10: Topics In Analytic Geometry556 Questions
Exam 11: Analytic Geometry In Three Dimensions256 Questions
Exam 12: Limits and An Introduction To Calculus259 Questions
Select questions type
Find the sum of the finite geometric sequence.(Round your answer to three decimal places.)
Free
(Multiple Choice)
4.8/5  (32)
(32)
Correct Answer:
C
Find the specified nth term in the expansion of the binomial.
Free
(Multiple Choice)
4.8/5  (39)
(39)
Correct Answer:
A
The educational attainment of a country population age 25 years or older in 2007 is shown in the circle graph.Use the fact that the population of people 25 years or older was approximately 243.32 million in 2007.Estimate the number of people 25 years or older who have advanced degrees.Round your anser to two decimal places. 
Free
(Multiple Choice)
4.9/5  (36)
(36)
Correct Answer:
A
A customer can choose one of two amplifiers, one of three compact disc players, and one of five speaker models for an entertainment system.Determine the number of possible system configurations.
(Multiple Choice)
4.9/5  (32)
(32)
Find a formula for an for the arithmetic sequence.
A1 = 4, d = 7
(Multiple Choice)
4.7/5  (31)
(31)
Assume that the sequence is defined recursively.Find the first three terms of the sequence.
(Multiple Choice)
4.7/5  (31)
(31)
Find the sum using the formulas for the sums of powers of integers.
(Multiple Choice)
4.8/5  (34)
(34)
Find the specified nth term in the expansion of the binomial.
(Multiple Choice)
4.8/5  (27)
(27)
Use the Binomial Theorem to expand the complex number.Simplify your result.
(Multiple Choice)
4.9/5  (29)
(29)
Find a formula for an for the arithmetic sequence.
A1 = 104, d = -6
(Multiple Choice)
4.9/5  (40)
(40)
Find the coefficient a of the term in the expansion of the binomial. Binomial Term
(Multiple Choice)
5.0/5  (34)
(34)
Determine whether the sequence is geometric.If so, find the common ratio.
(Multiple Choice)
4.7/5  (36)
(36)
Select the first five terms of the sequence.(Assume that n begins with 1.)
(Multiple Choice)
4.8/5  (30)
(30)
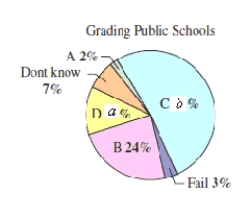
The figure shows the results of a recent survey in which 14 adults were asked to grade U.S.public schools.An adult is selected at random.What is the probability the adult will give the U.S.public schools a C or a D
( , ) 
(Multiple Choice)
4.9/5  (30)
(30)
Use mathematical induction to solve for all positive integers n.
(Multiple Choice)
4.8/5  (42)
(42)
Showing 1 - 20 of 405
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)