Exam 8: Confidence Intervals

What sample size is needed to estimate with 95 percent confidence the mean intake of calcium within 20 units of the true mean if the intake is normal with a variance of 1900 units?

What sample size is needed to obtain a 95 percent confidence interval for the proportion of fat in meat that is within 3 percent of the true value?

The quality control manager of a tire company wishes to estimate the tensile strength of a standard size of rubber used to make a class of radial tires.A random sample of 61 pieces of rubber from different production batches is subjected to a stress test.The test measures the force (in pounds)needed to break the rubber.According to the sample results,the average pressure is 238.4 pounds with a population standard deviation of 35 pounds.Determine the 98 percent confidence interval.

The weight of a product is measured in pounds.A sample of 50 units is taken from a batch.The sample yielded 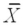= 75 lbs. ,and we know that σ² = 100 lbs.Calculate a 95 percent confidence interval for μ.

When sample size is 11,find t_.025.

The 95 percent confidence interval for the average weight of a product is from 72.23 lbs.to 77.77 lbs.Can we conclude that μ = 77,using a 95 percent confidence interval? Explain your answer.

The tolerance interval of 95.44 percent is ________ a 95.44 percent confidence interval.

When the level of confidence and sample standard deviation remain the same,a confidence interval for a population mean based on a sample of n = 100 will be narrower than a confidence interval for a population mean based on a sample of n = 50.

At the end of 1990,1991,and 1992,the average prices of a share of stock in a portfolio were $34.83,$34.65,and $31.26 respectively.To investigate the average share price at the end of 1993,a random sample of 30 stocks was drawn and their closing prices on the last trading day of 1993 were observed with a mean of 33.583 and a standard deviation of 19.149.Estimate the average price of a share of stock in the portfolio at the end of 1993 with a 90 percent confidence interval.

The coffee and soup machine at the local bus station is supposed to fill cups with 6 ounces of soup.Ten cups of soup are bought,with results of a mean of 5.93 ounces and a standard deviation of 0.13 ounces.Construct a 95.44 percent tolerance interval of the machine-fill amounts and a 95 percent confidence interval for the true machine-fill amount.

The success rate of a procedure is 37 per 120 cases in a sample.Find a 95 percent confidence interval for the actual success proportion of the procedure.

In a manufacturing process,we are interested in measuring the average length of a certain type of bolt.Past data indicate that the standard deviation is .25 inches.How many bolts should be sampled in order to make us 95 percent confident that the sample mean bolt length is within .02 inches of the true mean bolt length?

Given the following test scores,find a 95 percent confidence interval for the population mean: 148,154,158,160,161,162,166,170,182,195,236.Assume population normality.

A sample of 2,000 people yielded 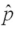= .52.Calculate a 99 percent confidence interval for p.

When the level of confidence and the sample size remain the same,a confidence interval for a population mean μ will be wider when the sample standard deviation s is small than when s is large.

Researchers studied the role that the age of workers has in determining the hours per month spent on personal tasks.A sample of 1,686 adults were observed for one month.The data are: Construct an 88 percent confidence interval for the mean hours spent on personal tasks for 18-24 year olds.

A tolerance interval is always longer than a corresponding confidence interval.

The 99 percent confidence interval for the average weight of a product is from 71.36 lbs.to 78.64 lbs.Can we conclude that μ is equal to 71,using a 99 percent confidence interval? Briefly explain.

There is an average of 22.455 employees at 22 office furniture dealers in a major metropolitan area,with a standard deviation of 18.52.Construct a 99 percent confidence interval for the true mean number of full-time employees at office furniture dealers.

When constructing a confidence interval for a population mean,if a population is normally distributed and a small sample is taken,then the distribution of 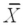is based on the ____________ distribution.