Exam 8: Calculus of Several Variables
Exam 1: Preliminaries209 Questions
Exam 2: Functions, Limits and the Derivative271 Questions
Exam 3: Differentiation331 Questions
Exam 4: Applications of the Derivative183 Questions
Exam 5: Exponential and Logarithmic Functions278 Questions
Exam 6: Integration313 Questions
Exam 7: Additional Topics in Integration250 Questions
Exam 8: Calculus of Several Variables205 Questions
Select questions type
Find the critical point(s) of the function.Then use the second derivative test to classify the nature of each point, if possible.Finally, determine the relative extrema of the function. 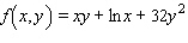
(Multiple Choice)
4.7/5  (28)
(28)
Determine whether the statement is true or false.If it is true, explain why it is true.If it is false, give an example to show why it is false. If 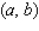 gives rise to a (constrained) relative extremum of
gives rise to a (constrained) relative extremum of 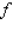 subject to the constraint
subject to the constraint 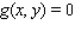 , then ,
, then , 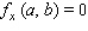 and
and  , simultaneously.
, simultaneously.
(Multiple Choice)
4.8/5  (40)
(40)
The population density of a certain city is given by the function  where the origin (0, 0) gives the location of the government center.Find the population inside the rectangular area described by
where the origin (0, 0) gives the location of the government center.Find the population inside the rectangular area described by 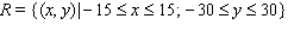
(Essay)
4.8/5  (33)
(33)
Find the volume of the solid bounded above by the surface z = f(x, y)
And below by the plane region R. 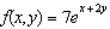 ; R is the triangle with vertices (0, 0), (5, 0) and (0, 5).
; R is the triangle with vertices (0, 0), (5, 0) and (0, 5).
(Multiple Choice)
4.9/5  (46)
(46)
Find the volume of the solid bounded above by the surface 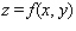 and below by the plane region
and below by the plane region 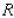 .
. 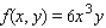 and
and 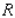 is the region bounded by the graphs of
is the region bounded by the graphs of 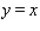 and
and  .
.
(Multiple Choice)
4.9/5  (36)
(36)
A building in the shape of a rectangular box is to have a volume of 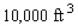 (see the figure).It is estimated that the annual heating and cooling costs will be $2/square foot for the top, $5/square foot for the front and back, and $2/square foot for the sides.Find the dimensions of the building that will result in a minimal annual heating and cooling cost.What is the minimal annual heating and cooling cost(C)?
(see the figure).It is estimated that the annual heating and cooling costs will be $2/square foot for the top, $5/square foot for the front and back, and $2/square foot for the sides.Find the dimensions of the building that will result in a minimal annual heating and cooling cost.What is the minimal annual heating and cooling cost(C)? 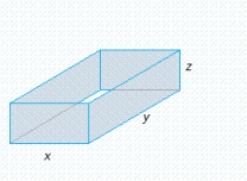
(Multiple Choice)
4.8/5  (41)
(41)
The volume of a cylindrical tank of radius r and height h is given by 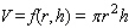 Find the volume of a cylindrical tank of radius 2 ft and height 8 ft.
Find the volume of a cylindrical tank of radius 2 ft and height 8 ft.
(Multiple Choice)
4.8/5  (33)
(33)
The projections of male life expectancy at age 65 in the United States are summarized in the following table.  (Here, x = 0 corresponds to the beginning of 2000.) Find the equation of the least-squares line for these data.Use the equation found to estimate the life expectancy at 65 of a male in 2050.
(Here, x = 0 corresponds to the beginning of 2000.) Find the equation of the least-squares line for these data.Use the equation found to estimate the life expectancy at 65 of a male in 2050.
(Multiple Choice)
4.8/5  (29)
(29)
Evaluate the double integral 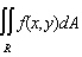 for the given function f(x, y) and the region R.
for the given function f(x, y) and the region R. 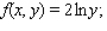 R is the rectangle defined by
R is the rectangle defined by 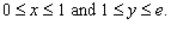
(Short Answer)
4.9/5  (36)
(36)
Use a double integral to find the volume of the solid shown in the figure. 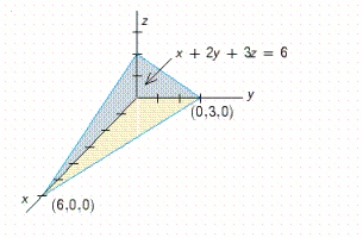
(Multiple Choice)
4.8/5  (38)
(38)
Evaluate the first partial derivatives of the function at the given point. 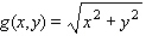 ;
; 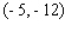
(Multiple Choice)
4.9/5  (35)
(35)
The Company requires that its corned beef hash containers have a capacity of  , be right circular cylinders, and be made of a tin alloy.Find the radius and height of the least expensive container that can be made if the metal for the side and bottom costs
, be right circular cylinders, and be made of a tin alloy.Find the radius and height of the least expensive container that can be made if the metal for the side and bottom costs  and the metal for the pull-off lid costs
and the metal for the pull-off lid costs  .
.
(Multiple Choice)
4.7/5  (33)
(33)
Use a double integral to find the volume of the solid shown in the figure. 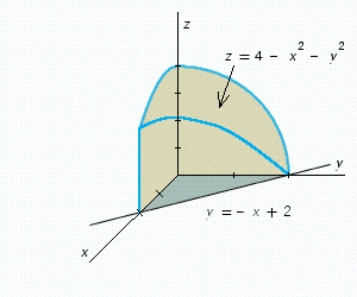
(Essay)
4.9/5  (27)
(27)
Evaluate the double integral 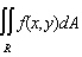 for the given function f(x, y) and the region R. f(x, y) = 6x + 12y; R is bounded by x = 1, x = 3, y = 0 and y = x + 1.
for the given function f(x, y) and the region R. f(x, y) = 6x + 12y; R is bounded by x = 1, x = 3, y = 0 and y = x + 1.
(Multiple Choice)
4.8/5  (26)
(26)
Find the average value of the given function f(x, y)
Over the plane region R.  ; R is the region bounded by the graph of y = 2x and y = 0 from x = 1 to x = 3.
; R is the region bounded by the graph of y = 2x and y = 0 from x = 1 to x = 3.
(Multiple Choice)
4.9/5  (38)
(38)
Find the critical point(s) of the function.Then use the second derivative test to classify the nature of each point, if possible.Finally, determine the relative extrema of the function. 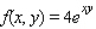
(Multiple Choice)
4.8/5  (41)
(41)
Evaluate the double integral  for the given function f(x, y) and the region R.
for the given function f(x, y) and the region R. 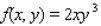 ; R is the rectangle defined by
; R is the rectangle defined by 
(Short Answer)
4.8/5  (42)
(42)
Showing 61 - 80 of 205
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)