Chat with AIExam 8: Calculus of Several Variables
Exam 1: Preliminaries209 Questions
Exam 2: Functions, Limits and the Derivative271 Questions
Exam 3: Differentiation331 Questions
Exam 4: Applications of the Derivative183 Questions
Exam 5: Exponential and Logarithmic Functions278 Questions
Exam 6: Integration313 Questions
Exam 7: Additional Topics in Integration250 Questions
Exam 8: Calculus of Several Variables205 Questions
Select questions type
Find the critical point(s) of the function.Then use the second derivative test to classify the nature of each point, if possible.Finally, determine the relative extrema of the function.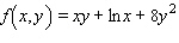 
Find the critical point(s) of the function.
Find the point(s) of maximum.
Find the point(s) of minimum.
Find the relative extrema of the function.
Find the critical point(s) of the function.
Find the point(s) of maximum.
Find the point(s) of minimum.
Find the relative extrema of the function. (Essay)
4.9/5 
(31)
(31) Find the second-order partial derivatives of the given function.Show that the mixed partial derivatives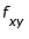 
and 
are equal. 
and 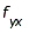are equal.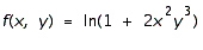 (Multiple Choice)
4.7/5 (30)
(30) 
Find the critical point(s) of the function.Then use the second derivative test to classify the nature of each point, if possible.Finally, determine the relative extrema of the function.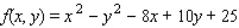 
(Multiple Choice)
4.8/5 (34)
(34) Use a double integral to find the volume of the solid shown in the figure.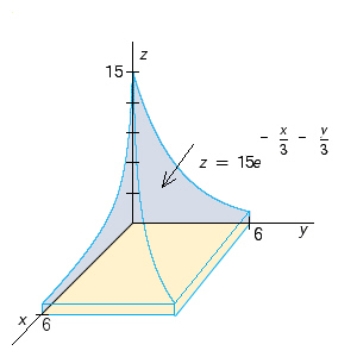 
(Essay)
4.8/5 (32)
(32) Evaluate the double integral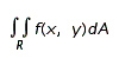 
for the given function f(x, y) and the region R. 
; R is the rectangle defined by 
for the given function f(x, y) and the region R. 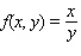; R is the rectangle defined by (Multiple Choice)
4.8/5 (31)
(31) 
subject to the constraints 
.Find the second-order partial derivatives of the given function.Show that the mixed partial derivatives 
and 
are equal. 
and 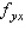are equal.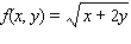 (Multiple Choice)
4.8/5 (44)
(44) 
subject to the constraint 
.
Evaluate the double integral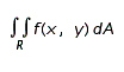 
for the function 
and the region 
. 
and 
is bounded by 
, 
, 
and 
.
for the function and the region . and 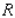is bounded by 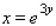, , 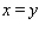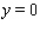and 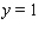. (Essay)
4.9/5 (37)
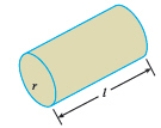
(37) Postal regulations specify that a parcel sent by parcel post may have a combined length and girth of no more than 
inches.Find the diamension of a cylendrical package of greatest volume that can be sent throught the mail.What is the volume of such a package? Hint: The length plus the grith is 
. 
inches.Find the diamension of a cylendrical package of greatest volume that can be sent throught the mail.What is the volume of such a package? Hint: The length plus the grith is 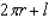. (Multiple Choice)
4.9/5 (37)
(37) 
subject to the constraint 
.Find the maximum and minimum values of the function 
subject to the constraint 
.
subject to the constraint 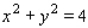. (Multiple Choice)
4.8/5 (38)
(38) Use a double integral to find the volume of the solid shown in the figure.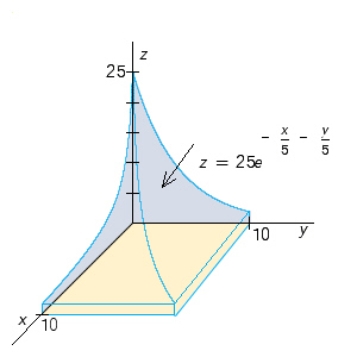 
(Multiple Choice)
4.8/5 (32)
(32) Sketch the level curves of the function corresponding to the given values of z.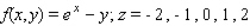 
(Multiple Choice)
4.9/5 (33)
(33) Evaluate the double integral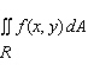 
for the given function f(x, y) and the region R. 
for the given function f(x, y) and the region R.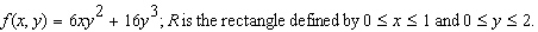 (Multiple Choice)
4.9/5 (40)
(40) 
.Compute 
.
subject to the constraint 
.
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)