Exam 8: Calculus of Several Variables
Exam 1: Preliminaries209 Questions
Exam 2: Functions, Limits and the Derivative271 Questions
Exam 3: Differentiation331 Questions
Exam 4: Applications of the Derivative183 Questions
Exam 5: Exponential and Logarithmic Functions278 Questions
Exam 6: Integration313 Questions
Exam 7: Additional Topics in Integration250 Questions
Exam 8: Calculus of Several Variables205 Questions
Select questions type
Evaluate the double integral  for the given function f(x, y) and the region R.
f(x, y) = 4x + 8y; R is bounded by x = 1, x = 3, y = 0 and y = x + 1.
for the given function f(x, y) and the region R.
f(x, y) = 4x + 8y; R is bounded by x = 1, x = 3, y = 0 and y = x + 1.
(Essay)
4.7/5  (38)
(38)
Let  Compute the following.
g(1, 1, 1) = __________
g(- 1, 0, 1) = __________
g(1, - 1, - 1) = __________
Compute the following.
g(1, 1, 1) = __________
g(- 1, 0, 1) = __________
g(1, - 1, - 1) = __________
(Essay)
4.9/5  (39)
(39)
The population density of a certain city is given by the function 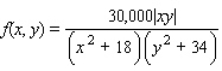 where the origin (0, 0) gives the location of the government center.Find the population inside the rectangular area described by
where the origin (0, 0) gives the location of the government center.Find the population inside the rectangular area described by 
(Multiple Choice)
4.8/5  (23)
(23)
Evaluate the double integral 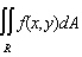 for the given function f(x, y) and the region R. f(x, y) = 2y + x; R is the rectangle defined by
for the given function f(x, y) and the region R. f(x, y) = 2y + x; R is the rectangle defined by 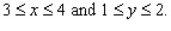
(Multiple Choice)
4.9/5  (34)
(34)
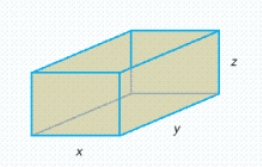
Postal regulations specify that the combined length and girth of a parcel sent by parcel post may not exceed 108 in.Find the dimensions of the rectangular package that would have the greatest possible volume under these regulations.(Hint: Let the dimensions of the box be  by
by 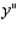 by
by 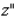 (see the figure below).Then,
(see the figure below).Then, 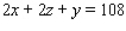 , and the volume
, and the volume 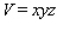 .So that
.So that 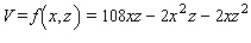 .Maximize
.Maximize 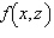 .)
.) 
(Multiple Choice)
4.8/5  (37)
(37)
Find the first partial derivative of the following function. 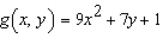
(Multiple Choice)
4.9/5  (32)
(32)
The following table gives the total sales of drugs (in billions of dollars) in one country from 1999  through 2003:
through 2003:  Find an equation of the least-square line for these data.
Find an equation of the least-square line for these data.
(Multiple Choice)
4.8/5  (28)
(28)
Evaluate the double integral 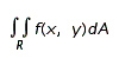 for the given function f(x, y) and the region R.
for the given function f(x, y) and the region R. 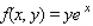 ; R is bounded by
; R is bounded by 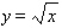 and y = x.
and y = x.
(Essay)
4.7/5  (47)
(47)
The management of UNICO Department Store decides to enclose an 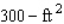 area outside their building to display potted plants.The enclosed area will be a rectangle, one side of which is provided by the external walls of the store.Two sides of the enclosure will be made of pine board, and the fourth side will be made of galvanized steel fencing material.If the pine board fencing costs $9/running foot and the steel fencing costs $3/running foot, determine the dimensions of the enclosure that will cost the least to erect.Round your answers to two decimal places.
area outside their building to display potted plants.The enclosed area will be a rectangle, one side of which is provided by the external walls of the store.Two sides of the enclosure will be made of pine board, and the fourth side will be made of galvanized steel fencing material.If the pine board fencing costs $9/running foot and the steel fencing costs $3/running foot, determine the dimensions of the enclosure that will cost the least to erect.Round your answers to two decimal places. 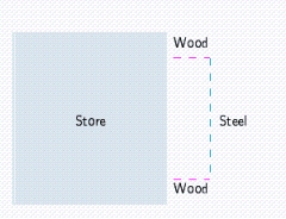
(Multiple Choice)
4.9/5  (33)
(33)
Determine whether the statement is true or false.If it is true, explain why it is true.If it is false, give an example to show why it is false.
If the data consist of two distinct points, then the least-squares line is just the line that passes through the two points.
(True/False)
4.8/5  (41)
(41)
The Company requires that its corned beef hash containers have a capacity of 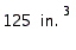 , be right circular cylinders, and be made of a tin alloy.Find the radius and height of the least expensive container that can be made if the metal for the side and bottom costs
, be right circular cylinders, and be made of a tin alloy.Find the radius and height of the least expensive container that can be made if the metal for the side and bottom costs  and the metal for the pull-off lid costs
and the metal for the pull-off lid costs  .
.
(Essay)
4.8/5  (34)
(34)
The total weekly revenue (in dollars) of Country Workshop associated with manufacturing and selling their rolltop desks is given by the function 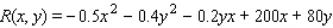 where x denotes the number of finished units and y denotes the number of unfinished units manufactured and sold each week.Compute
where x denotes the number of finished units and y denotes the number of unfinished units manufactured and sold each week.Compute 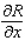 and
and 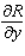 when x = 200 and y = 180.Interpret your results.
when x = 200 and y = 180.Interpret your results.
(Multiple Choice)
4.9/5  (31)
(31)
Find the critical point(s) of the function.Then use the second derivative test to classify the nature of each point, if possible.Finally, determine the relative extrema of the function. 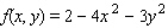
(Multiple Choice)
4.9/5  (29)
(29)
Find the second order partial derivative of the following function.Show that the mixed partial derivative 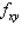 and
and 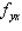 are equal.
are equal. 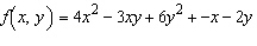
(Multiple Choice)
4.8/5  (36)
(36)
The volume of a cylindrical tank of radius r and height h is given by  Find the volume of a cylindrical tank of radius 1.5 ft and height 4 ft.
Find the volume of a cylindrical tank of radius 1.5 ft and height 4 ft.
(Essay)
4.8/5  (31)
(31)
An open rectangular box having a volume of 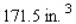 is to be constructed from a tin sheet.Find the dimensions of such a box if the amount of material used in its construction is to be minimal.
Hint: Let the dimensions of the box be x by y by z.Then, xyz = 108 and the amount of material used is given by S = xy + 2yz + 2xz.Show that
is to be constructed from a tin sheet.Find the dimensions of such a box if the amount of material used in its construction is to be minimal.
Hint: Let the dimensions of the box be x by y by z.Then, xyz = 108 and the amount of material used is given by S = xy + 2yz + 2xz.Show that 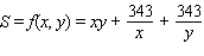 Minimize f(x, y)
x = __________
y = __________
z = __________
Minimize f(x, y)
x = __________
y = __________
z = __________
(Essay)
4.8/5  (33)
(33)
Find the critical point(s) of the function.Then use the second derivative test to classify the nature of each point, if possible.Finally, determine the relative extrema of the function. 
(Multiple Choice)
4.8/5  (38)
(38)
Showing 161 - 180 of 205
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)