Exam 1: Functions and Their Graphs

Choose the one alternative that best completes the statement or answers the question. Graph the function by starting with the graph of the basic function and then using the techniques of shifting, compressing, stretching, and/or reflecting. - $f(x)=x^{3}+1$

The graph of a function f is given. Use the graph to answer the question. -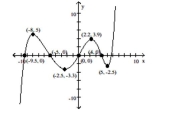 Find the numbers, if any, at which f has a local minimum. What are the local maxima?

Find the average rate of change for the function between the given values. - $f ( x ) = 2 x - 6$ ; from 1 to 3

Solve the problem. -While traveling at a constant speed in a car, the centrifugal acceleration passengers feel while the car is turning is inversely proportional to the radius of the turn. If the passengers feel an acceleration of 6 feet per second per Second when the radius of the turn is 50 feet, find the acceleration the passengers feel when the radius of the Turn is 100 feet.

Determine algebraically whether the function is even, odd, or neither. - $f ( x ) = - 4 x ^ { 2 } + 8$

Determine whether the equation defines y as a function of x. - $x ^ { 2 } + 2 y ^ { 2 } = 1$

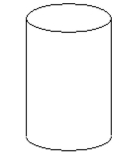
Choose the one alternative that best completes the statement or answers the question. -A steel can in the shape of a right circular cylinder must be designed to hold 550 cubic centimeters of juice (see figure). It can be shown that the total surface area of the can (including the ends) is given by $S ( r ) = 2 \pi r ^ { 2 } + \frac { 1,100 } { r }$ , where $r$ is the radius of the can in centimeters. Using the TABLE feature of a graphing utility, find the radius that minimizes the surface area (and thus the cost) of the can. Round to the nearest tenth of a centimeter.

Determine whether the graph is that of a function. If it is, use the graph to find its domain and range, the intercepts, if any, and any symmetry with respect to the x-axis, the y-axis, or the origin. -

Based on the graph, find the range of y = f(x). - $f ( x ) = \left\{ \begin{array} { l l } - \frac { 1 } { 4 } x & \text { if } x \neq 0 \\- 9 & \text { if } x = 0\end{array} \right.$

Graph the function by starting with the graph of the basic function and then using the techniques of shifting, compressing, stretching, and/or reflecting. - $f(x)=-(x+3)^{2}-2$

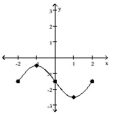
The graph of a function is given. Determine whether the function is increasing, decreasing, or constant on the given interval. - $(-2,-1)$

Find the value for the function. -Find $f ( - x )$ when $f ( x ) = 2 x ^ { 2 } + 3 x - 2$ .

Graph the function by starting with the graph of the basic function and then using the techniques of shifting, compressing, stretching, and/or reflecting. - $f(x)=|-x|$

For the given functions f and g, find the requested function and state its domain. - $f ( x ) = 16 - x ^ { 2 } ; g ( x ) = 4 - x$ Find $f + g$ .

Graph the function by starting with the graph of the basic function and then using the techniques of shifting, compressing, stretching, and/or reflecting. - $f(x)=\frac{1}{7} x^{3}$

Match the graph to the function listed whose graph most resembles the one given. -

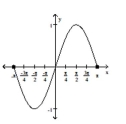
Determine whether the graph is that of a function. If it is, use the graph to find its domain and range, the intercepts, if any, and any symmetry with respect to the x-axis, the y-axis, or the origin. -

Solve the problem. -Bob wants to fence in a rectangular garden in his yard. He has 64 feet of fencing to work with and wants to use it all. If the garden is to be $x$ feet wide, express the area of the garden as a function of $x$ .

Find the value for the function. -Find $f ( 2 x )$ when $f ( x ) = - 2 x ^ { 2 } - 3 x + 1$

Solve the problem. -If $f ( x ) = \frac { x - B } { x - A } , f ( 4 ) = 0$ , and $f ( 9 )$ is undefined, what are the values of $A$ and $B$ ?