Exam 4: Exponential and Logarithmic Functions
Exam 1: Equations and Inequalities425 Questions
Exam 2: Functions and Graphs359 Questions
Exam 3: Polynomial and Rational Functions532 Questions
Exam 4: Exponential and Logarithmic Functions270 Questions
Exam 5: Trigonometric Functions386 Questions
Exam 6: Analytic Trigonometry226 Questions
Exam 7: Additional Topics in Trigonometry264 Questions
Exam 8: Systems of Equations and Inequalities288 Questions
Exam 9: Matrices and Determinants152 Questions
Exam 10: Conic Sections and Analytic Geometry228 Questions
Exam 11: Sequences, Induction, and Probability304 Questions
Exam 12: Prerequisites: Fundamental Concepts of Algebra409 Questions
Select questions type
Approximate the number using a calculator. Round your answer to three decimal places.
-The rabbit population in a forest area grows at the rate of monthly. If there are 160 rabbits in July, find how many rabbits (rounded to the nearest whole number) should be expected by next July. Use
(Multiple Choice)
4.9/5  (27)
(27)
Write the word or phrase that best completes each statement or answers the question.
Present data in the form of tables. For the data set shown by the table,
a. Create a scatter plot for the data.
b. Use the scatter plot to determine whether an exponential function or a logarithmic function is the best
choice for modeling the data.
- Number of Homes Built in a Town by Year Year Number of Homes 1985 11 1991 92 1994 145 1997 192 2002 224
(Essay)
4.8/5  (38)
(38)
Solve the exponential equation. Express the solution set in terms of natural logarithms.
-
(Multiple Choice)
4.8/5  (36)
(36)
Solve the equation by expressing each side as a power of the same base and then equating exponents.
-
(Multiple Choice)
4.8/5  (38)
(38)
Solve the problem.
-Larry has $2300 to invest and needs $2900 in 17 years. What annual rate of return will he need to get in order to accomplish his goal, if interest is compounded continuously? (Round your answer to two decimals.)
(Multiple Choice)
4.9/5  (36)
(36)
Use the compound interest formulas A
-Suppose that you have $10,000 to invest. Which investment yields the greater return over 9 years: 5.4% compounded monthly or 5.5% compounded quarterly?
(Multiple Choice)
4.9/5  (29)
(29)
Solve the logarithmic equation. Be sure to reject any value that is not in the domain of the original logarithmic
expressions. Give the exact answer.
-
(Multiple Choice)
4.8/5  (44)
(44)
Use common logarithms or natural logarithms and a calculator to evaluate to four decimal places
-
(Multiple Choice)
4.8/5  (25)
(25)
Use the compound interest formulas A
-Find the accumulated value of an investment of $1400 at 8% compounded quarterly for 4 years.
(Multiple Choice)
5.0/5  (38)
(38)
Solve.
-The value of a particular investment follows a pattern of exponential growth. In the year 2000, you invested money in a money market account. The value of your investment t years after 2000 is given by the exponential growth model . How much did you initially invest in the account?
(Multiple Choice)
4.9/5  (36)
(36)
Graph the function.
-Use the graph of to obtain the graph of .
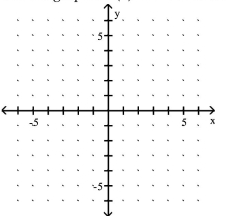
(Multiple Choice)
4.9/5  (42)
(42)
Solve the problem.
-Find out how long it takes a investment to earn interest if it is invested at compounded quarterly. Round to the nearest tenth of a year. Use the formula .
(Multiple Choice)
4.8/5  (32)
(32)
Use properties of logarithms to condense the logarithmic expression. Write the expression as a single logarithm
whose coefficient is 1. Where possible, evaluate logarithmic expressions.
-
(Multiple Choice)
4.8/5  (38)
(38)
Showing 241 - 260 of 270
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)