Exam 4: Exponential and Logarithmic Functions

Graph the function. -Use the graph of $\log _ { 3 } x$ to obtain the graph of $f ( x ) = \log _ { 3 } ( x + 1 )$ .

Use properties of logarithms to condense the logarithmic expression. Write the expression as a single logarithm whose coefficient is 1. Where possible, evaluate logarithmic expressions. - $\frac { 1 } { 6 } \left[ 3 \ln ( x + 7 ) - \ln x - \ln \left( x ^ { 2 } - 4 \right) \right]$

The graph of an exponential function is given. Select the function for the graph from the functions listed. -

Solve the problem. -The population of a certain country is growing at a rate of $1.3 \%$ per year. How long will it take for this country's population to double? Use the formula $t = \frac { \ln 2 } { k }$ , which gives the time, $t$ , for a population with growth rate k, to double. (Round to the nearest whole year.)

Use properties of logarithms to condense the logarithmic expression. Write the expression as a single logarithm whose coefficient is 1. Where possible, evaluate logarithmic expressions. - $3 \log _ { 6 } x + 5 \log _ { 6 } ( x - 6 )$

Solve the exponential equation. Express the solution set in terms of natural logarithms. - $e ^ { x + 5 } = 7$

Evaluate or simplify the expression without using a calculator. - $10 \log 3$

Use common logarithms or natural logarithms and a calculator to evaluate to four decimal places -Use the mathematical model for power gain, $G = \log \left( \frac { P _ { 0 } } { P _ { i } } \right) ^ { 10 }$ , where $P _ { 0 }$ is the output power in watts and $P _ { i }$ is the input power in watts. Determine the power gain $\mathrm { G }$ , in decibels, for an amplifier with an output $\mathrm { P } _ { 0 }$ of 21 watts and an input $P _ { i }$ of $1.2$ watts. Round to five decimal places if necessary.

Solve. -An endangered species of fish has a population that is decreasing exponentially $\left( A = A _ { 0 } e ^ { k t } \right)$ . The population 6 years ago was 1500 . Today, only 900 of the fish are alive. Once the population drops below 100 , the situation will be irreversible. When will this happen, according to the model? (Round to the nearest whole year.)

Graph the function. -Use the graph of $f ( x ) = \ln x$ to obtain the graph of $g ( x ) = 3 - \ln x$ .

Solve the logarithmic equation. Be sure to reject any value that is not in the domain of the original logarithmic expressions. Give the exact answer. - $\ln \sqrt { x + 5 } = 2$

Evaluate the expression without using a calculator. - $\log _ { 10 } 1000$

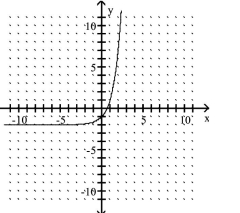
The graph of an exponential function is given. Select the function for the graph from the functions listed. -

Use the compound interest formulas A $A = P \left( 1 + \frac { r } { n } \right) ^ { n t } \text { and } A = P e ^ { r t } \text { to solve. }$ -Suppose that you have $4000 to invest. Which investment yields the greater return over 10 years: 6.25% compounded continuously or 6.3% compounded semiannually?

Solve the logarithmic equation. Be sure to reject any value that is not in the domain of the original logarithmic expressions. Give the exact answer. - $\log 3 x = \log 5 + \log ( x - 2 )$

Approximate the number using a calculator. Round your answer to three decimal places. -The function $\mathrm { D } ( \mathrm { h } ) = 8 \mathrm { e } ^ { - 0.4 \mathrm {~h} }$ can be used to determine the milligrams $\mathrm { D }$ of a certain drug in a patient's bloodstream h hours after the drug has been given. How many milligrams (to two decimals) will be present after 7 hours?

Solve the problem. -The pH of a solution ranges from 0 to 14. An acid has a pH less than 7 . Pure water is neutral and has a pH of 7 . The $\mathrm { pH }$ of a solution is given by $\mathrm { pH } = - \log x$ where $x$ represents the concentration of the hydrogen ions in the solution in moles per liter. Find the hydrogen ion concentration if the $\mathrm { pH } = 5$ .

Solve the logarithmic equation. Be sure to reject any value that is not in the domain of the original logarithmic expressions. Give the exact answer. - $\log x + \log ( x - 1 ) = \log 30$

Evaluate or simplify the expression without using a calculator. - $8 \log 10 ^ { 9.2 }$

Approximate the number using a calculator. Round your answer to three decimal places. - $4 ^ { - 2.9 }$