Exam 12: Congruence and Similarity With Constructions
Exam 1: An Introduction to Problem Solving85 Questions
Exam 2: Introduction to Logic and Sets153 Questions
Exam 3: Numeration Systems and Whole Number Operations194 Questions
Exam 4: Number Theory116 Questions
Exam 5: Integers122 Questions
Exam 6: Rational Numbers and Proportional Reasoning85 Questions
Exam 7: Rational Numbers As Decimals and Percents102 Questions
Exam 8: Real Numbers and Algebraic Thinking151 Questions
Exam 9: Probability129 Questions
Exam 10: Data Analysisstatistics: an Introduction57 Questions
Exam 11: Introductory Geometry115 Questions
Exam 12: Congruence and Similarity With Constructions121 Questions
Exam 13: Congruence and Similarity With Transformations56 Questions
Exam 14: Area, Pythagorean Theorem, and Volume124 Questions
Select questions type
Solve.
-Given the following triangle, construct the point P that is equidistant from the 3 vertices of the triangle. 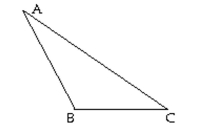
Free
(Essay)
4.8/5  (36)
(36)
Correct Answer:

Solve.
-How many different rotational symmetries does a square have?
Free
(Multiple Choice)
4.7/5  (34)
(34)
Correct Answer:
B
Answer the question.
-A building is 25 feet tall. Its shadow is 50 feet long. A nearby building is 15 feet tall. Find the length of the shadow of the second building.
Free
(Multiple Choice)
4.8/5  (30)
(30)
Correct Answer:
A
Provide an appropriate response.
-The two triangles shown below are congruent. The angles corresponding to , and (in order) are____
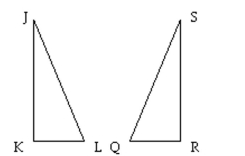
(Multiple Choice)
4.8/5  (36)
(36)
Provide an appropriate response.
-For what kind of triangles will the perpendicular bisectors of the sides intersect on a side of the triangle?
(Multiple Choice)
4.8/5  (31)
(31)
Use a ruler, protractor, and compass to construct, if possible, a triangle with the stated properties. If such a triangle cannot be drawn, explain why. Decide if there can be two or more noncongruent triangles with the stated properties.
-A triangle with sides of length 11 cm and 11 cm and a nonincluded angle of 46°
(Multiple Choice)
4.9/5  (37)
(37)
Solve.
-Describe how to construct a regular octagon in a given circle so that the circle circumscribes the octagon.
(Essay)
4.9/5  (37)
(37)
Answer the question.
-What minimum information is sufficient to determine the congruency of two equilateral triangles?
(Multiple Choice)
4.8/5  (28)
(28)
Solve.
-Given the following triangle, construct the point P that is equidistant from the 3 vertices of the triangle. 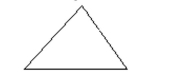
(Essay)
4.9/5  (32)
(32)
Solve.
-Explain how to construct a square given one side and constructing only parallel lines. If it is not possible, explain why.
(Essay)
5.0/5  (33)
(33)
Solve.
-Consider the isosceles triangles ABC and PQR. If side AB is glued to side PQ, what type of quadrilateral is formed? 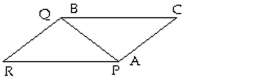
(Short Answer)
4.8/5  (37)
(37)
Use a ruler, protractor, and compass to construct, if possible, a triangle with the stated properties. If such a triangle cannot be drawn, explain why. Decide if there can be two or more noncongruent triangles with the stated properties.
-A triangle with angles measuring 11° and 113° and a nonincluded side of length 11 cm
(Multiple Choice)
4.9/5  (36)
(36)
Showing 1 - 20 of 121
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)