Exam 10: Conics, Parametric Equations, and Polar Coordinates
Exam 1: Preparation for Calculus125 Questions
Exam 2: Limits and Their Properties85 Questions
Exam 3: Differentiation193 Questions
Exam 4: Applications of Differentiation154 Questions
Exam 5: Integration184 Questions
Exam 6: Differential Equations93 Questions
Exam 7: Applications of Integration119 Questions
Exam 8: Integration Techniques and Improper Integrals130 Questions
Exam 9: Infinite Series181 Questions
Exam 10: Conics, Parametric Equations, and Polar Coordinates114 Questions
Exam 11: Vectors and the Geometry of Space130 Questions
Exam 12: Vector-Valued Functions85 Questions
Exam 13: Functions of Several Variables173 Questions
Exam 14: Multiple Integration143 Questions
Exam 15: Vector Anal142 Questions
Select questions type
Find a polar equation for the parabola with its focus at the pole, eccentricity e = 1, and directrix  .
.
(Multiple Choice)
4.9/5  (36)
(36)
Classify the graph of the equation as a circle, a parabola, an ellipse, or a hyperbola. 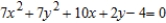
(Multiple Choice)
4.8/5  (23)
(23)
Classify the graph of the equation as a circle, a parabola, an ellipse, or a hyperbola. 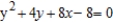
(Multiple Choice)
4.9/5  (33)
(33)
Find 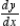 and
and 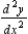 if possible, and find the slope and concavity (if possible) at the point corresponding to
if possible, and find the slope and concavity (if possible) at the point corresponding to  .
. 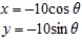
(Multiple Choice)
4.9/5  (37)
(37)
The parametric equations for the path of a projectile launched at a height h feet above the ground, at an angle  with the horizontal and having an initial velocity of
with the horizontal and having an initial velocity of  feet per second is given by
feet per second is given by 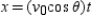 and
and 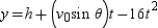 . The center field fence in a ballpark is 10 feet high and 400 feet from home plate. The ball is hit 2 feet above the ground. It leaves the bat at an angle of
. The center field fence in a ballpark is 10 feet high and 400 feet from home plate. The ball is hit 2 feet above the ground. It leaves the bat at an angle of  degrees with the horizontal at a speed of 95 miles per hour as shown in the figure. Find the minimum angle at which the ball must leave the bat in order for the hit to be a home run using the parametric equations
degrees with the horizontal at a speed of 95 miles per hour as shown in the figure. Find the minimum angle at which the ball must leave the bat in order for the hit to be a home run using the parametric equations 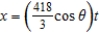 and
and 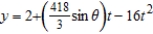 . Round your answer to one decimal place.
. Round your answer to one decimal place. 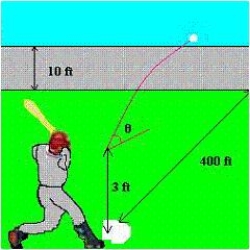
(Multiple Choice)
4.8/5  (37)
(37)
Find the center, foci, vertices, and eccentricity of the ellipse. 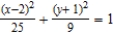
(Multiple Choice)
4.9/5  (42)
(42)
Neptune moves in an elliptical orbit with the sun at one of the foci. The length of the half of the major axis is 4,502,899,800 kilometers, and the eccentricity is 0.0112. Find the maximum distance (aphelion) of Neptune from the sun. Round your answer to nearest kilometer.
(Multiple Choice)
4.8/5  (31)
(31)
Use the result, "the set of parametric equations for the line passing through  and
and  is
is  " to find a set of parametric equations for the line passing through
" to find a set of parametric equations for the line passing through 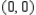 and
and 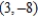 .
.
(Multiple Choice)
4.9/5  (37)
(37)
Find the area of the surface generated by revolving the curve  about the x-axis on the interval
about the x-axis on the interval 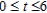 .
.
(Multiple Choice)
4.8/5  (33)
(33)
Find all points of intersection of the graphs of the equations. 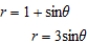
(Multiple Choice)
4.9/5  (28)
(28)
Determine the t intervals on which the curve  is concave downward or concave upward.
is concave downward or concave upward.
(Multiple Choice)
4.9/5  (38)
(38)
Find the points of intersection of the graphs of the equations. 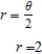
(Multiple Choice)
4.9/5  (35)
(35)
Showing 81 - 100 of 114
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)