Exam 9: Linear Transformations
Exam 1: Systems of Linear Equations57 Questions
Exam 2: Euclidean Space48 Questions
Exam 3: Matrices76 Questions
Exam 4: Subspaces60 Questions
Exam 5: Determinants48 Questions
Exam 6: Eigenvalues and Eigenvectors75 Questions
Exam 7: Vector Spaces45 Questions
Exam 8: Orthogonality75 Questions
Exam 9: Linear Transformations60 Questions
Exam 10: Inner Product Spaces45 Questions
Exam 11: Additional Topics and Applications75 Questions
Select questions type
Suppose B is the matrix of 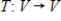 with respect to the basis H. Find the matrix A of T with respect to the basis G.
with respect to the basis H. Find the matrix A of T with respect to the basis G. 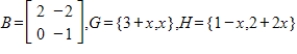
(Essay)
4.8/5  (41)
(41)
Suppose B is the matrix of 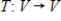 with respect to a basis H, and S is the change of basis matrix from a basis G to H. Find the matrix A of T with respect to G.
with respect to a basis H, and S is the change of basis matrix from a basis G to H. Find the matrix A of T with respect to G. 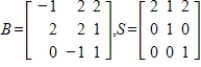
(Essay)
4.9/5  (40)
(40)
Determine the kernel and range of the linear transformation 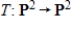 given by
given by
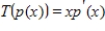 .
.
(Essay)
4.8/5  (33)
(33)
If 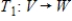 and
and 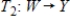 are isomorphisms, then the composition
are isomorphisms, then the composition 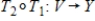 is an isomorphism.
is an isomorphism.
(True/False)
4.8/5  (41)
(41)
Suppose 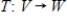 is a linear transformation and
is a linear transformation and 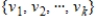 is a set of vectors in V. If
is a set of vectors in V. If  is a linearly dependent set, then so is
is a linearly dependent set, then so is 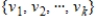 .
.
(True/False)
4.9/5  (39)
(39)
If A, B, C, and D are 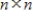 matrices such that A is similar to B and C is similar to D, then AC is similar to BD.
matrices such that A is similar to B and C is similar to D, then AC is similar to BD.
(True/False)
4.7/5  (33)
(33)
Suppose A and B are 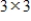 invertible matrices. Find
invertible matrices. Find 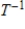 for the isomorphism
for the isomorphism 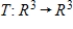 , where
, where  .
.
(Essay)
4.9/5  (33)
(33)
If 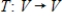 is a linear transformation, with V a vector space having basis
is a linear transformation, with V a vector space having basis 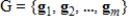 , and if
, and if 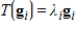 for all i, where
for all i, where  is a scalar, then the matrix of T is diagonal, where G is the basis used for both the domain and codomain.
is a scalar, then the matrix of T is diagonal, where G is the basis used for both the domain and codomain.
(True/False)
4.8/5  (34)
(34)
Suppose A is the matrix of the linear transformation 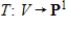 with respect to bases G and Q, respectively. Find
with respect to bases G and Q, respectively. Find 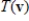 for the given
for the given 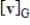 .
.

(Essay)
4.9/5  (43)
(43)
Determine whether the function 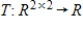 is a linear transformation, where
is a linear transformation, where 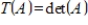 .
.
(Short Answer)
4.9/5  (43)
(43)
Showing 21 - 40 of 60
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)