Exam 9: Linear Transformations
Exam 1: Systems of Linear Equations57 Questions
Exam 2: Euclidean Space48 Questions
Exam 3: Matrices76 Questions
Exam 4: Subspaces60 Questions
Exam 5: Determinants48 Questions
Exam 6: Eigenvalues and Eigenvectors75 Questions
Exam 7: Vector Spaces45 Questions
Exam 8: Orthogonality75 Questions
Exam 9: Linear Transformations60 Questions
Exam 10: Inner Product Spaces45 Questions
Exam 11: Additional Topics and Applications75 Questions
Select questions type
The vector spaces 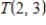 and
and 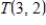 are isomorphic. (Recall that
are isomorphic. (Recall that  denotes the vector space of all linear transformations from
denotes the vector space of all linear transformations from 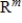 into
into 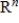
(True/False)
4.9/5  (35)
(35)
If A and B are similar matrices and B and C are similar matrices, then A and C are similar matrices.
(True/False)
4.9/5  (41)
(41)
Find the matrix A of the linear transformation 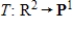 with respect to bases G and Q, respectively.
with respect to bases G and Q, respectively.
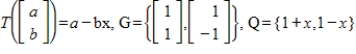
(Essay)
4.9/5  (36)
(36)
Let Q be an 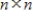 invertible matrix, and let P be an
invertible matrix, and let P be an 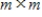 invertible matrix. Determine whether the function
invertible matrix. Determine whether the function  is a linear transformation, where
is a linear transformation, where 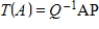 , and if so, determine if T is one-to-one and/or onto.
, and if so, determine if T is one-to-one and/or onto.
(Short Answer)
4.8/5  (31)
(31)
If 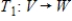 and
and 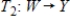 are linear transformations, then the composition
are linear transformations, then the composition 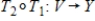 is a linear transformation.
is a linear transformation.
(True/False)
5.0/5  (38)
(38)
If S is a nonzero subspace of  , determine whether the function
, determine whether the function 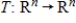 is a linear transformation, where
is a linear transformation, where 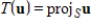 , and if so, determine
, and if so, determine  .
.
(Short Answer)
4.9/5  (39)
(39)
Let V be a vector space with basis 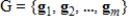 , and let
, and let 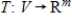 be the linear transformation
be the linear transformation 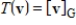 . Then T is an isomorphism, and the matrix of T with respect to G and the standard basis is the
. Then T is an isomorphism, and the matrix of T with respect to G and the standard basis is the 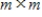 identity matrix.
identity matrix.
(True/False)
4.8/5  (43)
(43)
Let 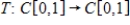 be defined by
be defined by  . Verify that
. Verify that 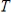 is a linear transformation, determine if
is a linear transformation, determine if 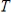 is an isomorphism, and if so, find
is an isomorphism, and if so, find 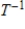 .
.
(Essay)
4.8/5  (30)
(30)
The subspace S of 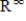 of all sequences that are eventually zero is isomorphic to
of all sequences that are eventually zero is isomorphic to  .
.
(True/False)
4.8/5  (32)
(32)
Determine if the linear transformation 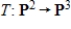 is one-to-one and/or onto.
is one-to-one and/or onto.
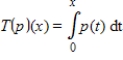
(Short Answer)
4.9/5  (31)
(31)
Determine whether the function  is a linear transformation, where
is a linear transformation, where 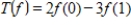 .
.
(Short Answer)
4.9/5  (33)
(33)
If V is a finite-dimensional vector space, then the matrix A of a linear transformation 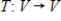 is invertible if and only if T is one-to-one.
is invertible if and only if T is one-to-one.
(True/False)
4.9/5  (39)
(39)
The function  defined by
defined by 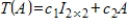 , where
, where 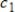 and
and  are nonzero scalars, is a linear transformation.
are nonzero scalars, is a linear transformation.
(True/False)
4.8/5  (41)
(41)
Showing 41 - 60 of 60
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)