Exam 13: Game Theory and Strategic Play

The following figure depicts four simultaneous-move games. The first element of each payoff cell is the payoff to Player A. The other element is the payoff to Player B. 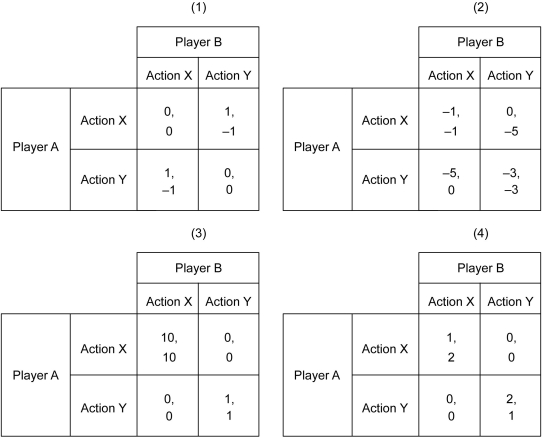-Refer to the figure above.Which of these simultaneous-move games best represent the following scenario: a coordination game.Ann and Bob have the opportunity to either go to the same party (X)or stay at their respective homes (Y).They would be much happier if they both went to the party,but staying at home is better than going to the party alone.

Scenario: The payoff matrix given below shows the payoffs to two rival firms in millions of U.S. dollars for each strategy they choose. The first number listed in each cell is the payoff to the row player, and the second number listed is the payoff to the column player. 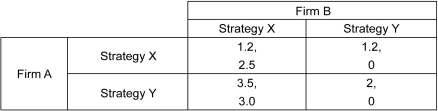-Refer to the scenario above.In the dominant strategy equilibrium,the payoff to Firm A is ________.

Scenario: Vladimir and Alphonso are collectors of Toy Story figurines. Each has six dollar bills to spend on three figurines-Sheriff Woody, Buzz Lightyear, and Jessie-that are put up for auction where Vlad and Alph are the only bidders. They simultaneously and independently write down their bids, in whole numbers adding up to $6, for the three figurines and give them to the auctioneer. Whoever wrote the higher number for a figurine wins it. For example, if Vlad bids ($3, $1, $2), meaning $3 for Sheriff Woody, $1 for Buzz Lightyear, and $2 for Jessie, and Alph bids ($2, $3, $1), then Vlad wins Sheriff Woody and Jessie, while Alph wins Buzz Lightyear. If there is a tie, then a fair coin is flipped to determine the winner so that each bidder has 50 percent chance of winning a figurine. Vlad and Alph consider this case as "winning" a $\frac{1}{2}$ figurine. Vlad and Alph care only about how many more, or less, figurines they win compared to the other. -Refer to the scenario above.If Vlad bid ($2,$2,$2),then he can win at least ________,no matter what Alph does.

Which of the following is true of a Nash equilibrium?

Scenario: The payoff matrix given below shows the payoffs to two firms in millions of U.S. dollars for choosing two alternative strategies. The first number listed in each cell is the payoff to the row player, and the second number listed is the payoff to the column player. 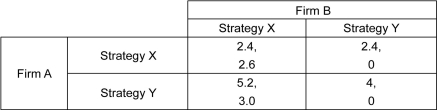-Refer to the scenario above.What is the payoff to Firm B in equilibrium?

Scenario: Contiguous states often use tax policy to attract residents, firms, and economic activity. These "tax competitions" between states can be modeled with game theory. Suppose New Jersey currently has a state sales tax of 7 percent and Pennsylvania has a state sales tax of 6 percent. The game shown below models the effect of a reduction in each state's sales tax rate to 3 percent on each state's sales tax revenue. Assume the motivation of each state is to maximize tax revenue. The first number in a cell is the payoff to New Jersey; the second number is the payoff to Pennsylvania. 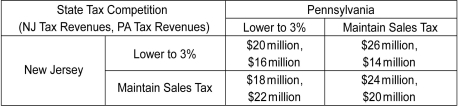(Source: John Greenwald, "A No-Win War Between the States," Time, April 8, 1996, 44-45. -Refer to the scenario above.Is there a set of payoffs that is superior to the payoffs realized at the dominant strategy equilibrium?

Scenario: Miguel and Stephanie are competitors who sell tacos out of their food trucks by the same beach. For each taco they sell, they get a profit of $1.00. Each morning they must decide at which of the three legally permitted locations, A, B, or C, they should park their trucks. The picture below shows the three locations. In each of the three locations, there are 100 customers per day, and each customer buys a taco from the nearest vendor. If the two trucks are in the same location, then each vendor gets half of the customers. So, for example, if Miguel's truck is in location A, and Stephanie's is also in location A, then half of customers from locations A, B, and C will buy from Miguel's and the other half will buy from Stephanie's, so that each gets a profit of $150. If Miguel's truck is in location A and Stephanie's is in location B, then everyone in location A will buy from Miguel and everyone from locations B and C will buy from Stephanie, so that Miguel's profit is $100 while Stephanie's is $200. If Miguel's truck is in location A and Stephanie's is in location C, then everyone in A plus a half from B will buy from Miguel, and everyone in C plus half from B will buy from Stephanie so that each vendor gets a profit of $150, and so on. The table below is the payoff matrix for this game. The first number in each cell is Miguel's profit, and the second number is Stephanie's profit. -Refer to the scenario above.If Miguel's truck is in location A,what is Stephanie's best response?

Scenario: Consider the tragedy of the commons problem. Suppose two firms (Row Inc. and Colm Inc.) are involved in a production process that exploits a natural resource. Each firm has two options: 1 and 2. The matrix below shows the game matrix for these two firms. The first number listed in each cell is the payoff to Row Inc., and the second number listed is the payoff to Colm Inc. 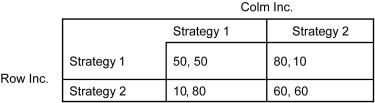-Refer to the scenario above.Considering the tragedy of the commons that these two firms are involved in,Strategy 1 is ________,and Strategy 2 is ________.

Scenario: Rita and Mike have been caught cheating on an examination. They are taken to separate rooms for interrogation. Their payoff matrix is given below. The first outcome listed in each cell is the payoff to the row player, and the second outcome listed is the payoff to the column player. -Refer to the scenario above.This is an example of a(n)________.

Scenario: The following payoff matrix represents a simultaneous-move game between two players: Dan and Jessa. Each player has two choices: Strategy X or Strategy Y. The first number in each cell is the payoff to Dan, and the second number is the payoff to Jessa. 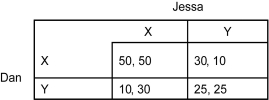-Refer to the scenario above.Which statement is true?

Scenario: Two rival firms charge equal prices for their products, which are perfect substitutes. Firm 1 is considering offering a 10 percent discount on the market price to increase sales. The game tree below shows the respective payoffs to each firm, depending on the decisions each makes. -Refer to the scenario above.Which of the following is true?

Scenario: Miguel and Stephanie are competitors who sell tacos out of their food trucks by the same beach. For each taco they sell, they get a profit of $1.00. Each morning they must decide at which of the three legally permitted locations, A, B, or C, they should park their trucks. The picture below shows the three locations. In each of the three locations, there are 100 customers per day, and each customer buys a taco from the nearest vendor. If the two trucks are in the same location, then each vendor gets half of the customers. So, for example, if Miguel's truck is in location A, and Stephanie's is also in location A, then half of customers from locations A, B, and C will buy from Miguel's and the other half will buy from Stephanie's, so that each gets a profit of $150. If Miguel's truck is in location A and Stephanie's is in location B, then everyone in location A will buy from Miguel and everyone from locations B and C will buy from Stephanie, so that Miguel's profit is $100 while Stephanie's is $200. If Miguel's truck is in location A and Stephanie's is in location C, then everyone in A plus a half from B will buy from Miguel, and everyone in C plus half from B will buy from Stephanie so that each vendor gets a profit of $150, and so on. The table below is the payoff matrix for this game. The first number in each cell is Miguel's profit, and the second number is Stephanie's profit. -Refer to the scenario above.Does Miguel have a dominant strategy?

Scenario: The payoff matrix given below shows the payoffs to two firms in millions of U.S. dollars for choosing two alternative strategies. The first number listed in each cell is the payoff to the row player, and the second number listed is the payoff to the column player. -Refer to the scenario above.Which of the following is true in this case?

Scenario: Miguel and Stephanie are competitors who sell tacos out of their food trucks by the same beach. For each taco they sell, they get a profit of $1.00. Each morning they must decide at which of the three legally permitted locations, A, B, or C, they should park their trucks. The picture below shows the three locations. In each of the three locations, there are 100 customers per day, and each customer buys a taco from the nearest vendor. If the two trucks are in the same location, then each vendor gets half of the customers. So, for example, if Miguel's truck is in location A, and Stephanie's is also in location A, then half of customers from locations A, B, and C will buy from Miguel's and the other half will buy from Stephanie's, so that each gets a profit of $150. If Miguel's truck is in location A and Stephanie's is in location B, then everyone in location A will buy from Miguel and everyone from locations B and C will buy from Stephanie, so that Miguel's profit is $100 while Stephanie's is $200. If Miguel's truck is in location A and Stephanie's is in location C, then everyone in A plus a half from B will buy from Miguel, and everyone in C plus half from B will buy from Stephanie so that each vendor gets a profit of $150, and so on. The table below is the payoff matrix for this game. The first number in each cell is Miguel's profit, and the second number is Stephanie's profit. -Refer to the scenario above.Is Miguel choosing B and Stephanie choosing A a Nash equilibrium? Why?

The following payoff matrix represents a simultaneous-move game between two players: Alec and Ben. Each player has to choices: Red and Yellow. The first number in each cell is the payoff to Alec, and the second number is the payoff to Ben. 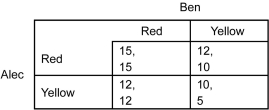-Refer to the table above.Which statement is true?

The following figure depicts four sequential games. Player A is the first to move and Player B is the second to move. 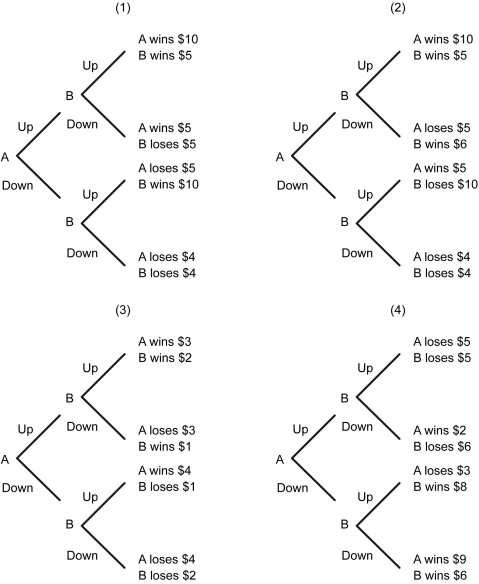-Refer to the figure above.In which game does Player A win money while Player B loses money in equilibrium?

A payoff matrix shows the ________.

A road that runs by your house has been damaged by a thunderstorm.The local authority will repair the road if at least one person lodges a complaint.There are only two houses on the road,yours and your neighbor's.The matrix below shows your payoffs depending on who decides to lodge a complaint.The first number listed in each cell is the payoff to the row player,and the second number listed is the payoff to the column player. 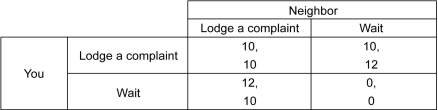a)Does this game have a Nash equilibrium? Explain your answer. b)Does this game have a dominant strategy equilibrium? Explain your answer.

Scenario: Diana and Artemis can either jointly hunt stags (adult deer) or individually hunt rabbits. Hunting stags requires cooperation, but if the two cooperate, then they get a large amount of meat. If either hunts stags alone, the chance of success is zero. Hunting rabbits is easy for an individual and even easier for if the two cooperate, but rabbits would provide smaller amounts of meat than would stags. The payoff matrix below reflects this situation. Payoffs are days' worth of meat. -Refer to the scenario above.Which of the following is true?

Another game frequently played in MBA strategy classes is the acquire a company game.The set-up of the game is as follows.Company A has only one asset,offshore oil leases.The expected profit from these leases is uniformly distributed between $0 and $100 million,so the expected value to company A is $50 million.Company B is thinking of acquiring Company A.Company B's management is more efficient than A's; specifically,the value of A's assets under B's management is 1.5 times greater.For example,the expected value of A's oil leases under B's management is 1.5 $50 million,or $75 million.Company B can submit a written bid to acquire Company A.A will review the bid,and accept or reject the offer after learning the value of the oil leases with certainty. You are advising Company B on its potential acquisition of Company A.What is the optimal bid for Company B to submit?