Exam 14: Functions of Two or More Variables
Exam 1: Linear Equations and Functions245 Questions
Exam 2: Quadratic and Other Special Functions120 Questions
Exam 3: Matrices230 Questions
Exam 4: Inequalities and Linear Programming119 Questions
Exam 5: Exponential and Logarithmic Functions109 Questions
Exam 6: Mathematics of Finance131 Questions
Exam 7: Introduction to Probability180 Questions
Exam 8: Further Topics in Probability and Data Description114 Questions
Exam 9: Derivatives249 Questions
Exam 10: Derivatives172 Questions
Exam 11: Derivatives Continued139 Questions
Exam 12: Indefinite Integrals120 Questions
Exam 13: Definite Integrals - Techniques370 Questions
Exam 13: A: Definite Integrals - Techniques370 Questions
Exam 14: Functions of Two or More Variables122 Questions
Exam 15: Algebraic Concepts 240 Questions
Exam 15: Algebraic Concepts 374 Questions
Exam 15: Algebraic Concepts 496 Questions
Exam 15: Algebraic Concepts 599 Questions
Select questions type
Find the values for each of the dimensions of a closed-top box of length x, width y, and height z (in inches) if the volume equals 32,768 cubic inches and the box requires the least amount of material to make.
(Multiple Choice)
4.8/5  (36)
(36)
Suppose that the utility function for two commodities is given by 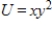 and that the budget constraint is
and that the budget constraint is 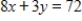 . What values of x and y will maximize utility?
. What values of x and y will maximize utility?
(Multiple Choice)
4.9/5  (37)
(37)
Use the points given in the table to write the equation of the line that is the best fit for the points. Round numerical values in your answer to two decimal places.
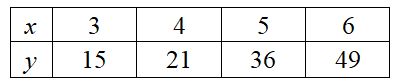
(Multiple Choice)
4.9/5  (33)
(33)
Suppose the production function for a product is 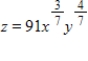 , where x is the capital expenditures and y is the number of work-hours. Find the marginal productivity of x.
, where x is the capital expenditures and y is the number of work-hours. Find the marginal productivity of x.
(Multiple Choice)
4.8/5  (30)
(30)
A company manufactures two products, A and B. If x is the number of thousands of units of A and y is the number of thousands of units of B, then the cost and revenue in thousands of dollars are 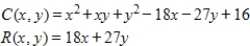
Find the number of each type of product that should be manufactured to maximize profit.
Find the number of each type of product that should be manufactured to maximize profit.
(Multiple Choice)
4.9/5  (27)
(27)
The demand functions for two products are given by 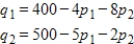
Where p1 and p2 are in dollars and q1 and q2 are numbers of units.
Find the demand for each of the products if the price of the first is
Where p1 and p2 are in dollars and q1 and q2 are numbers of units.
Find the demand for each of the products if the price of the first is 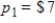 and the price of the second is
and the price of the second is 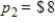 .
.
(Multiple Choice)
5.0/5  (32)
(32)
Suppose that the production function for a product is 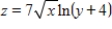 , where x represents the number of work-hours and y represents the available capital (per week). Find the marginal productivity of x.
, where x represents the number of work-hours and y represents the available capital (per week). Find the marginal productivity of x.
(Multiple Choice)
4.7/5  (40)
(40)
Find x and y such that 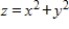 subject to the condition
subject to the condition  attains a minimum value.
attains a minimum value.
(Multiple Choice)
4.8/5  (30)
(30)
Find the maximum value of 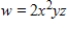 subject to the constraint
subject to the constraint  ,
,  ,
, 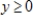 , and
, and 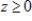 .
.
(Multiple Choice)
4.7/5  (42)
(42)
Suppose the production function for a product is 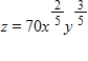 , where x is the capital expenditures and y is the number of work-hours. Find the marginal productivity of y.
, where x is the capital expenditures and y is the number of work-hours. Find the marginal productivity of y.
(Multiple Choice)
4.7/5  (31)
(31)
Evaluate the function at the given values of the independent variables. 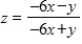 ,
,  ,
, 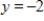
(Multiple Choice)
4.8/5  (25)
(25)
Suppose that the number of thousands of insects killed by two brands of pesticide is given by 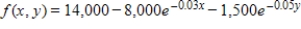 , where x is the number of liters of Brand X and y is the number of liters of Brand Y. What is the rate of change of insect deaths with respect to the number of liters of Brand X if 100 liters of each brand are currently being used? Round your answer to two decimal places.
, where x is the number of liters of Brand X and y is the number of liters of Brand Y. What is the rate of change of insect deaths with respect to the number of liters of Brand X if 100 liters of each brand are currently being used? Round your answer to two decimal places.
(Multiple Choice)
4.9/5  (42)
(42)
Suppose that the utility function for two commodities is given by 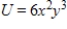 , and the budget constraint is
, and the budget constraint is 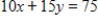 . Find the values of x and y that maximize utility.
. Find the values of x and y that maximize utility.
(Multiple Choice)
4.9/5  (36)
(36)
The cost (in dollars) of manufacturing one item is given by 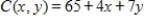 , where x is the cost of 1 hour of labor and y is the cost of 1 pound of material. If the hourly cost of labor is $5, and the material costs $2 per pound, what is the cost of manufacturing one of these items?
, where x is the cost of 1 hour of labor and y is the cost of 1 pound of material. If the hourly cost of labor is $5, and the material costs $2 per pound, what is the cost of manufacturing one of these items?
(Multiple Choice)
4.7/5  (27)
(27)
Showing 81 - 100 of 122
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)