Exam 13: Limits: a Preview of Calculus

Use the definition of area as a limit to find the area of the region that lies under the graph of $f ( x ) = x ^ { 2 }$ over the interval $1 \leq x \leq 5$ .

For the function g whose graph is given, state the value of the given quantity if it exists. 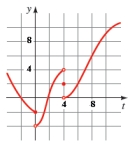a) $\lim _ { x \rightarrow 0 ^ { - } } g ( t )$ b) $\lim _ { x \rightarrow 0 ^ { + } } g ( t )$ c) $\lim _ { x \rightarrow 0 } g ( t )$

Use the definition of area as a limit to find the area of the region that lies under the graph of $f ( x ) = 3 x ^ { 2 } + 2 x$ over the interval $0 \leq x \leq 1$ .

Evaluate $\lim _ { x \rightarrow 1 } \frac { 1 - x ^ { 2 } } { 1 - x ^ { 3 } }$ .

Find the equation of the tangent line to the curve $y =$ $\sqrt { x }$ at the point $( 4,2 )$ .

Find $\lim _ { x \rightarrow \infty } \frac { 3 x } { 2 x ^ { 3 } - 1 }$ .