Exam 8: Estimation and Confidence Intervals

The Sugar Producers Association wants to estimate the mean yearly sugar consumption. A sample of 16 people reveals the mean yearly consumption to be 27 kg with a sample standard deviation of 9 kg. Assume a normal population. For a 90% confidence interval, what is the critical value needed?

i. The higher the degree of confidence, the larger the sample required to give a certain precision. ii. To determine the size of a sample, the standard deviation of the population must be estimated by either taking a pilot survey or by approximating it based on knowledge of the population. iii. One factor in determining the size of a sample is the maximum allowable error that you must decide on. It is the maximum error you will tolerate at a specified level of confidence.

There are 2,000 eligible voters in a precinct. Despite protests from knowledgeable persons that a sample size of 500 was too large in relation to the total, the 500 selected at random were asked to indicate whether they planned to vote for the Liberal incumbent or the Conservative challenger. Of the 500 surveyed, 350 said they were going to vote for the Liberal incumbent. Using the 0.99 confidence coefficient, what are the confidence limits for the proportion that plan to vote for the Liberal incumbent?

A survey of 25 grocery stores revealed that the average price of a 4-litre bag of milk was $2.98 with a standard error of $0.10. What is the 95% confidence interval to estimate the true cost of a 4-litre bag of milk?

A sample mean is the best point estimate of the

Dr. Patton is a professor of English. Recently she counted the number of misspelled works in a group of student essays. She noted the distribution of misspelled words per essay followed the normal distribution with a standard deviation of 2.44 words per essay. For her Tuesday class of 50 students, the mean number of misspelled words per essay was 6.05. Construct a 90% confidence interval for the mean number of misspelled words in the population of student essays.

(i. Reasoning from a sample or small group to the entire group or population is called statistical inference. (ii) A 95 percent confidence interval implies that about 95 out of 100 similarly constructed intervals will include the parameter being estimated. (iii) For a sampling distribution of the means, 95% percent of the means would be between $\pm$ 1.96 standard deviations.

The following summarizes the average price of Air Canada stock at the end of 20 randomly selected weeks in 2000. Determine a 90% confidence interval for the average Air Canada stock price in 2000.

Which of the following would be used as a point estimate for the population mean (µ)?

A sample of 100 students is selected from a known population of 1,000 students to construct a 95% confidence interval for the average SAT score. What correction factor should be used to compute the standard error?

A pharmaceutical company wanted to estimate the population mean of monthly sales for their 250 sales people. Forty sales people were randomly selected. Their mean monthly sales were $10,000 with a standard deviation of $1,000. Construct a 95% confidence interval for the population mean.

A manager of a local store wants to estimate the mean amount spent per shopping visit by customers. Summary statistics from a sample taken reveal the following: Determine a 95% confidence interval for the mean amount spent.

The sample size needed to estimate a population mean within 2 units with a 95% confidence when the population standard deviation equals 8 is

A student wanted to quickly construct a 95% confidence interval for the average age of students in her statistics class. She randomly selected 9 students. Their average age was 19.1 years with a standard deviation of 1.5 years. What is the best point estimate for the population mean?

The following summarizes the amount of snowfall in Ontario over the past number of years. 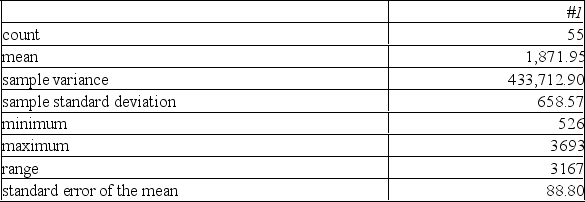You wonder whether the population mean could have been 2000 or 1900.

A financial analyst wanted to determine the mean annual return on mutual funds. A random sample of 60 returns shows a mean of 12%. If the population standard deviation is assumed to be 4%, estimate with 95% confidence the mean annual return on all mutual funds.

The t distribution approaches __________ as the sample size increases. As the sample size increases, the computed value of t ______________.

The Sugar Producers Association wants to estimate the mean yearly sugar consumption. A sample of 25 people reveals the mean yearly consumption to be 27 kg with a sample standard deviation of 9 kg. Assume a normal population. For a 99% confidence interval, what is the critical value needed?

i. The Student t distribution has a greater spread than does the z distribution. As a result, the critical values of t for a given level of significance are larger in magnitude than the corresponding z critical values. ii. The test statistic t has n-1 degrees of freedom. iii. William S. Gosset, a brewmaster, developed the t test for the Guinness Brewery in Ireland, who published it in 1908 using the pen name "Student."

The Kennel Club wants to estimate the proportion of children that have a dog as a pet. Assume a 95% level of confidence and that the club estimates that 30% of the children have a dog as a pet. If the club wants the estimate to be within 3% of the population proportion, how many children would they need to contact?