Exam 2: Introduction to Optimization and Linear Programming

Some resources (i.e. meat and dairy products, pharmaceuticals, a can of paint) are perishable. This means that once a package (e.g. a can or a bag) is open the content should be used in its entirety. Which of the following constraints reflects this fact?

Mathematical programming is referred to as

Solve the following LP problem graphically by enumerating the corner points.

If there is no way to simultaneously satisfy all the constraints in an LP model the problem is said to be

A common objective in the product mix problem is

The constraint for resource 1 is 5 X₁ + 4 X₂ ≥ 200. If X₂ = 20, what it the minimum value for X₁?

For an infeasible problem, the feasible region:

Limited resources are modeled in optimization problems as

Why do we study the graphical method of solving LP problems?

Level curves are used when solving LP models using the graphical method. To what part of the model do level curves relate?

The first step in formulating a linear programming problem is

Solve the following LP problem graphically using level curves.

Project 2.1 Joey Koons runs a small custom computer parts company. As a sideline he offers customized and pre-built computer system packages. In preparation for the upcoming school year, he has decided to offer two custom computer packages tailored for what he believes are current student needs. System A provides a strong computing capability at a reasonable cost while System B provides a much more powerful computing capability, but at a higher cost. Joey has a fairly robust parts inventory but is concerned about his stock of those components that are common to each proposed system. A portion of his inventory, the item cost, and inventory level is provided in the table below. 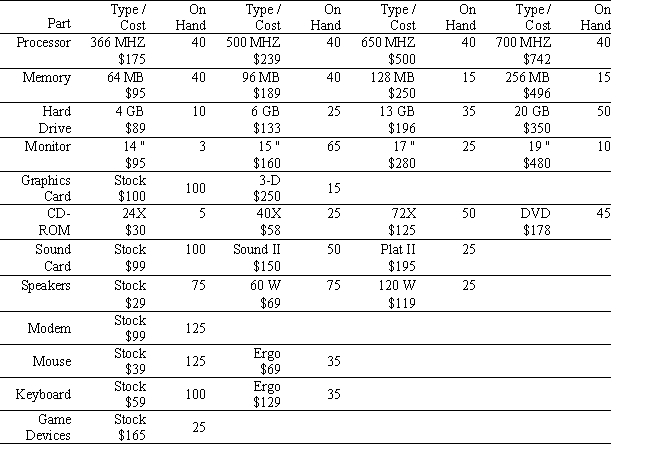The requirements for each system are provided in the following table: 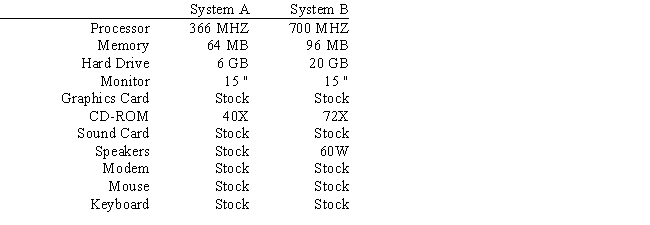Each system requires assembly, testing and packaging. The requirements per system built and resources available are summarized in the table below. Joey is uncertain about product demand. In the past he has put together similar types of computer packages but his sales results vary. As a result is unwilling to commit all his in-house labor force to building the computer packages. He is confident he can sell all he can build and is not overly concerned with lost sales due to stock-outs. Based on his market survey, he has completed his advertising flyer and will offer System A for $ 1250 and will offer system B for $ 2325. Joey now needs to let his workers know how many of each system to build and he wants that mix to maximize his profits. Formulate an LP for Dave's problem. Solve the model using the graphical method. What is Dave's preferred product mix? What profit does Dave expect to make from this product mix?

The second step in formulating a linear programming problem is

When do alternate optimal solutions occur in LP models?

Solve the following LP problem graphically by enumerating the corner points.