Exam 11: Rolling, Torque, and Angular Momentum
Exam 1: Measurement37 Questions
Exam 2: Motion Along a Straight Line90 Questions
Exam 3: Vector37 Questions
Exam 4: Motion in Two and Three Dimensions56 Questions
Exam 5: Force and Motion I73 Questions
Exam 6: Force and Motion II74 Questions
Exam 7: Kinetic Energy and Work73 Questions
Exam 8: Potential Energy and Conservation of Energy63 Questions
Exam 9: Center of Mass and Linear Momentum99 Questions
Exam 10: Rotation102 Questions
Exam 11: Rolling, Torque, and Angular Momentum66 Questions
Exam 12: Equilibrium and Elasticity57 Questions
Exam 13: Gravitation55 Questions
Exam 14: Fluids88 Questions
Exam 15: Oscillations75 Questions
Exam 16: Waves I82 Questions
Exam 17: Waves II71 Questions
Exam 18: Temperature, Heat, and the First Law of Thermodynamics96 Questions
Exam 19: The Kinetic Theory of Gases113 Questions
Exam 20: Entropy and the Second Law of Thermodynamics61 Questions
Exam 21: Electric Charge52 Questions
Exam 22: Electric Fields55 Questions
Exam 23: Gauss Law38 Questions
Exam 24: Electric Potential52 Questions
Exam 25: Capacitance61 Questions
Exam 26: Current and Resistance55 Questions
Exam 27: Circuits73 Questions
Exam 28: Magnetic Fields55 Questions
Exam 29: Magnetic Fields Due to Currents49 Questions
Exam 30: Induction and Inductance90 Questions
Exam 31: Electromagnetic Oscillations and Alternating Current88 Questions
Exam 32: Maxwells Equations; Magnetism of Matter81 Questions
Exam 33: Electromagnetic Waves83 Questions
Exam 34: Images79 Questions
Exam 35: Interference46 Questions
Exam 36: Diffraction77 Questions
Exam 37: Relativity68 Questions
Exam 38: Photons and Matter Waves57 Questions
Exam 39: More About Matter Waves41 Questions
Exam 40: All About Atoms79 Questions
Exam 41: Conduction of Electricity in Solids51 Questions
Exam 42: Nuclear Physics68 Questions
Exam 43: Energy From the Nucleus50 Questions
Exam 44: Quarks, Leptons, and the Big Bang55 Questions
Select questions type
A particle moves along the x axis. In order to calculate the torque on the particle, you need to know:
(Multiple Choice)
4.9/5  (32)
(32)
When the speed of a rear-drive car is increasing on a horizontal road the direction of the frictional force on the tires is:
(Multiple Choice)
4.7/5  (31)
(31)
A solid sphere and a solid cylinder of equal mass and radius are simultaneously released from rest on the same inclined plane sliding down the incline. Then:
(Multiple Choice)
4.8/5  (41)
(41)
A yo-yo, arranged as shown, rests on a frictionless surface. When a force is applied to the string as shown, the yo-yo: 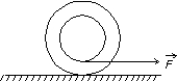
(Multiple Choice)
4.8/5  (32)
(32)
A solid wheel with mass M, radius R, and rotational inertia MR2/2, rolls without sliding on a horizontal surface. A horizontal force F is applied to the axle and the center of mass has an acceleration a. The magnitudes of the applied force F and the frictional force f of the surface, respectively, are:
(Multiple Choice)
4.9/5  (33)
(33)
A single force acts on a particle P. Rank each of the orientations of the force shown below according to the magnitude of the time rate of change of the particle's angular momentum about the point O, least to greatest. 
(Multiple Choice)
4.8/5  (31)
(31)
The angular momentum vector of Earth, due to its daily rotation, is directed:
(Multiple Choice)
4.7/5  (35)
(35)
A man, holding a weight in each hand, stands at the center of a horizontal frictionless rotating turntable. The effect of the weights is to double the rotational inertia of the system. As he is rotating, the man opens his hands and drops the two weights. They fall outside the turntable. Then:
(Multiple Choice)
4.8/5  (31)
(31)
A wheel of radius 0.5 m rolls without sliding on a horizontal surface as shown. Starting from rest, the wheel moves with constant angular acceleration 6 rad/s2. The distance in traveled by the center of the wheel from t = 0 to t = 3 s is: 
(Multiple Choice)
4.9/5  (36)
(36)
As a 2.0-kg block travels around a 0.50-m radius circle it has an angular speed of 12 rad/s. The circle is parallel to the xy plane and is centered on the z axis, 0.75 m from the origin. The magnitude of its angular momentum around the origin is:
(Multiple Choice)
4.7/5  (34)
(34)
A particle is located on the x axis at x = 2.0 m from the origin. A force of 25 N, directed 30° above the x axis in the x-y plane, acts on the particle. What is the torque about the origin on the particle?
(Multiple Choice)
4.9/5  (34)
(34)
A solid sphere starts from rest and rolls down a slope that is 5.1 m long. If its speed at the bottom of the slope is 4.3 m/s, what is the angle of the slope?
(Multiple Choice)
4.8/5  (35)
(35)
Two uniform cylinders have different masses and different rotational inertias. They simultaneously start from rest at the top of an inclined plane and roll without sliding down the plane. The cylinder that gets to the bottom first is:
(Multiple Choice)
4.8/5  (33)
(33)
A rod rests on frictionless ice. Forces that are equal in magnitude and opposite in direction are simultaneously applied to its ends as shown. The quantity that has a magnitude of zero is its: 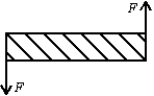
(Multiple Choice)
4.8/5  (25)
(25)
A playground merry-go-round has a radius of 3.0 m and a rotational inertia of 600 kg∙m2. It is initially spinning at 0.80 rad/s when a 20-kg child crawls from the center to the rim. When the child reaches the rim the angular velocity of the merry-go-round is:
(Multiple Choice)
4.9/5  (43)
(43)
A simple gyroscope consists of a wheel (M = 2.0 kg, R = 0.75 m, I = 1.1 kg∙m2) rotating on a horizontal shaft. If the shaft is balanced on a pivot 0.50 m from the wheel, and the wheel rotates at 500 rev/min, what is the precession frequency of the wheel?
(Multiple Choice)
4.9/5  (35)
(35)
A playground merry-go-round has a radius R and a rotational inertia I. When the merry-go-round is at rest, a child with mass m runs with speed v along a line tangent to the rim and jumps on. The angular velocity of the merry-go-round is then:
(Multiple Choice)
4.7/5  (30)
(30)
A wheel rolls without slipping along a horizontal road as shown. The velocity of the center of the wheel is represented by . Point P is painted on the rim of the wheel. The direction of the instantaneous velocity of point P is: 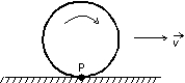
(Multiple Choice)
4.9/5  (29)
(29)
A simple gyroscope consists of a wheel (R = 0.75 m, I = 0.9 MR2) rotating on a horizontal shaft. If the shaft is balanced on a pivot 0.50 m from the wheel, and the wheel rotates at 500 rev/min, what is the precession frequency of the wheel?
(Multiple Choice)
4.8/5  (30)
(30)
Showing 21 - 40 of 66
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)