Exam 12: Computer Simulation: Basic Concepts
Exam 1: Introduction54 Questions
Exam 2: Linear Programming: Basic Concepts85 Questions
Exam 3: Linear Programming: Formulation and Applications76 Questions
Exam 4: The Art of Modeling With Spreadsheets75 Questions
Exam 5: What-If Analysis for Linear Programming75 Questions
Exam 6: Network Optimization Problems84 Questions
Exam 7: Using Binary Integer Programming to Deal With Yes-Or-No Decisions76 Questions
Exam 8: Nonlinear Programming75 Questions
Exam 9: Decision Analysis80 Questions
Exam 10: Forecasting77 Questions
Exam 11: Queueing Models78 Questions
Exam 12: Computer Simulation: Basic Concepts79 Questions
Exam 13: Computer Simulation With Analytic Solver77 Questions
Select questions type
The Queueing Simulator returned the results shown below. Which of the following waiting times in the queue is most likely to occur? 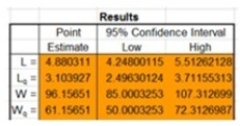
(Multiple Choice)
4.9/5  (42)
(42)
After some experimentation, you have determined that you have a biased coin. The probability of heads is 0.6 and the probability of tails is 0.4. What is the probability that you will observed heads on three successive flips?
(Multiple Choice)
4.9/5  (33)
(33)
You are reviewing a simulation model and find that the analyst who prepared the model used the formula = 100 + (175 − 100) × RAND() to generate the simulated selling price of a product. Which of the following assumptions did the analyst make about the selling price of the product?
I. The minimum price of the product is 175.
II. The maximum price of the product is 175.
III. The cost of the product is normally distributed.
(Multiple Choice)
4.9/5  (35)
(35)
You have determined that waiting times at a toll booth are uniformly distributed over the interval 20 to 60 seconds. The first random number your simulation returns is 0.4732. What is the waiting time that this random number generates?
(Multiple Choice)
4.9/5  (35)
(35)
Given this frequency distribution, the random number 0.2258 would be interpreted as a demand of:
Demand Frequency 0 38 1 22 2 22 3 18
(Multiple Choice)
4.8/5  (37)
(37)
A stochastic system is one that evolved over time according to a continuous probability distribution.
(True/False)
4.9/5  (36)
(36)
Over the course of an eight hour day, a business normally has about 200 customers arrive. Assuming that the customers arrive at about the same rate over the entire day, what is the interarrival time?
(Multiple Choice)
4.9/5  (28)
(28)
Which of the following is NOT a step in the process of conducting a simulation study?
(Multiple Choice)
4.7/5  (34)
(34)
One key advantage of computer simulation is that is makes full use of the simplifying approximations that are available.
(True/False)
4.8/5  (31)
(31)
Customers arrive at a carwash with on average once every 20 minutes. It seems likely that customer arrivals follow an exponential distribution. In a simulation, the random number 0.1398 is generated. How long it will be until the next simulated arrival occurs?
(Multiple Choice)
4.9/5  (31)
(31)
Simulation is often the first choice of decision makers instead of analytic models.
(True/False)
4.7/5  (36)
(36)
Service times at a doctor's office take an average of 40 minutes. It seems likely that service times follow an exponential distribution. In a simulation, what formula would you use to estimate how long the next service will take?
(Multiple Choice)
4.8/5  (36)
(36)
Simulation enables a decision maker to experiment with a system and observe its behavior.
(True/False)
4.8/5  (31)
(31)
The Queueing Simulator returned the results shown below. Which of the following waiting times in the queue is most likely to occur? 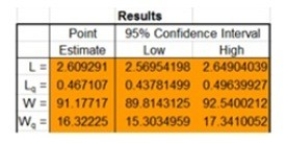
(Multiple Choice)
4.8/5  (36)
(36)
If a simulation begins with the first random number, the first simulated value would be:
Random numbers: 0.6246, 0.2594, 0.4055
Demand Frequency 0 0.15 1 0.30 2 0.25 3 0.15 4 0.15
(Multiple Choice)
4.9/5  (35)
(35)
When dealing with relatively complex systems, computer simulation is an inexpensive option for decision makers.
(True/False)
4.9/5  (27)
(27)
In order to generate more accurate results when using simulation:
(Multiple Choice)
4.9/5  (25)
(25)
Service times at a doctor's office take an average of 40 minutes. It seems likely that service times follow an exponential distribution. In a simulation, the random number 0.7588 is generated. How long it will be until the next simulated arrival occurs?
(Multiple Choice)
4.9/5  (28)
(28)
Note: This question requires the use of the Queueing Simulator spreadsheet in Excel. Use the queueing simulator to simulate a queueing system with 3 servers, uniform interarrival times between 20 and 50 seconds and exponential service times with an average of 75 seconds. Use a simulation run length of 10,000 arrivals. If you observe the system at a random time, what is the probability that there will be 4 or more customers waiting in line?
(Multiple Choice)
4.7/5  (45)
(45)
You are reviewing a simulation model and find that the analyst who prepared the model used the formula =NORM.INV(RAND(),100,5) to generate the waiting time at a restaurant (in seconds). Which of the following assumptions did the analyst make about the waiting time?
I. The minimum waiting time is 100.
II. The average (mean) waiting time is 100.
III. The waiting time is normally distributed.
(Multiple Choice)
4.9/5  (37)
(37)
Showing 41 - 60 of 79
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)