The Log-Log and Exponential Models,ln(x)= β 0 + β 1 ln(x)+ ε and (Y)=

The Answer of The Log-Log and Exponential Models,ln(x)= β 0 + β 1 ln(x)+ ε and (Y)=

The Log-Log and Exponential Models,ln(x)= β 0 + β 1 ln(x)+ ε and (Y)=

The Answer of The Log-Log and Exponential Models,ln(x)= β 0 + β 1 ln(x)+ ε and (Y)=

Solved

The Log-Log and Exponential Models,ln(x)= β₀ + β₁ln(x)+ ε and (Y)=

Question 35

Multiple Choice

The log-log and exponential models,ln(x) = β₀ + β₁ln(x) + ε and (y) = β₀ + β₁x + ε,were used to fit given data on y and x,and the following table summarizes the regression results.Which of the two models provides a better fit?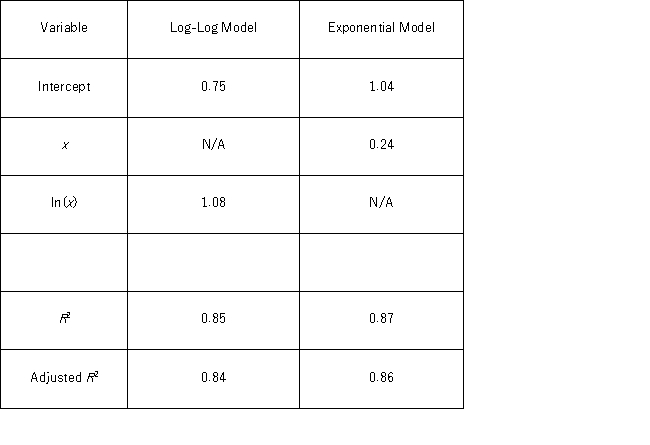

A) The log-log model.
B) The exponential model.
C) The models are not comparable.
D) The provided information is not sufficient to make the conclusion.

The Log-Log and Exponential Models,ln(x)= β0 + β1ln(x)+ ε and (Y)=

Multiple Choice

Correct Answer:VerifiedUnlock this answer nowGet Access to more Verified Answers free of chargeAccess For Free

The Log-Log and Exponential Models,ln(x)= β₀ + β₁ln(x)+ ε and (Y)=

Correct Answer:
Verified
Unlock this answer now
Get Access to more Verified Answers free of charge