Exam 9: Fundamentals of Hypothesis Testing: One-Sample Tests

TABLE 9-7 A major home improvement store conducted its biggest brand recognition campaign in the company's history.A series of new television advertisements featuring well-known entertainers and sports figures were launched.A key metric for the success of television advertisements is the proportion of viewers who "like the ads a lot".A study of 1,189 adults who viewed the ads reported that 230 indicated that they "like the ads a lot." The percentage of a typical television advertisement receiving the "like the ads a lot" score is believed to be 22%.Company officials wanted to know if there is evidence that the series of television advertisements are less successful than the typical ad at a 0.01 level of significance. -Referring to Table 9-7,the null hypothesis will be rejected if the test statistic is

TABLE 9-9 The president of a university claimed that the entering class this year appeared to be larger than the entering class from previous years but their mean SAT score is lower than previous years.He took a sample of 20 of this year's entering students and found that their mean SAT score is 1,501 with a standard deviation of 53.The university's record indicates that the mean SAT score for entering students from previous years is 1,520.He wants to find out if his claim is supported by the evidence at a 5% level of significance. -True or False: Referring to Table 9-9,if these data were used to perform a two-tail test,the p-value would be 0.1254.

How many tissues should the Kimberly Clark Corporation package of Kleenex contain? Researchers determined that 60 tissues is the mean number of tissues used during a cold.Suppose a random sample of 100 Kleenex users yielded the following data on the number of tissues used during a cold: 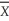= 52, S = 22.Give the null and alternative hypotheses to determine if the number of tissues used during a cold is less than 60.

How many tissues should the Kimberly Clark Corporation package of Kleenex contain? Researchers determined that 60 tissues is the mean number of tissues used during a cold.Suppose a random sample of 100 Kleenex users yielded the following data on the number of tissues used during a cold: 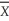= 52, S = 22.Using the sample information provided,calculate the value of the test statistic.

TABLE 9-2 A student claims that he can correctly identify whether a person is a business major or an agriculture major by the way the person dresses.Suppose in actuality that if someone is a business major,he can correctly identify that person as a business major 87% of the time.When a person is an agriculture major,the student will incorrectly identify that person as a business major 16% of the time.Presented with one person and asked to identify the major of this person (who is either a business or an agriculture major),he considers this to be a hypothesis test with the null hypothesis being that the person is a business major and the alternative that the person is an agriculture major. -Referring to Table 9-2,what is the power of the test?

Which of the following would be an appropriate alternative hypothesis?

TABLE 9-9 The president of a university claimed that the entering class this year appeared to be larger than the entering class from previous years but their mean SAT score is lower than previous years.He took a sample of 20 of this year's entering students and found that their mean SAT score is 1,501 with a standard deviation of 53.The university's record indicates that the mean SAT score for entering students from previous years is 1,520.He wants to find out if his claim is supported by the evidence at a 5% level of significance. -Referring to Table 9-9,state the null hypothesis for this study.

A major Blu-ray rental chain is considering opening a new store in an area that currently does not have any such stores.The chain will open if there is evidence that more than 5,000 of the 20,000 households in the area are equipped with Blu-ray players.It conducts a telephone poll of 300 randomly selected households in the area and finds that 96 have Blu-ray players.The rental chain's conclusion from the hypothesis test using a 5% level of significance is

TABLE 9-6 The quality control engineer for a furniture manufacturer is interested in the mean amount of force necessary to produce cracks in stressed oak furniture.She performs a two-tail test of the null hypothesis that the mean for the stressed oak furniture is 650.The calculated value of the Z test statistic is a positive number that leads to a p-value of 0.080 for the test. -True or False: Referring to Table 9-6,suppose the engineer had decided that the alternative hypothesis to test was that the mean was greater than 650.Then if the test is performed with a level of significance of 0.05,the null hypothesis would be rejected.

For a given level of significance (α),if the sample size n is increased,the probability of a Type II error (β)

The symbol for the probability of committing a Type II error of a statistical test is

TABLE 9-7 A major home improvement store conducted its biggest brand recognition campaign in the company's history.A series of new television advertisements featuring well-known entertainers and sports figures were launched.A key metric for the success of television advertisements is the proportion of viewers who "like the ads a lot".A study of 1,189 adults who viewed the ads reported that 230 indicated that they "like the ads a lot." The percentage of a typical television advertisement receiving the "like the ads a lot" score is believed to be 22%.Company officials wanted to know if there is evidence that the series of television advertisements are less successful than the typical ad at a 0.01 level of significance. -Referring to Table 9-7,state the alternative hypothesis for this study.

TABLE 9-1 Microsoft Excel was used on a set of data involving the number of defective items found in a random sample of 46 cases of light bulbs produced during a morning shift at a plant.A manager wants to know if the mean number of defective bulbs per case is greater than 20 during the morning shift.She will make her decision using a test with a level of significance of 0.10.The following information was extracted from the Microsoft Excel output for the sample of 46 cases: -True or False: Referring to Table 9-1,the manager can conclude that there is sufficient evidence to show that the mean number of defective bulbs per case is greater than 20 during the morning shift using a level of significance of 0.10.

A survey claims that 9 out of 10 doctors recommend aspirin for their patients with headaches.To test this claim against the alternative that the actual proportion of doctors who recommend aspirin is less than 0.90,a random sample of 100 doctors was selected.Suppose that the test statistic is -2.20.Can you conclude that H₀ should be rejected at the (a)α = 0.10,(b)α = 0.05,and (c)α = 0.01 level of Type I error?

True or False: Referring to Table 9-1,the manager can conclude that there is sufficient evidence to show that the mean number of defective bulbs per case is greater than 20 during the morning shift with no more than a 1% probability of incorrectly rejecting the true null hypothesis.

TABLE 9-9 The president of a university claimed that the entering class this year appeared to be larger than the entering class from previous years but their mean SAT score is lower than previous years.He took a sample of 20 of this year's entering students and found that their mean SAT score is 1,501 with a standard deviation of 53.The university's record indicates that the mean SAT score for entering students from previous years is 1,520.He wants to find out if his claim is supported by the evidence at a 5% level of significance. -True or False: Referring to Table 9-9,the president can conclude that the mean SAT score of the entering class this year is lower than previous years using a level of significance of 0.10.

An entrepreneur is considering the purchase of a coin-operated laundry.The current owner claims that over the past 5 years,the mean daily revenue was $675 with a population standard deviation of $75.A sample of 30 days reveals a daily mean revenue of $625.If you were to test the null hypothesis that the daily mean revenue was $675,which test would you use?

True or False: A proper methodology in performing hypothesis tests is to ask whether a random sample can be selected from the population of interest.

If a researcher rejects a false null hypothesis,she has made a(n)________ decision.

TABLE 9-6 The quality control engineer for a furniture manufacturer is interested in the mean amount of force necessary to produce cracks in stressed oak furniture.She performs a two-tail test of the null hypothesis that the mean for the stressed oak furniture is 650.The calculated value of the Z test statistic is a positive number that leads to a p-value of 0.080 for the test. -Referring to Table 9-6,suppose the engineer had decided that the alternative hypothesis to test was that the mean was greater than 650.What would be the p-value of this one-tail test?