Exam 20: Minimal Spanning Tree
Exam 1: Introduction49 Questions
Exam 2: An Introduction to Linear Programming52 Questions
Exam 3: Linear Programming: Sensitivity Analysis and Interpretation of Solution47 Questions
Exam 4: Linear Programming Applications in Marketing, Finance and Operations Management38 Questions
Exam 5: Advanced Linear Programming Applications35 Questions
Exam 6: Distribution and Network Problems54 Questions
Exam 7: Integer Linear Programming43 Questions
Exam 8: Nonlinear Optimization Models48 Questions
Exam 9: Project Scheduling: Pertcpm44 Questions
Exam 10: Inventory Models51 Questions
Exam 11: Waiting Line Models48 Questions
Exam 12: Simulation49 Questions
Exam 13: Decision Analysis42 Questions
Exam 14: Multicriteria Decisions45 Questions
Exam 15: Forecasting47 Questions
Exam 16: Markov Processes41 Questions
Exam 17: Linear Programming: Simplex Method46 Questions
Exam 18: Simplex-Based Sensitivity Analysis and Duality34 Questions
Exam 19: Solution Procedures for Transportation and Assignment Problems42 Questions
Exam 20: Minimal Spanning Tree18 Questions
Exam 21: Dynamic Programming30 Questions
Select questions type
Consider a minimal spanning tree problem in which pipe must be laid to connect sprinklers on a golf course. When represented with a network,
Free
(Multiple Choice)
4.9/5  (32)
(32)
Correct Answer:
A
The minimal spanning tree algorithm will lead to an optimal solution regardless of which node is chosen at the start of the algorithm.
Free
(True/False)
4.7/5  (28)
(28)
Correct Answer:
True
For the following eight cities with the given distances, find the minimal spanning tree path. To City From City 1 2 3 4 5 6 7 1 - 5 2 6 - - - 2 5 - - 4 6 - - 3 2 - - 3 - 12 - 4 6 4 3 - 9 8 - 5 - 6 - 9 - 0 14 6 - - 12 8 0 - 4 7 - - - - 14 4 -
Free
(Short Answer)
4.7/5  (29)
(29)
Correct Answer:
1-3-4-2-5-6-7
It is possible for minimal spanning tree problems to have alternative optimal solutions.
(True/False)
4.7/5  (31)
(31)
The minimum spanning tree allows a person to visit every node without backtracking.
(True/False)
4.8/5  (41)
(41)
The minimal spanning tree algorithm has connected nodes 8 and 9. Node 8 could be connected to nodes 11 (distance 6) and 12 (distance 5) and node 9 could be connected to node 12 (distance 3) and node 13 (distance 2). Which will you do next?
(Multiple Choice)
4.7/5  (37)
(37)
For a network consisting of N nodes, a minimal spanning tree will consist of:
(Multiple Choice)
4.8/5  (37)
(37)
What are some other criteria (measures), besides distance, that might be minimized in a minimal spanning tree problem? Provide an example situation for each criterion.
(Essay)
4.9/5  (35)
(35)
The arcs in a minimal spanning tree problem can be measured in terms of criteria other than distance.
(True/False)
5.0/5  (32)
(32)
Cases in which a greedy algorithm provides the optimal solution are rare.
(True/False)
4.9/5  (28)
(28)
The numbers on this network represent times to distribute a message. Use the minimal spanning tree algorithm to determine how messages should be passed in order to minimize total time. 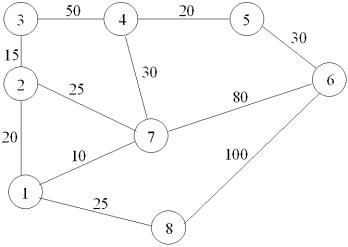
(Essay)
4.9/5  (33)
(33)
In the minimal spanning tree algorithm, you must consider the unconnected nodes that can be reached from any of the connected nodes, rather than arbitrarily considering only one of the connected nodes.
(True/False)
4.9/5  (42)
(42)
What is a greedy algorithm? What is an example of a greedy algorithm?
(Short Answer)
4.9/5  (39)
(39)
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)