Exam 13: Simple Linear Regression
Exam 1: Defining and Collecting Data202 Questions
Exam 2: Organizing and Visualizing256 Questions
Exam 3: Numerical Descriptive Measures217 Questions
Exam 4: Basic Probability167 Questions
Exam 5: Discrete Probability Distributions165 Questions
Exam 6: The Normal Distribution and Other Continuous Distributions170 Questions
Exam 7: Sampling Distributions165 Questions
Exam 8: Confidence Interval Estimation219 Questions
Exam 9: Fundamentals of Hypothesis Testing: One-Sample Tests194 Questions
Exam 10: Two-Sample Tests240 Questions
Exam 11: Analysis of Variance170 Questions
Exam 12: Chi-Square and Nonparametric188 Questions
Exam 13: Simple Linear Regression243 Questions
Exam 14: Introduction to Multiple394 Questions
Exam 15: Multiple Regression146 Questions
Exam 16: Time-Series Forecasting235 Questions
Exam 17: Getting Ready to Analyze Data386 Questions
Exam 18: Statistical Applications in Quality Management159 Questions
Exam 19: Decision Making126 Questions
Exam 20: Probability and Combinatorics421 Questions
Select questions type
SCENARIO 13-7
An investment specialist claims that if one holds a portfolio that moves in the opposite direction to the
market index like the S&P 500, then it is possible to reduce the variability of the portfolio's return. In
other words, one can create a portfolio with positive returns but less exposure to risk.
A sample of 26 years of S&P 500 index and a portfolio consisting of stocks of private prisons, which
are believed to be negatively related to the S&P 500 index, is collected. A regression analysis was
performed by regressing the returns of the prison stocks portfolio (Y) on the returns of S&P 500 index
(X) to prove that the prison stocks portfolio is negatively related to the S&P 500 index at a 5% level
of significance. The results are given in the following EXCEL output. Coefficients \bullet Standard Error \bullet T Stat \bullet P- Coefficients Standard Error T Stat P-value Intercept 4.8660 0.3574 13.6136 0.0000 S\&P -0.5025 0.0716 -7.0186 0.0000
-Referring to Scenario 13-7, to test whether the prison stocks portfolio is negatively related to the S&P 500 index, the appropriate null and alternative hypotheses are, respectively, a) vs.
b) vs.
c) vs.
d) vs.
(Short Answer)
4.8/5  (30)
(30)
SCENARIO 13-14-B
You are the CEO of a dairy company. You are planning to expand production by purchasing
additional cows, lands and hiring more workers. From the existing 50 farms owned by the company,
you have collected data on total milk production (in liters) and the number of milking cows. The data
are shown below and also available in the Excel file Scenario13-14-DataB.XLSX. MILK 84686 101876 103248 70508 76072 86615 87508 105195 120351 68658 85478 91902 110374 125364 159401 102883 113151 133297 140073 145434 152513 128275 138040 161276 208079 224119 231071 122114 132222 155092 177273 196399 205329 89564 94838 94920 97577 102163 103754 239585 239773 241293 249157 294388 318813 66462 100444 103846 154118 155460 COWS 21 20 22 17 16 20 21 19 21 19 22 24 26 26 27 22 27 24 27 24 29 21 25 26 33 31 33 23 27 29 27 28 32 22 26 24 26 28 26 39 44 42 41 42 47 18 21 22 27 27 You believe that the number of milking cows is the best predictor for total milk production on any
given farm.
-Referring to Scenario 13-14-B, if you purchase 40 cows for the new farm, the lower and upper
limit of the 95% prediction interval for the total milk production are _____ liters and _____ liters,
respectively.
(Short Answer)
4.9/5  (34)
(34)
SCENARIO 13-5
The managing partner of an advertising agency believes that his company's sales are related to the
industry sales. He uses Microsoft Excel to analyze the last 4 years of quarterly data with
the following results: 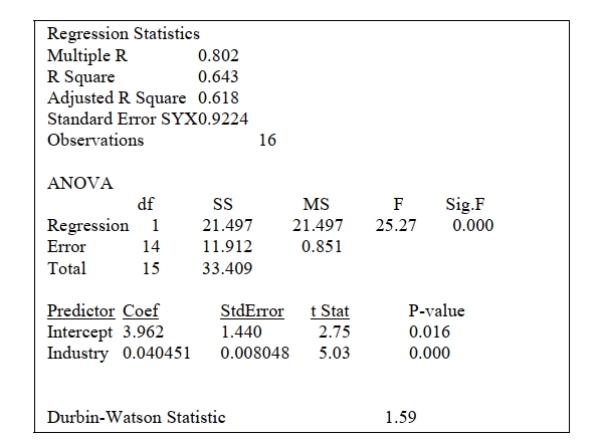 -Referring to Scenario 13-5, the standard error of the estimated slope coefficient is ________.
-Referring to Scenario 13-5, the standard error of the estimated slope coefficient is ________.
(Short Answer)
4.9/5  (33)
(33)
SCENARIO 13-3
The director of cooperative education at a state college wants to examine the effect of cooperative
education job experience on marketability in the work place. She takes a random sample of 4
students. For these 4, she finds out how many times each had a cooperative education job and how
many job offers they received upon graduation. These data are presented in the table below. Student CoopJobs JobOffer 1 1 4 2 2 6 3 1 3 4 0 1
-Referring to Scenario 13-3, set up a scatter plot.
(Essay)
4.8/5  (30)
(30)
Testing for the existence of correlation is equivalent to a) testing for the existence of the slope .
b) testing for the existence of the -intercept .
c) the confidence interval estimate for predicting .
d) None of the above.
(Short Answer)
4.8/5  (34)
(34)
EXPLANATION: The t-test statistic is KEYWORDS: t test on slope, p-value, slope
SCENARIO 13-4
The managers of a brokerage firm are interested in finding out if the number of new clients a broker
brings into the firm affects the sales generated by the broker. They sample 12 brokers and determine
the number of new clients they have enrolled in the last year and their sales amounts in thousands of
dollars. These data are presented in the table that follows. 1 27 52 2 11 37 3 42 64 4 33 55 5 15 29 6 15 34 7 25 58 8 36 59 9 28 44 10 30 48 11 17 31 12 22 38
-Referring to Scenario 13-4, the managers of the brokerage firm wanted to test the hypothesis that
the population slope was equal to 0. The p-value of the test is ________.
(Essay)
4.9/5  (37)
(37)
SCENARIO 13-3
The director of cooperative education at a state college wants to examine the effect of cooperative
education job experience on marketability in the work place. She takes a random sample of 4
students. For these 4, she finds out how many times each had a cooperative education job and how
many job offers they received upon graduation. These data are presented in the table below. Student CoopJobs JobOffer 1 1 4 2 2 6 3 1 3 4 0 1
-Referring to Scenario 13-3, suppose the director of cooperative education wants to construct a
95% prediction interval for the number of job offers received by a student who has had exactly
two cooperative education jobs. The prediction interval is from ________ to ________.
(Short Answer)
4.8/5  (36)
(36)
EXPLANATION: The t-test statistic is KEYWORDS: t test on slope, p-value, slope
SCENARIO 13-4
The managers of a brokerage firm are interested in finding out if the number of new clients a broker
brings into the firm affects the sales generated by the broker. They sample 12 brokers and determine
the number of new clients they have enrolled in the last year and their sales amounts in thousands of
dollars. These data are presented in the table that follows. 1 27 52 2 11 37 3 42 64 4 33 55 5 15 29 6 15 34 7 25 58 8 36 59 9 28 44 10 30 48 11 17 31 12 22 38
-Referring to Scenario 13-4, suppose the managers of the brokerage firm want to construct a 99%
confidence interval estimate for the mean sales made by brokers who have brought into the firm
24 new clients. The confidence interval is from ________ to ________.
(Short Answer)
4.7/5  (37)
(37)
SCENARIO 13-12
The manager of the purchasing department of a large saving and loan organization would like to
develop a model to predict the amount of time (measured in hours) it takes to record a loan
application. Data are collected from a sample of 30 days, and the number of applications recorded and
completion time in hours is recorded. Below is the regression output: Regression Statistics Multiple R 0.9447 R Square 0.8924 Adjusted R 0.8886 Square Standard 0.3342 Error Observations 30
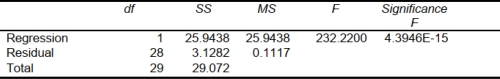 Coefficients Standard Error t Stat P-value Lower 95\% Upper 95\% Intercept 0.4024 0.1236 3.2559 0.0030 0.1492 0.6555 Applications 0.0126 0.0008 15.2388 0.0000 0.0109 0.0143
-Referring to Scenario 13-12, the value of the measured t-test statistic to test whether the amount of time depends linearly on the number of loan applications recorded is
Coefficients Standard Error t Stat P-value Lower 95\% Upper 95\% Intercept 0.4024 0.1236 3.2559 0.0030 0.1492 0.6555 Applications 0.0126 0.0008 15.2388 0.0000 0.0109 0.0143
-Referring to Scenario 13-12, the value of the measured t-test statistic to test whether the amount of time depends linearly on the number of loan applications recorded is
(Multiple Choice)
4.8/5  (29)
(29)
SCENARIO 13-14-B
You are the CEO of a dairy company. You are planning to expand production by purchasing
additional cows, lands and hiring more workers. From the existing 50 farms owned by the company,
you have collected data on total milk production (in liters) and the number of milking cows. The data
are shown below and also available in the Excel file Scenario13-14-DataB.XLSX. MILK 84686 101876 103248 70508 76072 86615 87508 105195 120351 68658 85478 91902 110374 125364 159401 102883 113151 133297 140073 145434 152513 128275 138040 161276 208079 224119 231071 122114 132222 155092 177273 196399 205329 89564 94838 94920 97577 102163 103754 239585 239773 241293 249157 294388 318813 66462 100444 103846 154118 155460 COWS 21 20 22 17 16 20 21 19 21 19 22 24 26 26 27 22 27 24 27 24 29 21 25 26 33 31 33 23 27 29 27 28 32 22 26 24 26 28 26 39 44 42 41 42 47 18 21 22 27 27 You believe that the number of milking cows is the best predictor for total milk production on any
given farm.
-Referring to Scenario 13-14-B, the degrees of freedom for the F test on whether the number of
milking cows is a good predictor for the total milk production are _________.
(Essay)
4.9/5  (34)
(34)
EXPLANATION: The t-test statistic is KEYWORDS: t test on slope, p-value, slope
SCENARIO 13-4
The managers of a brokerage firm are interested in finding out if the number of new clients a broker
brings into the firm affects the sales generated by the broker. They sample 12 brokers and determine
the number of new clients they have enrolled in the last year and their sales amounts in thousands of
dollars. These data are presented in the table that follows. 1 27 52 2 11 37 3 42 64 4 33 55 5 15 29 6 15 34 7 25 58 8 36 59 9 28 44 10 30 48 11 17 31 12 22 38
-Referring to Scenario 13-4, the managers of the brokerage firm wanted to test the hypothesis that
the number of new clients brought in had a positive impact on the amount of sales generated. At a
level of significance of 0.01, the decision that should be made implies that the number of new
clients brought in _____ (had or did not have) a positive impact on the amount of sales
generated.
(Short Answer)
4.9/5  (36)
(36)
SCENARIO 13-14-B
You are the CEO of a dairy company. You are planning to expand production by purchasing
additional cows, lands and hiring more workers. From the existing 50 farms owned by the company,
you have collected data on total milk production (in liters) and the number of milking cows. The data
are shown below and also available in the Excel file Scenario13-14-DataB.XLSX. MILK 84686 101876 103248 70508 76072 86615 87508 105195 120351 68658 85478 91902 110374 125364 159401 102883 113151 133297 140073 145434 152513 128275 138040 161276 208079 224119 231071 122114 132222 155092 177273 196399 205329 89564 94838 94920 97577 102163 103754 239585 239773 241293 249157 294388 318813 66462 100444 103846 154118 155460 COWS 21 20 22 17 16 20 21 19 21 19 22 24 26 26 27 22 27 24 27 24 29 21 25 26 33 31 33 23 27 29 27 28 32 22 26 24 26 28 26 39 44 42 41 42 47 18 21 22 27 27 You believe that the number of milking cows is the best predictor for total milk production on any
given farm.
-Referring to Scenario 13-14-B, if you purchase 40 cows for the new farm, its predicted total
milk production is _________ liters.
(Short Answer)
4.9/5  (37)
(37)
SCENARIO 13-11
A computer software developer would like to use the number of downloads (in thousands) for the trial
version of his new shareware to predict the amount of revenue (in thousands of dollars) he can make
on the full version of the new shareware. Following is the output from a simple linear regression
along with the residual plot and normal probability plot obtained from a data set of 30 different
sharewares that he has developed: 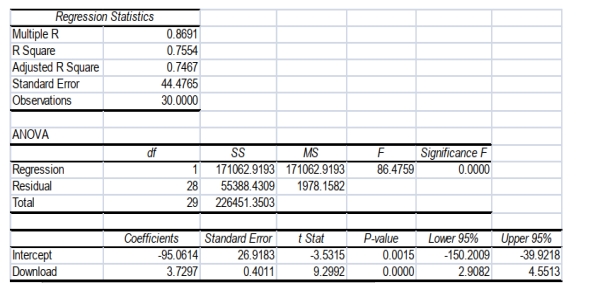
 -Referring to Scenario 13-11, what is the standard error of estimate?
-Referring to Scenario 13-11, what is the standard error of estimate?
(Short Answer)
4.9/5  (34)
(34)
SCENARIO 13-11
A computer software developer would like to use the number of downloads (in thousands) for the trial
version of his new shareware to predict the amount of revenue (in thousands of dollars) he can make
on the full version of the new shareware. Following is the output from a simple linear regression
along with the residual plot and normal probability plot obtained from a data set of 30 different
sharewares that he has developed: 

 -Referring to Scenario 13-11, what is the p-value for testing whether there is a linear relationship
between revenue and the number of downloads at a 5% level of significance?
-Referring to Scenario 13-11, what is the p-value for testing whether there is a linear relationship
between revenue and the number of downloads at a 5% level of significance?
(Short Answer)
4.7/5  (34)
(34)
SCENARIO 13-1
A large national bank charges local companies for using their services. A bank official reported the
results of a regression analysis designed to predict the bank's charges (Y) -- measured in dollars per
month -- for services rendered to local companies. One independent variable used to predict service
charges to a company is the company's sales revenue (X) -- measured in millions of dollars. Data for
21 companies who use the bank's services were used to fit the model:
The results of the simple linear regression are provided below.
-Referring to Scenario 13-1, interpret the estimate of ?, the standard deviation of the random error term (standard error of the estimate) in the model.
(Multiple Choice)
4.9/5  (42)
(42)
SCENARIO 13-8
It is believed that GPA (grade point average, based on a four point scale) should have a positive linear
relationship with ACT scores. Given below is the Excel output for predicting GPA using ACT scores
based a data set of 8 randomly chosen students from a Big-Ten university. Regressing GPA on ACT
Regression Statistics Multiple R 0.7598 R Square 0.5774 Adjusted R Square 0.5069 Standard Error 0.2691 Observations 8
ANOVA
df SS MS F Significance F Regression 1 0.5940 0.5940 8.1986 0.0286 Residual 6 0.4347 0.0724 Total 7 1.0287
Coefficients Standard Error t Stat P-value Lower 95\% Upper 95\% Intercept 0.5681 0.9284 0.6119 0.5630 -1.7036 2.8398 ACT 0.1021 0.0356 2.8633 0.0286 0.0148 0.1895
-Referring to Scenario 13-8, the value of the measured (observed) test statistic of the F-test for
(Multiple Choice)
4.8/5  (35)
(35)
SCENARIO 13-12
The manager of the purchasing department of a large saving and loan organization would like to
develop a model to predict the amount of time (measured in hours) it takes to record a loan
application. Data are collected from a sample of 30 days, and the number of applications recorded and
completion time in hours is recorded. Below is the regression output: Regression Statistics Multiple R 0.9447 R Square 0.8924 Adjusted R 0.8886 Square Standard 0.3342 Error Observations 30
 Coefficients Standard Error t Stat P-value Lower 95\% Upper 95\% Intercept 0.4024 0.1236 3.2559 0.0030 0.1492 0.6555 Applications 0.0126 0.0008 15.2388 0.0000 0.0109 0.0143
-Referring to Scenario 13-12, predict the amount of time it would take to process 150 invoices.
Coefficients Standard Error t Stat P-value Lower 95\% Upper 95\% Intercept 0.4024 0.1236 3.2559 0.0030 0.1492 0.6555 Applications 0.0126 0.0008 15.2388 0.0000 0.0109 0.0143
-Referring to Scenario 13-12, predict the amount of time it would take to process 150 invoices.
(Short Answer)
4.9/5  (34)
(34)
SCENARIO 13-3
The director of cooperative education at a state college wants to examine the effect of cooperative
education job experience on marketability in the work place. She takes a random sample of 4
students. For these 4, she finds out how many times each had a cooperative education job and how
many job offers they received upon graduation. These data are presented in the table below. Student CoopJobs JobOffer 1 1 4 2 2 6 3 1 3 4 0 1
-Referring to Scenario 13-3, the standard error of estimate is __________.
(Short Answer)
4.8/5  (36)
(36)
SCENARIO 13-13
In this era of tough economic conditions, voters increasingly ask the question: "Is the educational
achievement level of students dependent on the amount of money the state in which they reside
spends on education?" The partial computer output below is the result of using spending per student
($) as the independent variable and composite score which is the sum of the math, science and
reading scores as the dependent variable on 35 states that participated in a study. The table includes
only partial results. Regression Statistics Multiple R 0.3122 R Square 0.0975 Adjusted R 0.0701 Square Standard 26.9122 Error Observations 35

 -Referring to Scenario 13-13, if the state decides to spend 1,000 dollar more per student, the
estimated change in mean composite score is _________.
-Referring to Scenario 13-13, if the state decides to spend 1,000 dollar more per student, the
estimated change in mean composite score is _________.
(Short Answer)
4.9/5  (36)
(36)
SCENARIO 13-2
A candy bar manufacturer is interested in trying to estimate how sales are influenced by the price of
their product. To do this, the company randomly chooses 6 small cities and offers the candy bar at
different prices. Using candy bar sales as the dependent variable, the company will conduct a simple
linear regression on the data below:
-Referring to Scenario 13-2, what is the coefficient of correlation for these data?
(Multiple Choice)
4.7/5  (37)
(37)
Showing 61 - 80 of 243
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)